函数范围

数学中的功能可以被视为自动售货机。给定投入的钱，他们会给一些罐头或饼干作为回报。类似地，函数接受一些输入数字并提供一些输出。可以说，在现实生活中，一切都可以借助功能来制定和解决。从建筑设计，建筑到巨型摩天大楼，现实生活中几乎所有事物的数学模型都需要函数，因此，不可避免的是函数在我们的生活中具有举足轻重的意义。域和范围是可以描述函数的一个方面。

例如：假设它写在机器顶部，只能使用Rs.20和Rs.50纸币购买东西。如果有人使用10卢比的钞票怎么办?机器将不输出任何信息。因此，域表示函数可以具有的输入类型。在这种情况下，Rs.20和Rs.50便笺是“自动售货机”的域。同样，放多少钱也没关系，他/她永远也不会从机器上得到三明治。因此，范围的概念在这里发挥了作用，范围是机器可以提供的可能的输出。

函数的范围和域

函数：

域是可以进入函数的所有值，为其提供有效的输出。它是函数所有可能输入的函数。

例如：在下图中，f(x)= x ² 。所有输入的集合称为“域”，所有输出的集合被视为范围。

如何找到函数的域?

函数的域应包含所有实数，但分母变为零且平方根下的项变为负的点除外。要查找域，请尝试查找未定义函数的点或输入值。

问题1：找出$\frac{1}{1-x}$

回答：

This function can give undefined output when x = 1. So, then domain is R – {1}. 

问题2：找到以下函数的域：

$\frac{x^2}{(x-3)(x-5)}$

答：

It is Important to not make the function either Infinity or Undefined, therefore, we need to see what Domain values can make the Function Undefined or Infinity.

Taking a look at the denominator, It is clear that the values 3 and 5 are making the denominator 0, hence, making the function Infinite which is not desirable. 

Therefore, the values x=3 and x=5 can’t be placed here.

The Domain will be R – {3,5}.

问题3：找到函数Y =(2x ² -1)和Z =(1-3x)相等的域值。

答：

Equating the two Functions:

2 x²– 1 = 1 – 3 x

2x² + 3x – 2 = 0

2x² + 4x – x – 2 = 0

2x (x + 2) – 1 (x+2)= 0

(2x – 1) (x + 2) = 0

x = 1/2, -2.

Therefore, the Domain values are {1/2, -2}.

函数范围

函数的范围是一组所有可能的输出。

示例：让我们考虑一个函数ƒ：A⇢A，其中A = {1,2,3,4}。

集合域的元素称为原图像，映射到原图像的集合共同域的元素称为图像。函数的范围是域中元素的所有图像的集合。在此示例中，函数的范围为{2,3}。

如何找到一个函数的范围?

范围是函数输出值的范围。如果我们能够计算函数的最大值和最小值，则可以了解函数的范围。

问题1：找到范围。 f(x)= $\sqrt{x - 1}$

回答：

Now, since the function is a square root, it can never give negative values as output. So, the minimum value can only be 0 at x = 1. Maximum value can go up to infinity as we keep on increasing x. 

So, the range of the function is [0,∞). 

问题2：由f(x)=定义的函数ƒ的域$\frac{1}{\sqrt{x - |x|}}$是?

回答：

Given, f(x) = $\frac{1}{\sqrt{x - |x|}}$. 

One has to ensure two things while selecting the set of domain, 

• Denominator never goes to zero.
• Term is inside the square root doesn’t become negative.

Let’s expand what’s written inside term within square root. 

$\sqrt{x - |x|}= \begin{cases} x - x = 0,& \text{if } x\geq 0\\ 2x, & \text{otherwise} \end{cases}$ 

In this case, we cannot put either of the values, x ≥ 0 or x < 0. 

Hence, f is not defined for any x ∈ R. So, the domain is an empty set. 

二次函数的域和范围

二次函数是形式为f(x)= ax ² + bx + c的函数，其中a，b和c为常数且a≠0。二次函数的图为抛物线形式。它基本上是向上或向下打开的弯曲形状。

让我们看一下如何绘制二次函数图，

所以，在我们的二次函数

• 如果a> 0，则抛物线向上打开。
• 如果<0，则抛物线向下打开。

现在，顶点是曲线的最高点或最低点，具体取决于二次函数的图。查找一般二次表达式的图的顶点。

在标准二次形式中，顶点由下式给出$(\frac{-b}{2a}, f(\frac{-b}{2a}))$必须先找到顶点的x值，然后才将其插入函数以获取y值。

Note: Each curve is symmetrical around its vertical axis. 

让我们看一些例子，

问题：绘制f(x)= 2x ² + 4x + 2的图。

回答：

Comparing this equation with the general quadratic function equation. a = 2, b = -4 and c = 2. 

Since a > 0, this parabola will open upwards. 

• Vertex x-value = $\frac{-b}{2a} = \frac{-4}{4} = -1$
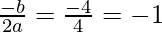
• Vertex y-value = 2(-1)² + 4(-1) + 2 = 0

So, the vertex is at (-1,0). Since the parabola opens upwards, this must be the minimum value of the function. 

The point where graph cuts y-axis is (0,2). 

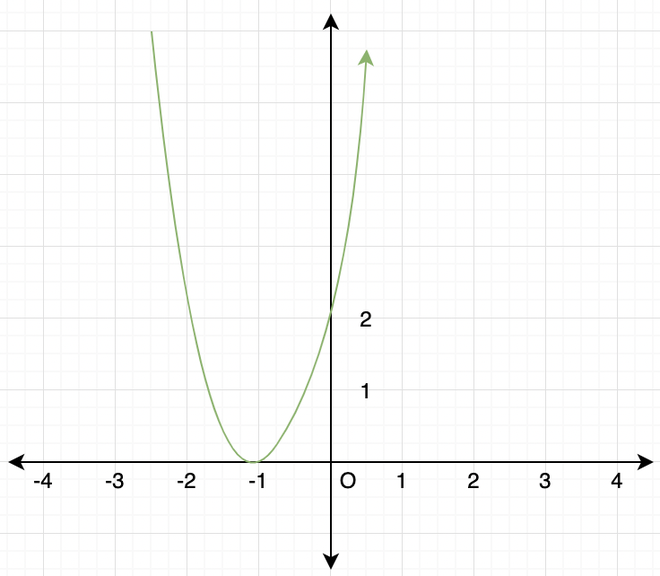
通过绘制图形可以轻松找出二次函数的范围和域。不一定总是需要绘制完整的图形，因为对于范围，仅应该知道抛物线的方向(向上或向下)和抛物线在顶点处的值。顶点的值始终是最小值/最大值，这取决于抛物线的方向。这些函数的域始终是整数，因为在各处都定义了整数，即：没有输入值，可能会导致它们给出未定义的输出。

让我们看一下有关抛物线的范围和范围的另一个示例。

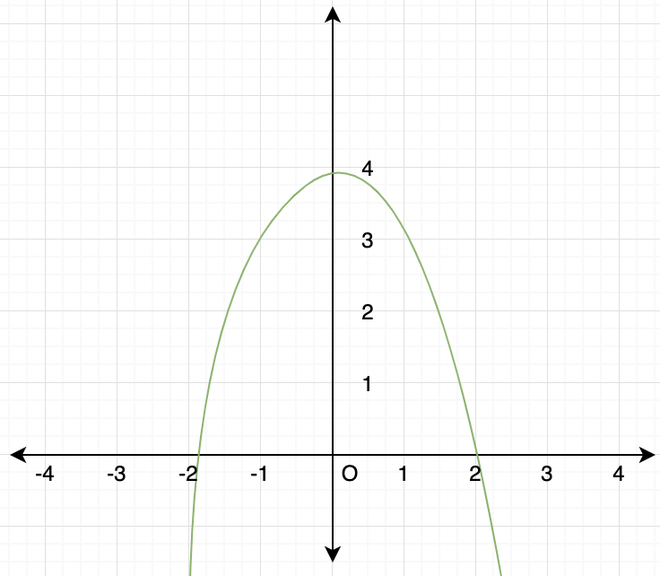
问题：绘制图形并找到给定函数f(x)= -x ² + 4的域和范围。

回答：

Since, a = -1. Parabola will open downwards i.e; there will be no minimum value, it will extend to infinity. But there will be a maximum value which will occur at vertex. 

To find the position of the vertex, previous formula can be used. The vertex is at position (0,4). 

The value at vertex (0,4) = (0)² + 4 = 4. 

So, the maximum value is 4 and minimum value is negative of infinity. 

Range of the function – (-∞, 4] and the domain is R.