三角形的同余

勾股定理是几何的一种支柱。在数学中，勾股定理或毕达哥拉斯定理是直角三角形三个边之间的欧几里得几何学的基本关系。让我们看看勾股定理的实际概念和例子。

毕达哥拉斯定理声明

如果我们有一个三角形，并且三角形必须是直角三角形，即三角形中的三个角度之一必须为90度。现在，根据毕达哥拉斯定理，如果我们知道直角三角形的两个边，我们可以找出第三边。

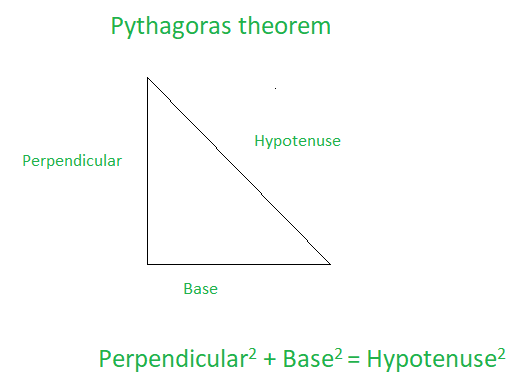
直角三角形的最长边是与直角相反的边，即斜边。同样，我们可以在上图中看到，与90度角相邻的两侧的平方等于斜边的平方。

勾股定理例子

让我们看一下勾股定理的一些基本例子。

示例1：确定边16个单位的等边三角形的高度?

解决方案：

For the given problem we can split the triangle into two equal parts from which we can get the hypotenuse length is 16 units. And the other leg is 8 units i.e. half of 16 units. So, let the height be x.

According to question,

x² + 8² = 16²

=> x² = 192

=> x = √192

Taking positive value of x

=> x = 13.86 units

Hence, the required height is 13.86 units.

示例2：一个人向西走60m，然后向北走60m。找到他与起点的距离?

解决方案：

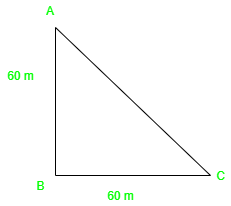
According to the given question, we can draw the diagram as shown above and we have to find the distance AC.

Now, According to the Pythagoras theorem, we can get the equation as

                                 AB² + BC ² = AC²

                                   =>  AC² = 60²  + 60 ²

                          =>  AC²  = 7200

                          =>  AC  =  √7200

                          =>  AC =  84.85 m ( Since, it is a distance unit. Therefore taking positive value of square root)

Hence, the required distance is 84.85 m.

三角形同余假设/条件

一种。 SSS(侧面，侧面，侧面)

在SSS的假设中，如果一个三角形的所有三个边都等于另一个三角形的相应三个边，则它满足SSS的条件。

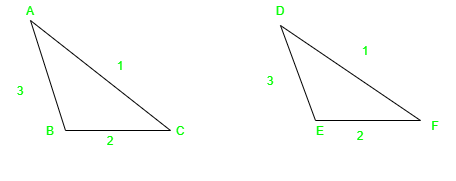
在上图中，我们可以看到AB = DE，AC = DF，BC = EF。因此，ΔABC≅ΔDEF

b。 SAS(侧面，角度，侧面)

在SAS的假设中，如果两个三角形之间以及两个三角形之间的夹角相等，则在两个三角形之间进行比较。然后满足SAS的条件。

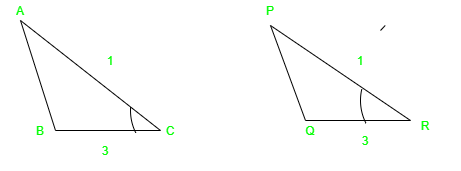
在上图中，我们可以看到，AC = PR，BC = QR，QRC =∠R。因此，ΔACB≅ΔPRQ。

C。 ASA(角度，侧面，角度)

在这里，如果我们有两个三角形，则其中一个三角形的两个角度之间包含的任意两个角度和侧面等于相应三角形的角度和侧面。然后，两个三角形将满足ASA一致性要求。

在上面给出的图中，我们可以看到BC = YZ，∠B=，Y，∠C=∠Z。因此，ΔACB≅ΔXZY。

d。 AAS(角度，角度，侧面)

如果三角形和不包含边的任意两个角度等于相应三角形的两个角度和边。这样就可以满足AAS一致性要求。

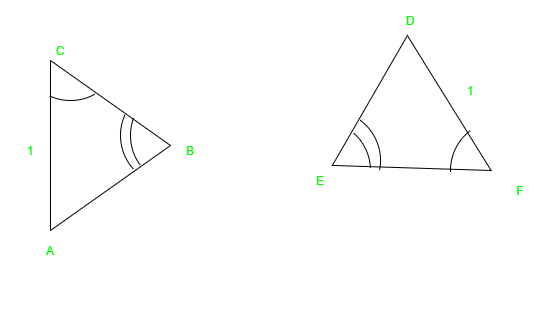
从给定的数字，我们可以说，

AC = DF，

∠B=∠E，

∠C=∠F

因此，ΔACB≅ΔDFE

e。 RHS(直角-斜边-侧面)

RHS标准仅对直角三角形感到满意。因此，在RHS一致性中，如果只有斜边和直角三角形的一侧的两个边等于另一个斜角和另一个直角三角形的一侧的，则它们相等。那么我们可以说RHS感到满意。

从上面给出的直角三角形示例中，我们得到AC = DF和BC = EF。因此，ΔACB≅ΔDFE。

为什么SSA不是一致的假设/标准?

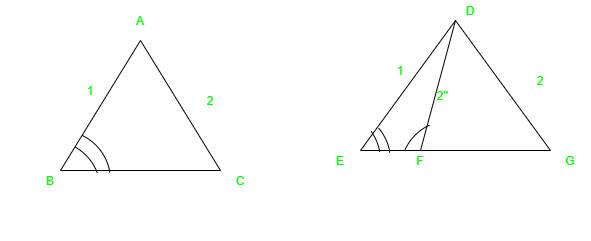
证明：

Let’s first see in Δ ABC and Δ DEG

∠B = ∠E, AB= DE and AC = DG    

So, from this above condition, we cannot justify that Δ ABC ≅  ΔDEG. To understand this, let’s construct a line DF in Δ DEG as shown above, and DF is congruent to AC and DG. We have got a new triangle i.e. Δ DEF.

Now, we can see that AC is congruent to DF, ∠B = ∠E, and AC = DE. But,  Δ ABC is not congruent to ΔDEF. Because of which we can make a conclusion that SSA is not only sufficient to tell that triangles are congruent. Therefore, it is important to know the ∠A and ∠D means the angle between the two sides.

There is only one exceptional case which we have seen in the RHS postulate, on which SSA or ASS postulate will get satisfied. Henceforth, in a general or common scenario, SSA or ASS postulate will not be satisfying.