弯曲表面的光折射

当介质发生变化时，速度和波长会发生变化，但频率保持不变。光速在特定介质中保持不变。光似乎在透明介质中沿着直线路径传播。但是当它从一种透明介质传播到另一种时会发生什么，答案就是折射。

考虑部分浸入水中的铅笔的明显位移。从浸没在水中的铅笔部分照射到你的光似乎是从与水面上方不同的方向发出的。因此，铅笔似乎在界面处移动。由此，我们可以说光在所有介质中的传播方向并不相同，因此当从一种介质倾斜地传播到另一种介质时，光在第二种介质中的传播方向会发生变化。这种现象称为光的折射。

When a monochromatic ray of light travels from one transparent medium into another transparent medium, its direction changes (except for normal incidence). 

折射定律

• 折射第一定律：入射光线和折射光线在入射点的法线的相对两侧，并且三者都在同一个平面上。
• 折射第二定律：对于给定的一对介质，入射角的正弦与折射角的正弦之比是恒定的。 （该常数称为第二介质相对于第一介质的折射率）。它写成，

¹μ₂ = sin i / sin r = Constant

This is called Snell`s law.

When a ray of light travels from medium 1 into medium 2, then Snell`s law is written as,

¹μ₂ = ¹n₂ sin i / sin r

where,

i is the angle of incidence in 1st medium, 

r is the angle of refraction in 2nd medium, and      

¹n₂ is the relative refractive index.

曲面的折射

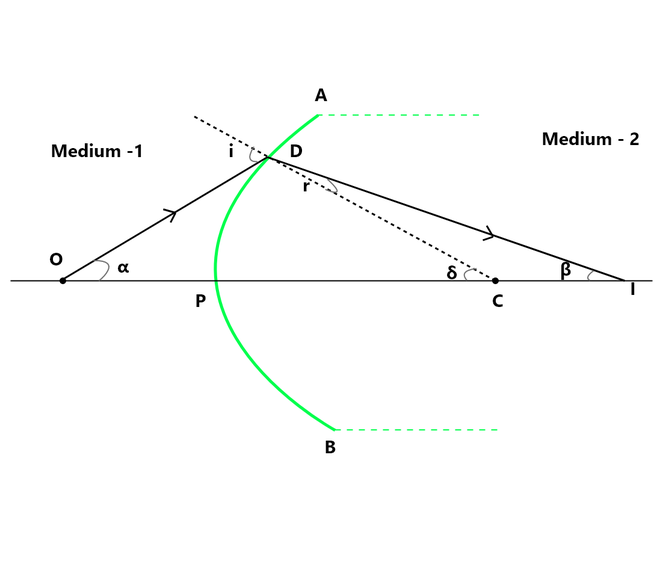
曲面折射

考虑将介质 1 和介质 2 分开的球面的 APB。令 μ ₁表示介质 1 的折射率，μ ₂表示介质 2 的折射率 (μ ₂ > μ ₁ ) 其中 C 是曲率中心，R 是曲率半径，i 是入射角.

现在，考虑介质中的对象“O”- 1. 入射光线 OP 与 PI 一起通过介质 2 不受影响。考虑第二条光线 OD，它入射到表面上并在点 D 处形成入射角“i”。折射后，它向法线 CD 弯曲并在点 I 处与轴相交。因此，点 I 是点对象O的真实表示。因此，OP表示对象到表面的距离，PI表示图像到表面的距离，PC表示曲率半径（因为C是曲率中心）。

Looking at the above figure and according to the new Cartesian sign convention, we can say that, object distance OP is -ve and image distance (PI) and radius of curvature (PC) are +ve.

Therefore, 

• Object distance = OP = -u
• Image distance = PI = +v
• Radius of curvature  = PC = +R  

Let α, β, δ be the angles made by OD, ID and CD with the principle axis. From Snell`s Law, we can write,

μ₂ / μ₁ = sin i / sin r                         …….(1)

Now if we consider the point D to be very close to point P, then all the angles, i, r, α, β, δ will also be small, So in terms of radians,

sin i = i and sin r = r

μ₁ i = μ₂ r                                   …….(2)

From figure,

∠i = ∠α + ∠δ                       (By Exterior angle theorem)

Similarly,

∠δ = ∠r + ∠β  

or,

∠r = ∠δ – ∠β 

Substituting both the values in equation (2) we get,

μ₁ (α + β) = μ₂ (δ – β)

μ₁ α + μ₂ β = ( μ₂ – μ₁  ) δ                               …….(3)

As angles are small, we can express them in radians as,

$\alpha = \frac{arc\;PD}{PO}; \;\;\; \beta = \frac{arc\;PD}{PI}; \;\;\; \delta = \frac{arc\;PD}{PC}$

$\alpha = \frac{arc\;PD}{-u}; \;\;\; \beta = \frac{arc\;PD}{+v}; \;\;\; \delta = \frac{arc\;PD}{+R}$

Substituting these values in equation (3) as,

$\mu_1\;(\frac{arc\;PD}{-u}) \;+\;\mu_2(\frac{arc\;PD}{+v}) \; = \; (\mu_2 - \mu_1)(\frac{arc\;PD}{+R})$

μ₂  / v – μ₁ / u =( μ₂ – μ₁ )/ R

The above expression gives the relation between u, v, μ₁, μ₂ and R for refraction at the curved surface. 

该表达式是针对真实图像获得的，但同样适用于虚拟图像和任何一对折射介质。在使用上述表达式时，量 v、u、R 将与其适当的符号一起使用（根据新的符号笛卡尔系统）。

折射在曲面上的应用

• 透镜用于折射以生成图片，类似于放大。
• 门上的窥视孔，实验室使用的放大镜。
• 折射的另一种用途是 VIBGYOR，它是白光在穿过玻璃棱镜时被分成颜色光谱的方式。
• 为了矫正人眼的屈光缺陷，使用了屈光凹凸眼镜。
• 海市蜃楼或星星的闪烁是大气折射的一个突出例子。

示例问题

问题1：一束光线以70°的入射角入射到水面上。当光线进入水中时，光线会从 25° 向法线方向偏离。计算水的折射率。

解决方案：

Given that,

i = 70°

δ = 25° 

Since, it is known that,

δ = i – r

r = i – δ

= 45°

Also, 

μ = sin i / sin r

= sin 70° / sin 45°

= 0.9397 / 0.7071

= 1.33

The refractive index of water is 1.33.

问题 2：曲率半径为 5 厘米的球面将水和玻璃隔开。一个物体放置在距离表面 50 厘米的水中的主轴上。如果表面是，则找到图像的位置，

• 凹和
• 凸的。

（水的折射率为 4/3，玻璃的折射率为 1.5）。

解决方案：

Given that,

u = object distance = -50cm

μ₁ = refractive index of water = 4/3

μ₂ = refractive index of glass = 1.5

Due to refraction at curved surface,

μ₂ / v – μ₁ / u =( μ₂ – μ₁ )/ R

μ₂ / v =( μ₂ – μ₁ )/ R + μ₁ / u

• For concave surface R = -5cm

1.5 / v = [1.5 – (4 / 3) / (-5)] + [(4 / 3) / -50]

v = -25 cm

A virtual image is formed at 25 cm from the pole and on the same side of object.

• For convex surface R = +5cm

1.5 / v = [1.5 – (4 / 3) / (+5)] + [(4 / 3) / -50]

v = 225 cm

A real image is formed at 225 cm from the pole and on the other side of surface.

问题 3：凸面将折射率为 1.3 和 1.5 的两种介质分开。如果曲率半径为 20 厘米，物体距离折射面 260 厘米，则计算图像到折射面的距离。

解决方案：

Given that,

μ₁ = 1.3

μ₂ = 1.5

R = +20cm

u = -260cm (object distance)

Therefore, 

μ₂ / v – μ₁ / u =( μ₂ – μ₁ )/ R

1.5 / v – 1.3 / (-260) = (1.5 – 1.3) / 20

1.5 / v = 0.2 / 20 – 1.3 / 260

This implies,

v = 300 cm 

The image distance from surface is 300 cm.

问题4：空气中的点状物体位于距其极点15cm的凸玻璃表面的主轴上，玻璃的折射率为1.5 ，球面的曲率半径为30cm。找到图像的位置并说明其性质。

解决方案：

Given that,

u = -15cm

μ₁ = 1

μ₂ =1.5    

R = 30cm

Therefore,

μ₂ / v – μ₁ / u =( μ₂ – μ₁ )/ R

or

μ₂ / v =( μ₂ – μ₁ )/ R + μ₁ / u

So,

1.5 / v = ( 1.5 – 1 ) / 30 + (1 / -15)

1.5 / v = -(1 / 20)

This implies,

v = -20 × 1.5 

= -30 cm

The image is located 30 cm from the pole, on the same side as the object. And the nature of image is Virtual.

问题 5：一个点物体被放置在距离一个半径为 2 厘米、折射率为 1.6 的玻璃球中心 8 厘米处。画出射线图并从截然相反的位置观察时找到图像。

解决方案：

Given that,

Object distance = 8 cm

Radius = 2 cm

Refractive index = 1.6

Ray diagram:

A paraxial ray OD suffers two refractions, at the two sides of the glass sphere. For refraction at the side 1, the refracted ray is DE. Had the medium (glass) been continously beyond P₁ ,the ray DE would have formed a real image let`s say at I₁ . Thus, in this case

u = P₁O = -6 cm

mage distance v` = P₁I₁ 

R = P₁C = 2cm

μ₁ = 1

μ₂ = 1.6

We have,

μ₂ / v – μ₁ / u =( μ₂ – μ₁ )/ R

μ₂ / v =( μ₂ – μ₁ )/ R + μ₁ / u

1.6 / v’ = (1.6 – 1) / 2 + 1 / (-6)

            = 0.6 / 2 – 1 / 6

            = 0.8 / 6

v’ = 1.6 × 6 / 0.8

= 12 cm  

For the refraction at the second side, the incidence ray DE is in the glass, the refracted ray is in the air and I₁ acts as the virtual object with object distance

P₂I₁ = P₁I₁ – P₁P₂ = 12cm – 4cm = 8cm

Thus, in this case, 

u = P₂I₁ = 8

image distance = v = P₂I

R = P₂C = -2cm

μ₁ = 1.6

μ₂ = 1

μ₂ / v = (μ₂ – μ₁) / R + μ₂ / u

1 / v = (1 – 1.6) / (-2) + (1.6 / 8)

= 0.3 + 0.2 = 0.5

v = 1 / 0.5

= 2 cm

The final image (real) is formed in air on the side of the observer at a distance of 4cm from the centre of the sphere.