给定两个字符串,长度为N str1和明显不同的字符长度M的STR2,任务是统计的方式发出str1和str2的交替的所有字符数。
注意: | N – M | ≤1
例子:
Input: str1 =“ae”, str2 = “bd”
Output: 8
Explanations:
Possible strings after rearrangements are : {“abed”, “ebad”, “adeb”, “edab”, “bade”, “beda”, “dabe”, “deba”}. Therefore, the required output is 8.
Input: str1= “aegh”, str2=”rsw”
Output: 144
方法:可以根据以下观察结果解决问题:
如果N!= M:仅考虑N> M的情况,因为类似地,它将适用于N

交替放置str1 []和str2 []的可能方法
Total number of ways to rearrange all the characters of str1 = N!
Total number of ways to rearrange all the characters of str2 = M!.
Therefore, the total number of ways to place all the characters of str1 and str2 alternatively are = N! * M!
如果N == M:
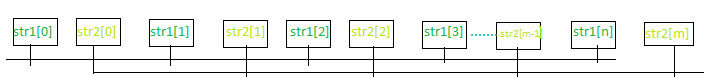
首先STR1 [],然后的字符放置STR2的字符[]

首先STR2 [],然后的字符放置STR1的字符[]
Total number of ways to rearrange all the characters of str1 = N!
Total number of ways to rearrange all the characters of str2 = M!
Now,
There are two cases possible here:
- First place the character of str1 and then place the character of str2.
- First place the character of str2 and then place the character of str1.
Therefore, the total number of ways = (2 * N! * M!).
下面是上述方法的实现:
C++
// C++ Program to implement
// the above approach
#include
using namespace std;
// Function to get the
// factorial of N
int fact(int n)
{
int res = 1;
for (int i = 1; i <= n; i++) {
res = res * i;
}
return res;
}
// Function to get the total
// number of distinct ways
int distinctWays(string str1, string str2)
{
// Length of str1
int n = str1.length();
// Length of str2
int m = str2.length();
// If both strings have equal length
if (n == m) {
return 2 * fact(n) * fact(m);
}
// If both strings do not have
// equal length
return fact(n) * fact(m);
}
// Driver code
int main()
{
string str1 = "aegh";
string str2 = "rsw";
cout << distinctWays(str1, str2);
} Java
// Java program to implement
// the above approach
import java.io.*;
class GFG{
// Function to get the
// factorial of N
static int fact(int n)
{
int res = 1;
for(int i = 1; i <= n; i++)
{
res = res * i;
}
return res;
}
// Function to get the total
// number of distinct ways
static int distinctWays(String str1,
String str2)
{
// Length of str1
int n = str1.length();
// Length of str2
int m = str2.length();
// If both strings have equal length
if (n == m)
{
return 2 * fact(n) * fact(m);
}
// If both strings do not have
// equal length
return fact(n) * fact(m);
}
// Driver code
public static void main (String[] args)
{
String str1 = "aegh";
String str2 = "rsw";
System.out.print(distinctWays(str1, str2));
}
}
// This code is contributed by code_huntPython3
# Python3 program to implement
# the above approach
# Function to get the
# factorial of N
def fact(n):
res = 1
for i in range(1, n + 1):
res = res * i
return res
# Function to get the total
# number of distinct ways
def distinctWays(str1, str2):
# Length of str1
n = len(str1)
# Length of str2
m = len(str2)
# If both strings have equal length
if (n == m):
return 2 * fact(n) * fact(m)
# If both strings do not have
# equal length
return fact(n) * fact(m)
# Driver code
str1 = "aegh"
str2 = "rsw"
print(distinctWays(str1, str2))
# This code is contributed by code_huntC#
// C# program to implement
// the above approach
using System;
class GFG{
// Function to get the
// factorial of N
static int fact(int n)
{
int res = 1;
for(int i = 1; i <= n; i++)
{
res = res * i;
}
return res;
}
// Function to get the total
// number of distinct ways
static int distinctWays(string str1,
string str2)
{
// Length of str1
int n = str1.Length;
// Length of str2
int m = str2.Length;
// If both strings have equal length
if (n == m)
{
return 2 * fact(n) * fact(m);
}
// If both strings do not have
// equal length
return fact(n) * fact(m);
}
// Driver code
public static void Main ()
{
string str1 = "aegh";
string str2 = "rsw";
Console.Write(distinctWays(str1, str2));
}
}
// This code is contributed by code_huntJavascript
144时间复杂度: O(N + M)
辅助空间: O(1)