它与最小生成树不同,因为两个顶点之间的最短距离可能不包括图形的所有顶点。
Dijkstra算法的工作原理
Dijkstra的算法的工作原理是,顶点A和D之间的最短路径A -> D B -> D的任何子路径B -> D也是顶点B和D之间的最短路径。
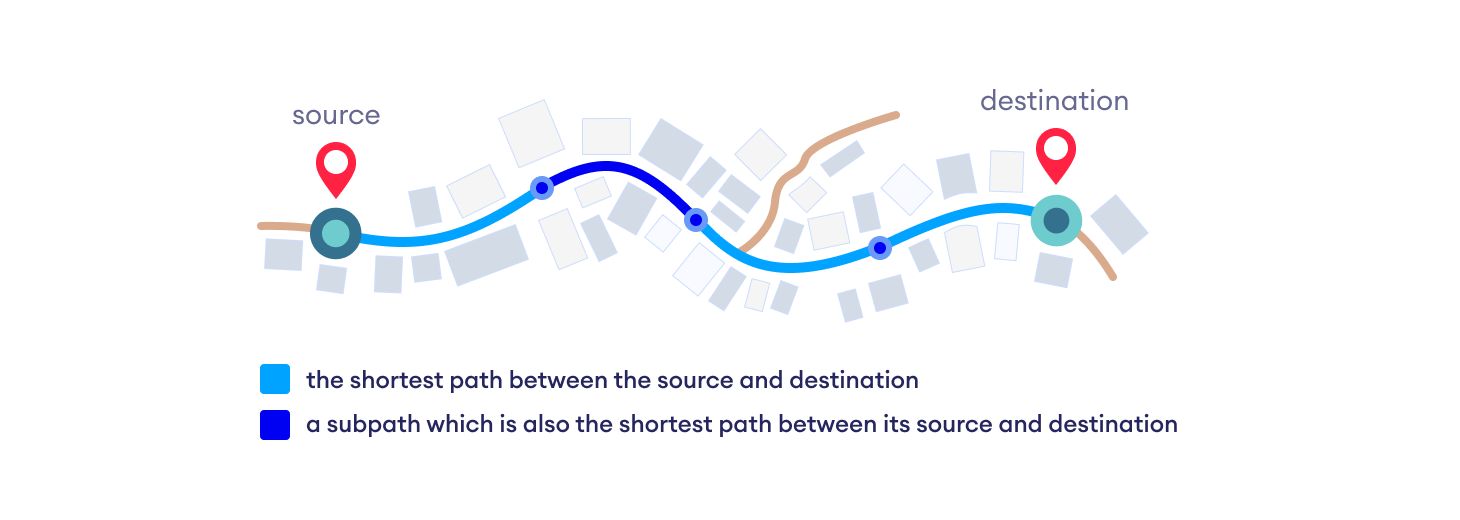
Djikstra在相反的方向上使用了此属性,即我们高估了每个顶点到起始顶点的距离。然后,我们访问每个节点及其邻居,以找到到这些邻居的最短子路径。
该算法使用贪婪方法,因为我们找到了下一个最佳解决方案,希望最终结果是整个问题的最佳解决方案。
Dijkstra算法的示例
从一个示例开始,然后考虑该算法会更容易。
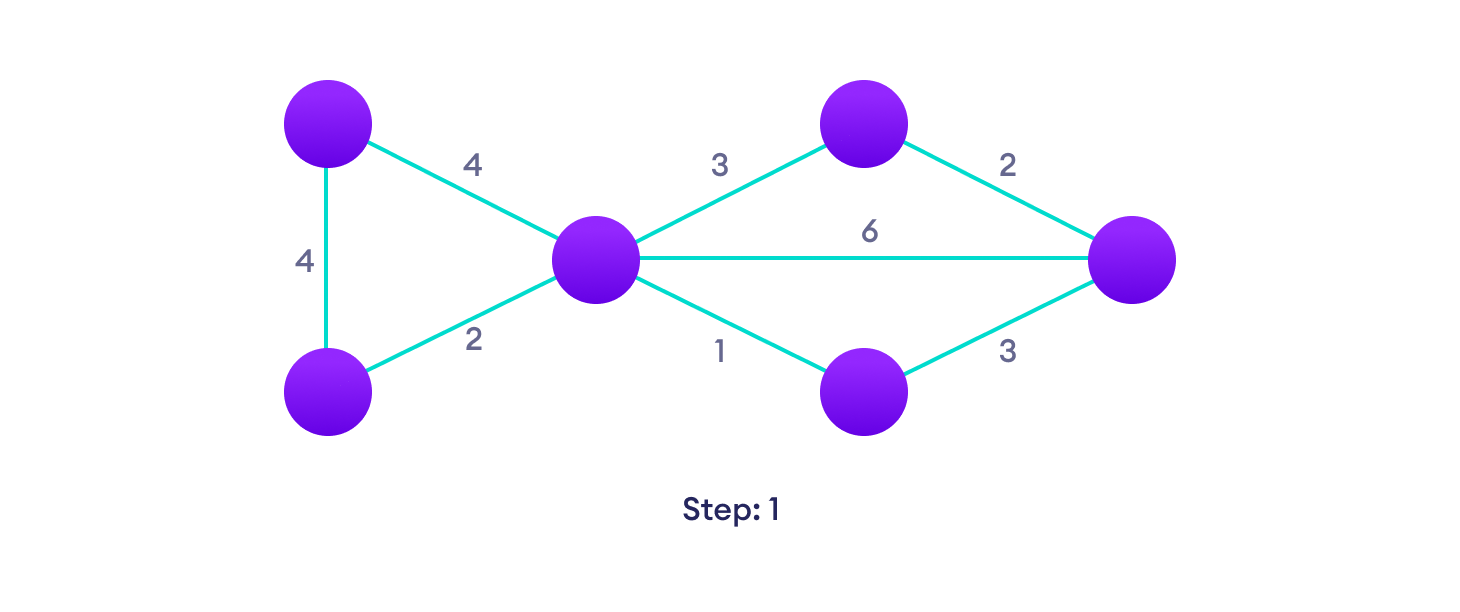
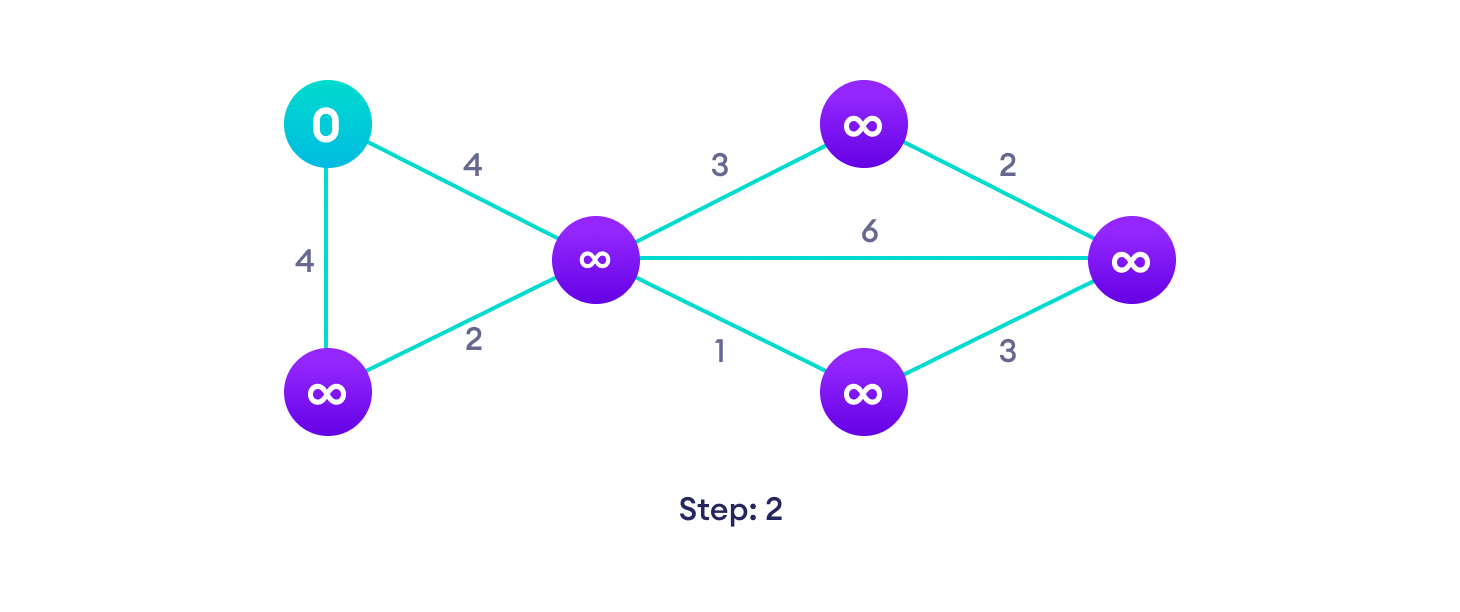

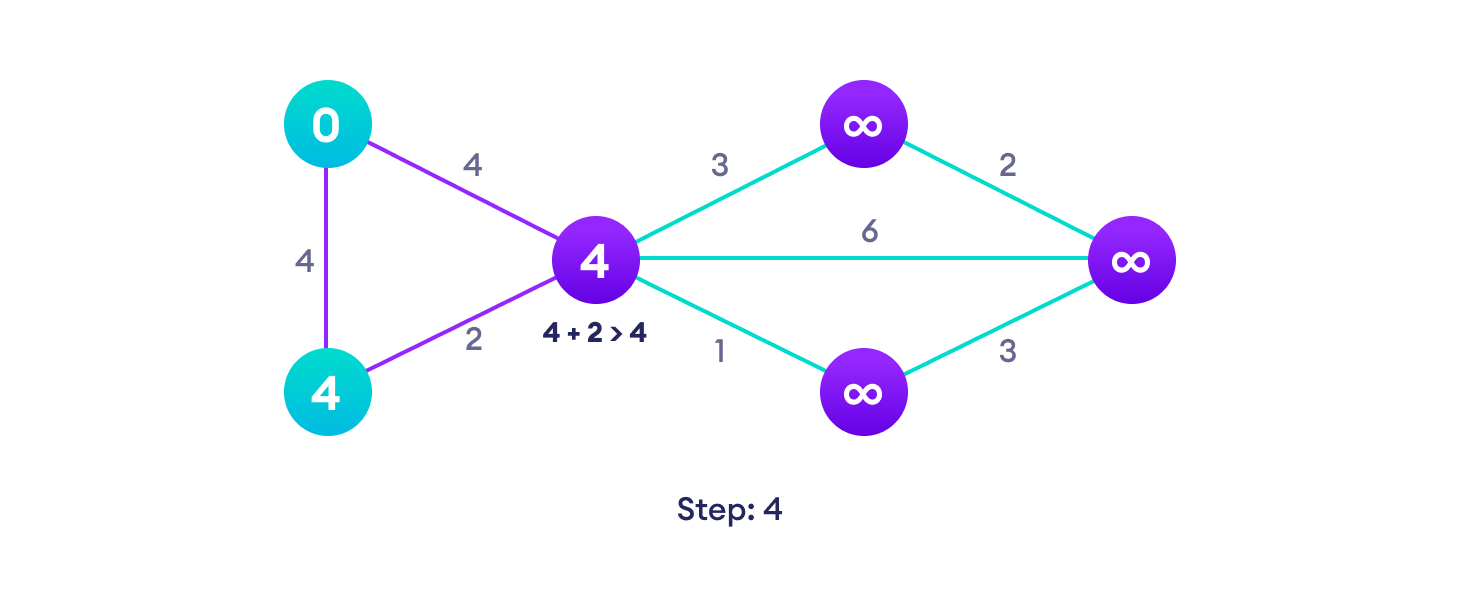
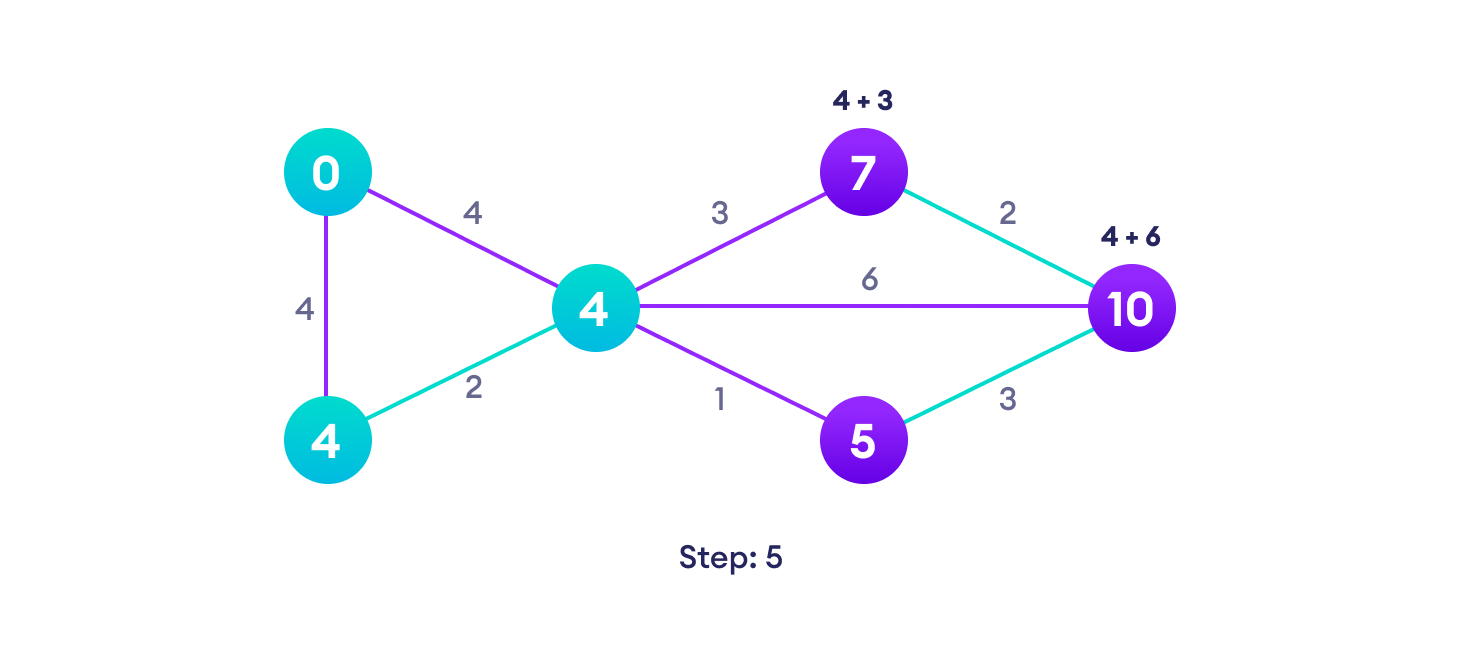


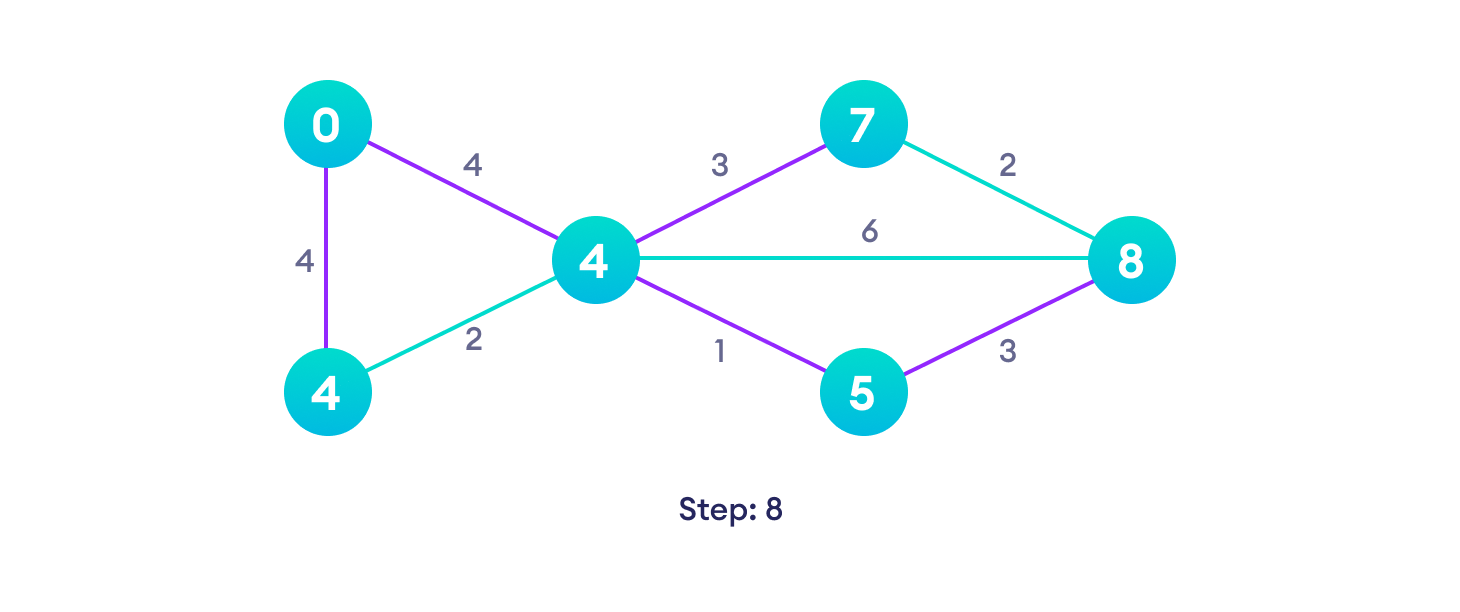
Djikstra的算法伪代码
我们需要保持每个顶点的路径距离。我们可以将其存储在大小为v的数组中,其中v是顶点数。
我们还希望能够获得最短路径,而不仅知道最短路径的长度。为此,我们将每个顶点映射到最后更新其路径长度的顶点。
算法结束后,我们可以从目标顶点回溯到源顶点以找到路径。
最小优先级队列可用于以最小路径距离有效接收顶点。
function dijkstra(G, S)
for each vertex V in G
distance[V] Dijkstra算法的代码
下面给出了Dijkstra算法在C++中的实现。可以提高代码的复杂性,但抽象方法可以方便地将代码与算法关联起来。
Python
爪哇
C
C++
# Dijkstra's Algorithm in Python
import sys
# Providing the graph
vertices = [[0, 0, 1, 1, 0, 0, 0],
[0, 0, 1, 0, 0, 1, 0],
[1, 1, 0, 1, 1, 0, 0],
[1, 0, 1, 0, 0, 0, 1],
[0, 0, 1, 0, 0, 1, 0],
[0, 1, 0, 0, 1, 0, 1],
[0, 0, 0, 1, 0, 1, 0]]
edges = [[0, 0, 1, 2, 0, 0, 0],
[0, 0, 2, 0, 0, 3, 0],
[1, 2, 0, 1, 3, 0, 0],
[2, 0, 1, 0, 0, 0, 1],
[0, 0, 3, 0, 0, 2, 0],
[0, 3, 0, 0, 2, 0, 1],
[0, 0, 0, 1, 0, 1, 0]]
# Find which vertex is to be visited next
def to_be_visited():
global visited_and_distance
v = -10
for index in range(num_of_vertices):
if visited_and_distance[index][0] == 0 \
and (v < 0 or visited_and_distance[index][1] <=
visited_and_distance[v][1]):
v = index
return v
num_of_vertices = len(vertices[0])
visited_and_distance = [[0, 0]]
for i in range(num_of_vertices-1):
visited_and_distance.append([0, sys.maxsize])
for vertex in range(num_of_vertices):
# Find next vertex to be visited
to_visit = to_be_visited()
for neighbor_index in range(num_of_vertices):
# Updating new distances
if vertices[to_visit][neighbor_index] == 1 and \
visited_and_distance[neighbor_index][0] == 0:
new_distance = visited_and_distance[to_visit][1] \
+ edges[to_visit][neighbor_index]
if visited_and_distance[neighbor_index][1] > new_distance:
visited_and_distance[neighbor_index][1] = new_distance
visited_and_distance[to_visit][0] = 1
i = 0
# Printing the distance
for distance in visited_and_distance:
print("Distance of ", chr(ord('a') + i),
" from source vertex: ", distance[1])
i = i + 1// Dijkstra's Algorithm in Java
public class Dijkstra {
public static void dijkstra(int[][] graph, int source) {
int count = graph.length;
boolean[] visitedVertex = new boolean[count];
int[] distance = new int[count];
for (int i = 0; i < count; i++) {
visitedVertex[i] = false;
distance[i] = Integer.MAX_VALUE;
}
// Distance of self loop is zero
distance[source] = 0;
for (int i = 0; i < count; i++) {
// Update the distance between neighbouring vertex and source vertex
int u = findMinDistance(distance, visitedVertex);
visitedVertex[u] = true;
// Update all the neighbouring vertex distances
for (int v = 0; v < count; v++) {
if (!visitedVertex[v] && graph[u][v] != 0 && (distance[u] + graph[u][v] < distance[v])) {
distance[v] = distance[u] + graph[u][v];
}
}
}
for (int i = 0; i < distance.length; i++) {
System.out.println(String.format("Distance from %s to %s is %s", source, i, distance[i]));
}
}
// Finding the minimum distance
private static int findMinDistance(int[] distance, boolean[] visitedVertex) {
int minDistance = Integer.MAX_VALUE;
int minDistanceVertex = -1;
for (int i = 0; i < distance.length; i++) {
if (!visitedVertex[i] && distance[i] < minDistance) {
minDistance = distance[i];
minDistanceVertex = i;
}
}
return minDistanceVertex;
}
public static void main(String[] args) {
int graph[][] = new int[][] { { 0, 0, 1, 2, 0, 0, 0 }, { 0, 0, 2, 0, 0, 3, 0 }, { 1, 2, 0, 1, 3, 0, 0 },
{ 2, 0, 1, 0, 0, 0, 1 }, { 0, 0, 3, 0, 0, 2, 0 }, { 0, 3, 0, 0, 2, 0, 1 }, { 0, 0, 0, 1, 0, 1, 0 } };
Dijkstra T = new Dijkstra();
T.dijkstra(graph, 0);
}
}// Dijkstra's Algorithm in C
#include
#define INFINITY 9999
#define MAX 10
void Dijkstra(int Graph[MAX][MAX], int n, int start);
void Dijkstra(int Graph[MAX][MAX], int n, int start) {
int cost[MAX][MAX], distance[MAX], pred[MAX];
int visited[MAX], count, mindistance, nextnode, i, j;
// Creating cost matrix
for (i = 0; i < n; i++)
for (j = 0; j < n; j++)
if (Graph[i][j] == 0)
cost[i][j] = INFINITY;
else
cost[i][j] = Graph[i][j];
for (i = 0; i < n; i++) {
distance[i] = cost[start][i];
pred[i] = start;
visited[i] = 0;
}
distance[start] = 0;
visited[start] = 1;
count = 1;
while (count < n - 1) {
mindistance = INFINITY;
for (i = 0; i < n; i++)
if (distance[i] < mindistance && !visited[i]) {
mindistance = distance[i];
nextnode = i;
}
visited[nextnode] = 1;
for (i = 0; i < n; i++)
if (!visited[i])
if (mindistance + cost[nextnode][i] < distance[i]) {
distance[i] = mindistance + cost[nextnode][i];
pred[i] = nextnode;
}
count++;
}
// Printing the distance
for (i = 0; i < n; i++)
if (i != start) {
printf("\nDistance from source to %d: %d", i, distance[i]);
}
}
int main() {
int Graph[MAX][MAX], i, j, n, u;
n = 7;
Graph[0][0] = 0;
Graph[0][1] = 0;
Graph[0][2] = 1;
Graph[0][3] = 2;
Graph[0][4] = 0;
Graph[0][5] = 0;
Graph[0][6] = 0;
Graph[1][0] = 0;
Graph[1][1] = 0;
Graph[1][2] = 2;
Graph[1][3] = 0;
Graph[1][4] = 0;
Graph[1][5] = 3;
Graph[1][6] = 0;
Graph[2][0] = 1;
Graph[2][1] = 2;
Graph[2][2] = 0;
Graph[2][3] = 1;
Graph[2][4] = 3;
Graph[2][5] = 0;
Graph[2][6] = 0;
Graph[3][0] = 2;
Graph[3][1] = 0;
Graph[3][2] = 1;
Graph[3][3] = 0;
Graph[3][4] = 0;
Graph[3][5] = 0;
Graph[3][6] = 1;
Graph[4][0] = 0;
Graph[4][1] = 0;
Graph[4][2] = 3;
Graph[4][3] = 0;
Graph[4][4] = 0;
Graph[4][5] = 2;
Graph[4][6] = 0;
Graph[5][0] = 0;
Graph[5][1] = 3;
Graph[5][2] = 0;
Graph[5][3] = 0;
Graph[5][4] = 2;
Graph[5][5] = 0;
Graph[5][6] = 1;
Graph[6][0] = 0;
Graph[6][1] = 0;
Graph[6][2] = 0;
Graph[6][3] = 1;
Graph[6][4] = 0;
Graph[6][5] = 1;
Graph[6][6] = 0;
u = 0;
Dijkstra(Graph, n, u);
return 0;
} // Dijkstra's Algorithm in C++
#include
#include
#define INT_MAX 10000000
using namespace std;
void DijkstrasTest();
int main() {
DijkstrasTest();
return 0;
}
class Node;
class Edge;
void Dijkstras();
vector* AdjacentRemainingNodes(Node* node);
Node* ExtractSmallest(vector& nodes);
int Distance(Node* node1, Node* node2);
bool Contains(vector& nodes, Node* node);
void PrintShortestRouteTo(Node* destination);
vector nodes;
vector edges;
class Node {
public:
Node(char id)
: id(id), previous(NULL), distanceFromStart(INT_MAX) {
nodes.push_back(this);
}
public:
char id;
Node* previous;
int distanceFromStart;
};
class Edge {
public:
Edge(Node* node1, Node* node2, int distance)
: node1(node1), node2(node2), distance(distance) {
edges.push_back(this);
}
bool Connects(Node* node1, Node* node2) {
return (
(node1 == this->node1 &&
node2 == this->node2) ||
(node1 == this->node2 &&
node2 == this->node1));
}
public:
Node* node1;
Node* node2;
int distance;
};
///////////////////
void DijkstrasTest() {
Node* a = new Node('a');
Node* b = new Node('b');
Node* c = new Node('c');
Node* d = new Node('d');
Node* e = new Node('e');
Node* f = new Node('f');
Node* g = new Node('g');
Edge* e1 = new Edge(a, c, 1);
Edge* e2 = new Edge(a, d, 2);
Edge* e3 = new Edge(b, c, 2);
Edge* e4 = new Edge(c, d, 1);
Edge* e5 = new Edge(b, f, 3);
Edge* e6 = new Edge(c, e, 3);
Edge* e7 = new Edge(e, f, 2);
Edge* e8 = new Edge(d, g, 1);
Edge* e9 = new Edge(g, f, 1);
a->distanceFromStart = 0; // set start node
Dijkstras();
PrintShortestRouteTo(f);
}
///////////////////
void Dijkstras() {
while (nodes.size() > 0) {
Node* smallest = ExtractSmallest(nodes);
vector* adjacentNodes =
AdjacentRemainingNodes(smallest);
const int size = adjacentNodes->size();
for (int i = 0; i < size; ++i) {
Node* adjacent = adjacentNodes->at(i);
int distance = Distance(smallest, adjacent) +
smallest->distanceFromStart;
if (distance < adjacent->distanceFromStart) {
adjacent->distanceFromStart = distance;
adjacent->previous = smallest;
}
}
delete adjacentNodes;
}
}
// Find the node with the smallest distance,
// remove it, and return it.
Node* ExtractSmallest(vector& nodes) {
int size = nodes.size();
if (size == 0) return NULL;
int smallestPosition = 0;
Node* smallest = nodes.at(0);
for (int i = 1; i < size; ++i) {
Node* current = nodes.at(i);
if (current->distanceFromStart <
smallest->distanceFromStart) {
smallest = current;
smallestPosition = i;
}
}
nodes.erase(nodes.begin() + smallestPosition);
return smallest;
}
// Return all nodes adjacent to 'node' which are still
// in the 'nodes' collection.
vector* AdjacentRemainingNodes(Node* node) {
vector* adjacentNodes = new vector();
const int size = edges.size();
for (int i = 0; i < size; ++i) {
Edge* edge = edges.at(i);
Node* adjacent = NULL;
if (edge->node1 == node) {
adjacent = edge->node2;
} else if (edge->node2 == node) {
adjacent = edge->node1;
}
if (adjacent && Contains(nodes, adjacent)) {
adjacentNodes->push_back(adjacent);
}
}
return adjacentNodes;
}
// Return distance between two connected nodes
int Distance(Node* node1, Node* node2) {
const int size = edges.size();
for (int i = 0; i < size; ++i) {
Edge* edge = edges.at(i);
if (edge->Connects(node1, node2)) {
return edge->distance;
}
}
return -1; // should never happen
}
// Does the 'nodes' vector contain 'node'
bool Contains(vector& nodes, Node* node) {
const int size = nodes.size();
for (int i = 0; i < size; ++i) {
if (node == nodes.at(i)) {
return true;
}
}
return false;
}
///////////////////
void PrintShortestRouteTo(Node* destination) {
Node* previous = destination;
cout << "Distance from start: "
<< destination->distanceFromStart << endl;
while (previous) {
cout << previous->id << " ";
previous = previous->previous;
}
cout << endl;
}
// these two not needed
vector* AdjacentEdges(vector& Edges, Node* node);
void RemoveEdge(vector& Edges, Edge* edge);
vector* AdjacentEdges(vector& edges, Node* node) {
vector* adjacentEdges = new vector();
const int size = edges.size();
for (int i = 0; i < size; ++i) {
Edge* edge = edges.at(i);
if (edge->node1 == node) {
cout << "adjacent: " << edge->node2->id << endl;
adjacentEdges->push_back(edge);
} else if (edge->node2 == node) {
cout << "adjacent: " << edge->node1->id << endl;
adjacentEdges->push_back(edge);
}
}
return adjacentEdges;
}
void RemoveEdge(vector& edges, Edge* edge) {
vector::iterator it;
for (it = edges.begin(); it < edges.end(); ++it) {
if (*it == edge) {
edges.erase(it);
return;
}
}
} Dijkstra的算法复杂度
时间复杂度: O(E Log V)
其中,E是边数,V是顶点数。
空间复杂度: O(V)
Dijkstra的算法应用
- 寻找最短路径
- 在社交网络中的应用
- 在电话网中
- 在地图上查找位置