Triacontakaihenagonal number

Triacontakaihenagonagonal数字是一类数字。它具有31面的多边形，称为triacontakaihenagon 。第N个三方正七角点数是31个点的数量，所有其他点都被一个公共的共享角包围并形成一个图案。
前三个三苯环己烯醇的编号是：

1, 31, 90, 178 … 
 

检查N是否为triacontakaihenagonol号

给定数N ，任务是找到^第N^个三二十碳六烯对角数。
例子：

Input: N = 2 
Output: 31 
Explanation: 
The second triacontakaihenagonol number is 31. 
Input: N = 3 
Output: 90 
 

方法：在数学中，第N个triacontakaihenagonagonal数由下式给出：

• 侧多边形的第N个项= $\frac{((s-2)n^2 - (s-4)n)}{2}$
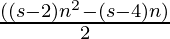
• 因此，第31个面的多边形的第N个项是

$Tn =\frac{((31-2)n^2 - (31-4)n)}{2} =\frac{(29n^2 - 27n)}{2}$

下面是上述方法的实现：

C++

// C++ implementation for
// above approach
 
#include 
using namespace std;
 
// Function to find the Nth
// triacontakaihenagonal Number
int triacontakaihenagonalNum(int n)
{
    return (29 * n * n - 27 * n) / 2;
}
 
// Driver Code
int main()
{
    int n = 3;
    cout << triacontakaihenagonalNum(n);
 
    return 0;
}

Java

// Java implementation for the
// above approach
import java.util.*;
 
class GFG{
 
// Function to find the Nth
// triacontakaihenagonal Number
static int triacontakaihenagonalNum(int n)
{
    return (29 * n * n - 27 * n) / 2;
}
 
// Driver Code
public static void main (String[] args)
{
     
    // Given number
    int n = 3;
 
    // Function call
    System.out.print(triacontakaihenagonalNum(n));
}
}
 
// This code is contributed by Ritik Bansal

Python3

# Python3 implementation for
# above approach
 
# Function to find the Nth
# triacontakaihenagonal Number
def triacontakaihenagonalNum(n):
 
    return (29 * n * n - 27 * n) // 2;
 
# Driver Code
n = 3;
print(triacontakaihenagonalNum(n));
 
# This code is contributed by Code_Mech

C#

// C# implementation for the
// above approach
using System;
  
class GFG{
  
// Function to find the Nth
// triacontakaihenagonal Number
static int triacontakaihenagonalNum(int n)
{
    return (29 * n * n - 27 * n) / 2;
}
  
// Driver Code
public static void Main (string[] args)
{
      
    // Given number
    int n = 3;
  
    // Function call
    Console.Write(triacontakaihenagonalNum(n));
}
}
  
// This code is contributed by rock_cool

Javascript

// Javascript implementation for the
// above approach
 
    // Function to find the Nth
    // triacontakaihenagonal Number
    function triacontakaihenagonalNum( n)
    {
        return (29 * n * n - 27 * n) / 2;
    }
 
    // Driver Code
      
    // Given number
    let n = 3;
     
    // Function call
    document.write(triacontakaihenagonalNum(n));
 
// This code is contributed by Rajput-Ji

输出：

90