Python中的巴恩斯利蕨类植物

巴恩斯利蕨类植物是由数学家迈克尔巴恩斯利创造的分形形状。这种分形的几何特征类似于天然蕨类植物，因此得名。 Barnsley fern 是通过对 Barnsley 引入的四个数学方程（称为迭代函数系统 (IFS)）进行大量迭代而创建的。

巴恩斯利使用的变换有以下公式：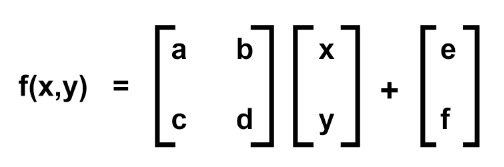

其中，字母具有以下值：

a	b	c	d	e	f	p	PART
0	0	0	0.16	0	0	0.01	Stem
0.85	0.04	-0.04	0.85	0	1.60	0.85	Small Leaflet
0.20	-0.26	0.23	0.22	0	1.60	0.07	Large Leaflet(Left)
-0.15	0.28	0.26	0.24	0	0.44	0.07	Large Leaflet(Right)

“p”是概率。

因此，四个方程是：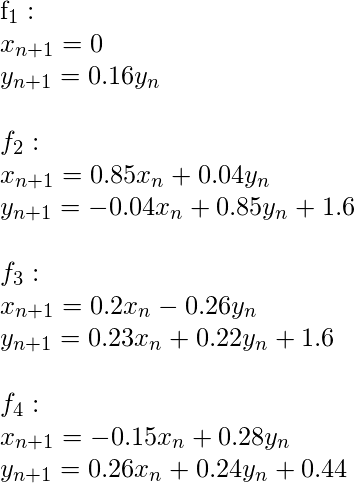
$f_1 :\\ x_{n+1} = 0\\ y_{n+1} = 0.16 y_n\\ \\ f_2 :\\ x_{n+1} = 0.85 x_n + 0.04 y_n\\ y_{n+1} = -0.04 x_n + 0.85 y_n + 1.6\\ \\ f_3 :\\ x_{n+1} = 0.2 x_n - 0.26 y_n\\ y_{n+1} = 0.23 x_n + 0.22 y_n + 1.6\\ \\ f_4 :\\ x_{n+1} = -0.15 x_n + 0.28 y_n\\ y_{n+1} = 0.26 x_n + 0.24 y_n + 0.44\\$
在上述方程的帮助下，蕨类植物被创造出来。现在让我们看看 Python3 的实现。

# importing necessary modules
import matplotlib.pyplot as plt
from random import randint
  
# initializing the list
x = []
y = []
  
# setting first element to 0
x.append(0)
y.append(0)
  
current = 0
  
for i in range(1, 50000):
  
    # generates a random integer between 1 and 100
    z = randint(1, 100)
  
    # the x and y coordinates of the equations
    # are appended in the lists respectively.
      
    # for the probability 0.01
    if z == 1:
        x.append(0)
        y.append(0.16*(y[current]))
      
    # for the probability 0.85    
    if z>= 2 and z<= 86:
        x.append(0.85*(x[current]) + 0.04*(y[current]))
        y.append(-0.04*(x[current]) + 0.85*(y[current])+1.6)
      
    # for the probability 0.07    
    if z>= 87 and z<= 93:
        x.append(0.2*(x[current]) - 0.26*(y[current]))
        y.append(0.23*(x[current]) + 0.22*(y[current])+1.6)
      
    # for the probability 0.07    
    if z>= 94 and z<= 100:
        x.append(-0.15*(x[current]) + 0.28*(y[current]))
        y.append(0.26*(x[current]) + 0.24*(y[current])+0.44)
          
    current = current + 1
   
plt.scatter(x, y, s = 0.2, edgecolor ='green')
  
plt.show()        

输出 ：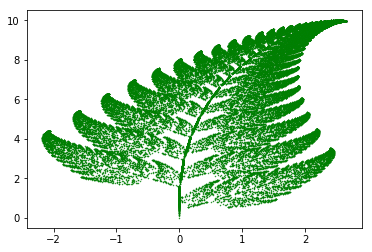

注意：整个输出取决于方程的系数。一个实验可能是改变系数并每次都得到一个新的模式。