有障碍的矩阵中可能的最长路径

给定一个M x N矩阵，任意设置几个障碍，计算矩阵中从源到目的地的可能的最长路径的长度。我们只能移动到不是障碍的相邻单元格。该路线不能包含任何对角线移动，并且无法再次访问在特定路径中曾经访问过的位置。

例如，下面的矩阵突出显示了从源到目的地没有障碍的最长路径。路径的长度是24。

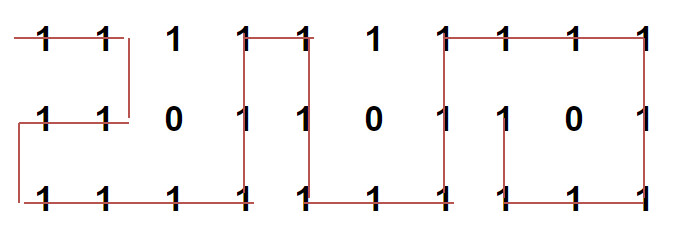
这个想法是使用回溯。我们从矩阵的源单元格开始，在所有允许的四个方向上前进，然后递归检查它们是否导致求解。如果找到了目标，则我们将更新最长路径的值，否则，如果以上解决方案均无效，则我们将从函数返回false。

以下是上述想法的C++实现–

// C++ program to find Longest Possible Route in a
// matrix with hurdles
#include 
using namespace std;
#define R 3
#define C 10
  
// A Pair to store status of a cell. found is set to
// true of destination is reachable and value stores
// distance of longest path
struct Pair
{
    // true if destination is found
    bool found;
  
    // stores cost of longest path from current cell to
    // destination cell
    int value;
};
  
// Function to find Longest Possible Route in the
// matrix with hurdles. If the destination is not reachable
// the function returns false with cost INT_MAX.
// (i, j) is source cell and (x, y) is destination cell.
Pair findLongestPathUtil(int mat[R][C], int i, int j,
    int x, int y, bool visited[R][C])
{
  
    // if (i, j) itself is destination, return true
    if (i == x && j == y)
    {
        Pair p = { true, 0 };
        return p;
    }
  
    // if not a valid cell, return false
    if (i < 0 || i >= R || j < 0 || j >= C ||
            mat[i][j] == 0 || visited[i][j])
    {
        Pair p = { false, INT_MAX };
        return p;
    }
  
    // include (i, j) in current path i.e.
    // set visited(i, j) to true
    visited[i][j] = true;
  
    // res stores longest path from current cell (i, j) to
    // destination cell (x, y)
    int res = INT_MIN;
  
    // go left from current cell
    Pair sol = findLongestPathUtil(mat, i, j - 1, x, y, visited);
  
    // if destination can be reached on going left from current
    // cell, update res
    if (sol.found)
        res = max(res, sol.value);
  
    // go right from current cell
    sol = findLongestPathUtil(mat, i, j + 1, x, y, visited);
  
    // if destination can be reached on going right from current
    // cell, update res
    if (sol.found)
        res = max(res, sol.value);
  
    // go up from current cell
    sol = findLongestPathUtil(mat, i - 1, j, x, y, visited);
  
    // if destination can be reached on going up from current
    // cell, update res
    if (sol.found)
        res = max(res, sol.value);
  
    // go down from current cell
    sol = findLongestPathUtil(mat, i + 1, j, x, y, visited);
  
    // if destination can be reached on going down from current
    // cell, update res
    if (sol.found)
        res = max(res, sol.value);
  
    // Backtrack
    visited[i][j] = false;
  
    // if destination can be reached from current cell,
    // return true
    if (res != INT_MIN)
    {
        Pair p = { true, 1 + res };
        return p;
    }
  
    // if destination can't be reached from current cell,
    // return false
    else
    {
        Pair p = { false, INT_MAX };
        return p;
    }
}
  
// A wrapper function over findLongestPathUtil()
void findLongestPath(int mat[R][C], int i, int j, int x,
                                                  int y)
{
    // create a boolean matrix to store info about
    // cells already visited in current route
    bool visited[R][C];
  
    // initailize visited to false
    memset(visited, false, sizeof visited);
  
    // find longest route from (i, j) to (x, y) and
    // print its maximum cost
    Pair p = findLongestPathUtil(mat, i, j, x, y, visited);
    if (p.found)
        cout << "Length of longest possible route is "
             << p.value;
  
    // If the destination is not reachable
    else
        cout << "Destination not reachable from given source";
}
  
// Driver code
int main()
{
    // input matrix with hurdles shown with number 0
    int mat[R][C] =
    {
        { 1, 1, 1, 1, 1, 1, 1, 1, 1, 1 },
        { 1, 1, 0, 1, 1, 0, 1, 1, 0, 1 },
        { 1, 1, 1, 1, 1, 1, 1, 1, 1, 1 }
    };
  
    // find longest path with source (0, 0) and
    // destination (1, 7)
    findLongestPath(mat, 0, 0, 1, 7);
  
    return 0;
}

输出：

Length of longest possible route is 24