在日常生活中,我们会遇到很多物体,需要找到它们的体积和表面积。例如,我们可能必须计算水箱可以容纳多少水。为此,我们需要知道水箱的形状,此外,我们还需要知道如何计算水箱的体积。有时,某些实体是不同标准形状的组合。

上图显示了一些基本的实体形状。对象通常由这些形状的组合组成。但是有时候我们可以通过移除一部分来从实体中制作出另一种形状。例如,Frustum。在这种形状下,我们有兴趣去除圆锥的一部分。让我们详细研究视锥。
视锥
让我们假设一个正确的圆锥形并从其中除去一部分。让我们用平行于底面的平面切割圆锥。

切掉小上圆锥体后保留的形状称为平截头体。我们每个人在日常生活中都已经看到过这种形状,我们的水杯也处于这种形状。因此,视锥可以定义为
If a right circular cone is cut off by a plane parallel to it’s base, the shape of the portion between the cutting plane and the base plane is called frustum of that cone.
让我们看一下这种形状的体积和表面积。
视锥的体积
上面给出的视锥的定义指出,视锥是圆锥体的切面部分。因此,要计算平截头体的体积,我们只需要计算较大和较小圆锥体的体积之差即可。
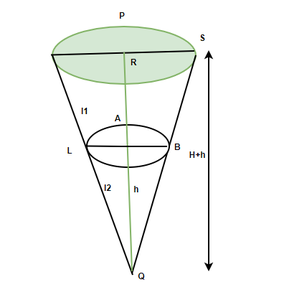
在上面给出的图中,我们假设
- 圆锥体的总高度为“ H + h”
- 总倾斜高度为“ l1 + l2”
- 较大的圆锥半径= R
- 切锥的半径= r
圆锥的总体积V 1 = ![]()
被移除的较小圆锥体的体积V 2 = ![]()
现在,我们来计算平截头体(V)的体积
V = V 1 – V 2
= ![]() –
– ![]()
= ![]()
现在,使用三角函数中的切线函数公式,我们可以得出,
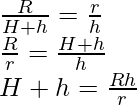
在“体积”公式中插入此H + h值。
V = ![由QuickLaTeX.com渲染 \\ \frac{1}{3} \pi [R^2(\frac{Rh}{r} - r^2h] \\ = \frac{1}{3}\pi \frac{[R^3h - r^3h]}{r}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Frustum%20of%20a%20Cone_9.jpg)
再次使用相似三角形的属性,我们将找出h的值。
将h的这个值代入公式中,我们得到
![由QuickLaTeX.com渲染 \\ V = \frac{1}{3}\pi \frac{[R^3h - r^3h]}{r} \\ = \frac{1}{3} \pi \frac{h(R^3 - r^3)}{r} \\ = \frac{1}{3} \pi \frac{\frac{Hr}{R - r}(R^3 - r^3)}{r} \\ = \frac{1}{3} \pi H(R^2 + r^2 + Rr) \\](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Frustum%20of%20a%20Cone_11.jpg)
截锥体的表面积
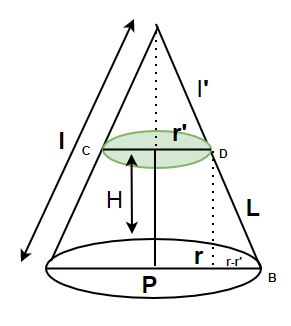
类似于体积,表面积也将等于较大圆锥体和较小圆锥体的表面积之差。
大圆锥体的曲面面积=πRl
较小圆锥体的曲面面积=πrl’
表面积之差=π(Rl – rl’)
在上面给出的图中,三角形OAB和OCD是相似的。所以,
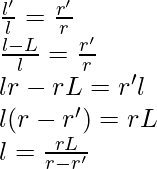
让我们计算一下底边的周长
C =2πr和C’=2πr’
因此,视锥A的总弯曲表面积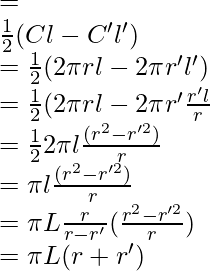
此处,L是由下式给出的倾斜高度,L = ![]()
截锥体的总表面积=截锥体的总弯曲表面积A +两个基座的面积
让我们看看与我们刚得出的这些公式有关的一些问题。
样本问题
问题1:找出一个圆锥台的截头圆锥体的体积,该圆锥体的高度为20cm,两个基座的半径分别为5cm和8cm。
解决方案:
Using the formula studied above,
![]()
We are given, H = 20cm, r = 5cm and R = 8cm. Plugging these values in the equation.
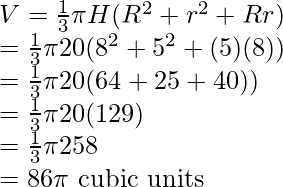
问题2:找出圆锥台的截锥体的表面积和总表面积,该截锥体的高度为10cm,两个基座的半径分别为4cm和8cm。
解决方案:
We know the formula for surface area and total surface area of the frustum. We need to plug in the required values.
Curved Surface area of the frustum = ![]()
Where L ![]()
Given, H = 10cm, r = 4cm and r’ = 8cm
Calculating the value of L, L = 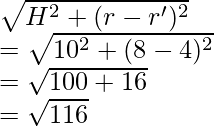
Curved Surface area of the frustum =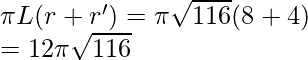
Total Surface Area = Curved surface are of the frustum + Area of both the bases
= 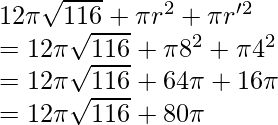
问题3:假设我们有一个开放的金属桶,其高度为50厘米,底座的半径为10厘米和20厘米。找到用于制造铲斗的金属板的面积。

解决方案:
Bucket is in the form of frustum which closed from the bottom. We need to calculate the total surface area of this frustum.
Given : H = 50cm, r1 = 10cm and r2 = 20cm.
Curved surface area of the frustum S = ![]()
Let’s calculate L first,

Putting this value in the surface area of the formula,
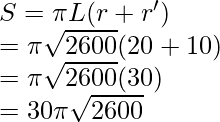
This is the curved surface. To find the total area (A) of the metal sheet used,
A = S + Area of the bottom
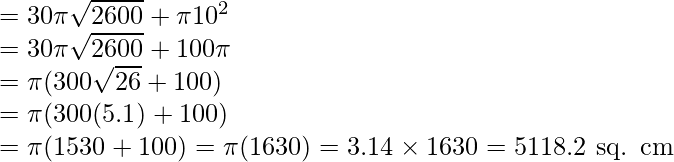
问题4:如果截头锥体的高度为5y cm,半径为y和2y,则求出截头锥体的体积表达式。
解决方案:
Using the formula studied above,
![]()
We are given, H = 5y, r = y and R = 2y. Plugging these values in the equation.
