四面体和八面体空隙

晶体固体的空间晶格和紧密原子堆积将它们区分开来。然而，这些结构包含空隙，这是它们排列中的间隙。许多研究人员一致认为，原子之间的距离对物质的特性有重大影响。让我们看看四面体和八面体空隙。

什么是空隙？

In close packing of spheres, some hollows or voids are left blank. These vacancies in the crystal are called interstitial Voids or interstitial sites or simply, voids. The two essential interstitial voids are Tetrahedral Voids and Octahedral Voids.

排列两层后由两种类型的坯料组成的球体的包装。由四个球体形成的空隙称为四面体空隙，由六个球体形成的空隙称为八面体空隙。

间隙空位或间隙位点的存在在过渡金属的化学中起着重要作用。由于金属原子之间的空间，过渡金属可以很容易地容纳较小的非金属原子，例如氢、硼、碳和氮。这些化合物称为间隙化合物。

空隙类型

四面体空隙

第二层中的球体放置在第一层中彼此接触的三个球体的顶部。这些球体的中心位于四面体的顶部。空隙的形状很可能不是四面体，而是围绕这个空隙的排列是四面体。具有四面体排列的四个球体之间的空间称为四面体空隙或四面体空间。晶体每个原子有两个四面体空隙。

可以很容易地计算出晶格中四面体空隙的数量。在这种情况下，空隙的数量将是球体（即晶胞）数量的两倍。结果，将有“2n”个四面体空隙。

真空的体积远小于球体的体积。因为空隙出现在四个球体的中心，所以四面体空隙的配位数为四。

四面体空隙

Relationship among the Radius of the Tetrahedral Void and the Radius of the atoms in Close Packing- A tetrahedral void is perhaps represented by placing four spheres at the disjunctive corners of a cube. It may be noted that in a stable tetrahedral arrangement there are four spheres at the corners touching each other. However, for the sake of simplicity, spheres are shown by distant circles. Exactly all the spheres are touching each other. Let the length of each side of the cube be one cm and the radius of the tetrahedron void is r and the radius of the sphere is R.

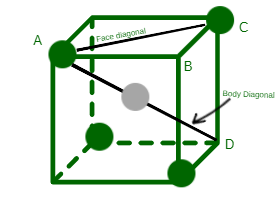
A tetrahedral void

AC² = AB² + BC²

AC = √(AB² + BC²)

     = √(a²+ a²) = √2 × a 

A and C on the diagonal of the face

AC = R + R = 2R

2R = √2 a or R = (√2 × a)/2                                                                                                                                                               …(i)

Now in right angled triangle ACD, AD is the diagonal of the body and

AD² = AC² + CD²

AD = √(AC² + CD²)

     = √2a² + a² = √3a

The tetrahedron presents us at the center of the diagonal AD of the body so that half the length of this diagonal is equal to the sum of the radii of R and r. Thus,

R + r = (AD)/2 = (√(3) × a)/2                                                                                                                                                         …(ii)

Dividing eq. (ii) by (i) get

(R + r)/R = (√(3a))/2 × 2/(√(2a)) 

             = (√3)/(√2)

1 + r/R = (√3)/(√2)

      r/R = (√3)/(√2) – 1 = (√3 – √2)/(√2)

           = (1.732-1.414)/1.414

       r = 0.225 R

Thus, for an atom to occupy a tetrahedral void, its radius must be 0.225 times the radius of the sphere.

八面体空隙

八面体空间是在六个圆的中心形成的一种空间或空隙。从图中可以看出，每个八面体空隙都是由第一层和第二层的三角形空隙组合而成的。由两个等边三角形对边的顶点形成的空隙称为八面体空隙或八面体部位。因此，这个空隙在正八面体的顶点被 6 个球体包围。晶体每个原子有一个八面体空隙。

结果，当第一层的四面体空隙和第二层的四面体空隙对齐时形成八面体空隙。在这里，六个球体的中心形成了一个虚空。所以八面体空隙的配位数为六。

如果结构中的球体数量为“n”，则八面体空隙的数量将相同。 “n”就是一个很好的例子。

八面体空隙

Relationship among the radius of the Octahedral void and the radius of the atoms in close packing-

An octahedral void is surrounded by 6 spheres, only 4 are shown.

Octahedral void with radius r

Suppose that the length of the unit cell is a cm and radius of octahedral void is r and the radius of sphere is R.

If the length of the unit cell is a cm, then at right angle ABC,

AB = BC = a cm

The diagonal AC is:-

AC = √(AB² + BC²) = √(a² +a²) = √(2a)

(AC)/(AB) = (√(2) × a)/a = √2/1

AC = R + 2r + R 

AC = 2R + 2r

AB = 2R

1 + r/R = (√(2))/1 

or 

r/R = √(2) – 1

      = 1.414 – 1 

      = 0.414

   r = 0.414R

Thus, for an atom to occupy an octahedral void, its radius has to be 0.414 times the radius of the sphere. Hence, a tetrahedral void is much smaller than an octahedral void.

八面体和四面体空隙的数量

• 每个球体有两个四面体空隙，每个球体只有一个八面体空隙。因此，在 N 个球体的密集排列结构中，有：
  • 四面体空隙 = 2N
  • 八面体空隙 = N
• 四面体和八边形空位的总数 = N 协调数
• 与给定球体接触的球体数称为坐标数。因此，配位数是晶格中任何组成粒子的最近（或最近）邻居的数量。
• 一个球体与中心原子同一平面上的其他 6 个壳层成比例关系。它在其顶层接触三个球体，在其底层接触三个球体。因此，在六方密排（hcp）和立方密排（ccp）排列中，其配位数为 12。
• 据推测，配位数 4、6、8 和 12 在不同类型的晶体中更为常见。

四面体和八面体空隙之间的区别

Tetrahedral Voids	Octahedral voids
The space between four spheres having a tetrahedral arrangement is called a tetrahedral void or a tetrahedral site.	The void formed by the vertices on opposite sides by two equilateral triangles is called octahedron al void or octahedral site.
Tetrahedral voids = 2N	Octahedral voids = N
The radius must be 0.225 times the radius of the sphere.	The radius has to be 0.414 times the radius of the sphere.
Tetrahedral vacancies can be seen on the sides of the unit cell.	Octahedral voids can be seen in the centre of the unit cell.
There are two tetrahedral vacancies per sphere in the space lattice.	There are two octahedral vacancies per sphere in the crystal lattice.

示例问题

问题 1. 表示 fcc 和 bcc 晶胞中原子半径 (r) 和边长 (a) 的关系，其中 a = 边长。

回答：

For face centered cubic unit cell, radius = a/(2√(2)) = (√(2) × a)/4

For body-centered cubic unit cell, radius = (√(3) × a)/4

问题 2. 在简单立方晶格的情况下，填充的百分比效率是多少？

回答：

A simple cubic mesh has a packing capacity of 52.4%.

问题 3. 为什么将液体和气体归类为流体？

回答：

Liquids and gases have the property of flow i.e. molecules of liquids and gases can easily move past and fall freely on each other. Because they are used to flow, they are classified as liquids.

问题 4. 为什么固体不可压缩？

回答：

In a solid, the internal distance between the constituent particles (atoms, molecules, or ions) is very small. Bringing them closer, there will be great repulsive forces between the electron clouds of these particles. Therefore, the solid cannot be compressed.

问题 5. 尽管粒子排列具有长程有序性，为什么晶体通常并不完美？

回答：

During the crystallization process, some deviations from the ideal ordered arrangement may occur. As a result, crystals are usually not perfect.

问题 6. 为什么食盐 NaCl 有时会呈黄色？

回答：

The yellow colour of sodium chloride crystals is due to excess impurities of the metal. In this defect, the unpaired electrons get trapped in the anion vacancies. These sites are called F-centers. The yellow colour results from the excitation of these electrons when they absorb energy from visible light falling on the crystal.