容量和容量–测定八级数学

测量是数学的一个分支，它讨论不同几何对象的长度，体积和面积。形状可以是2-D或3-D。我们找到了3D对象的体积和2D对象的面积。

什么是音量?

体积是给定固体所占总空间的量度。体积是在3-D中定义的，因为要具有体积，对象必须具有三个变量，即长度，宽度或厚度或宽度以及高度。

留在中空主体内部的空间总量与主体所占据的空间之间的差是图形的体积。

现在，让我们以称为长方体的3d形状为例，它是一个以六个矩形为边界的实心图形，因此具有六个矩形面。长方体的尺寸如下：长度l，宽度b和高度h.height

长方体的体积为：

Volume = length * height * width

体积适用于实心和空心物体。例如立方体，长方体，圆锥体，圆柱体等。

什么是容量?

当空心物体充满液体或空气，并且呈该物体或容器的形状时。填充在容器内部的水和空气的总体积称为容器的容量。

Note: Capacity is calculated only for hollow object.

示例：圆锥体，圆柱体，空心半球等。

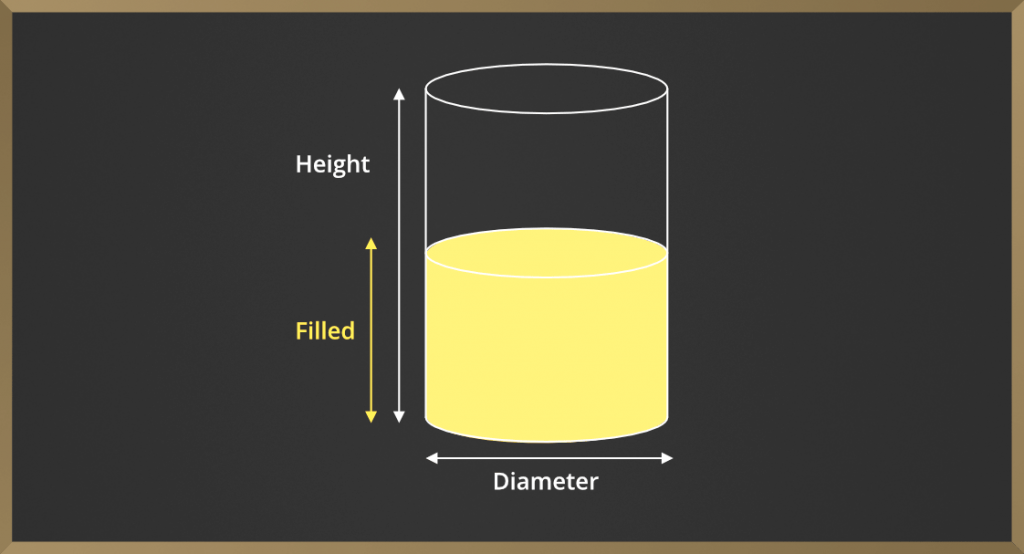
示例：找到半径为14厘米，高度为21厘米的圆柱体的体积和容量。

解：我们给出，r = 14cm，h = 21cm；

圆柱体体积=πr* r * r * h。

V = 22/7 * 14 * 14 * 21

V = 12,936cm3。

容量为12,936 / 1000升……. as(1000cm ³ = 1升)

= 12.936升。

Note: The unit of capacity is litres(l) and millilitres(ml).

某些封闭数字的确定

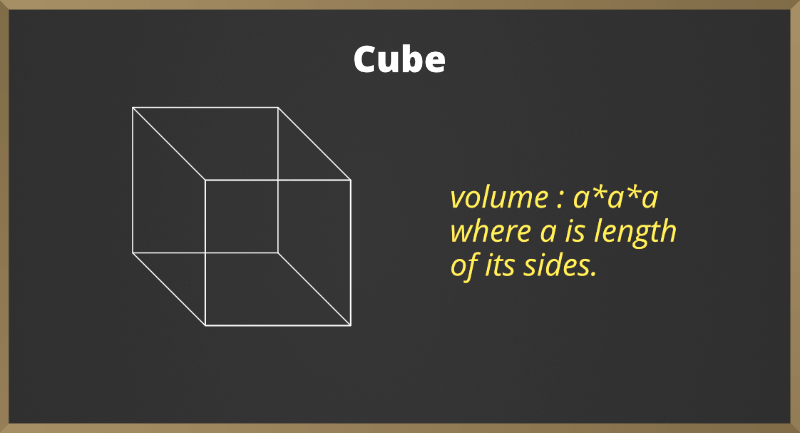
立方体

立方体的表面积定义为立方体每个面的面积。例如，我们知道立方体的每一面都是相等的。因此，一张脸的面积是长×长或宽×宽或高×高中的三个，我们根据问题选择一个。  

因此，立方体的总表面积为6 *长度*长度。因为立方体有六个面，每个面都有一个长*长的区域。

现在立方体的体积是它的中空部分，可以写成长*长*长或宽*宽*宽或高*高*高。并且它的单位将是立方的。

示例：找到体积为625cm ³的立方体的宽度。

解决方案：

We know that volume of the cube is a * a * a =a³ where a  can be the length, width, height.

So, a³ = 125 then

a = 5cm ( 5 is cube root of 125)

So width is 5 cm.

长方体

长方体的表面积定义为长方体的表面积，但区别在于，所有侧面都不相等，并且长方体的长度，宽度，高度不同。因此，其表面积将是每个面的面积，然后加上所有面积，我们将获得总表面积。

因此，长方体的总表面积为长*宽+宽*高+高*长+长*宽+宽*高+高*长，即每六个面的面积之和

所以总表面积是

2 * (length * width + width * height + height * length)

长方体的体积是长方体内部的中空部分，

Volume = length * width * height

示例：发现长方体的高度为625cm ³ ，其底面积为25cm ² 。

解决方案：

Base area of cube means width * length this forms base in cube

As we know volume of cube is 

=> l * b * h = 625

=> h = 625 / b * l

=> h = 625 / 25

=> h = 25cm

So the height is 25 cm

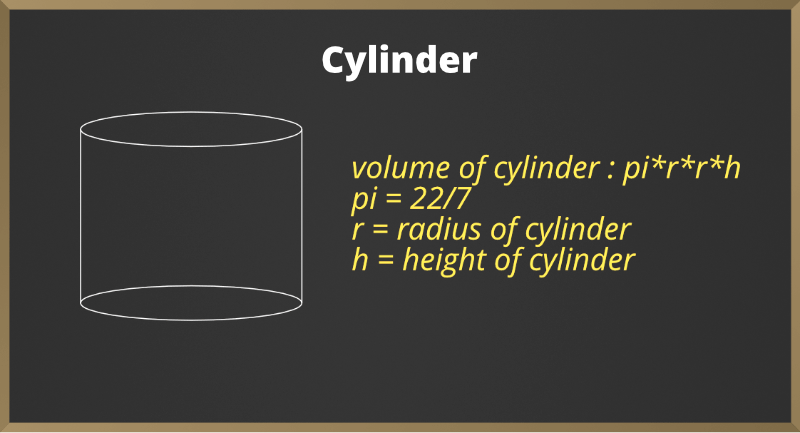
圆筒

在这里，我们谈论的是用于圆柱，直管灯，水管等的右圆形圆柱体。圆柱体的体积是圆柱体内的中空部分。

Volume of Cylinder = 22/7 * r * r * h

Here, 

r = radius of cylinder and h = height of cylinder

示例：将长11厘米，宽4厘米的矩形纸卷成4厘米高的圆柱。气缸的容积是多少?

解决方案：

Let the cylinder if radius = r and its height = h

Perimeter of  base of cylinder = 2 * pi * r = 11cm

                                                => 2 * 22/7 * r = 11cm

                                                => r = 7/4 cm

Volume = 22/7 * r* r * h

=> 22/7 * 7/4 * 7/4 * 4 

=> 38.5cm³

Hence, the volume of cylinder is 38.5cm³

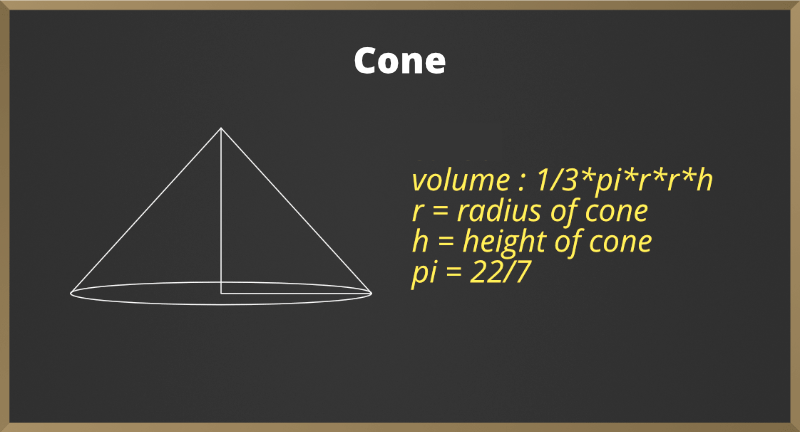
锥体

圆锥体的体积是圆锥体内的中空部分。

Volume = 1/3 * 22/7 * r * r * h

Here, 

h = height of cone

r = radius of cone

容量和容量示例问题

问题1：以立方厘米为单位，找到长，宽，高分别为1 m，1m和1m的立方罐的容量。

解决方案：

First we convert between volume and capacity in cm³ and ml so we need to find volumes in cm³

The volume is 1m * 1m * 1m = 1m³  as it is cube so we used cube formula

As 1m = 100cm so volume in cm³ is 100cm * 100cm * 100cm = 1000000cm³

As 1cm³ = 1ml So the capacity of the tank in ml is 1000000ml.

and finally capacity of tank in litre is 1000000/1000=1000litres.

As 1000ml is 1 litre. So the capacity of tank is 1000litres.

问题2：一个坦克长2m，1m，2m。找到罐的容量。

解决方案：

As we know that 1m = 100cm.

As it is cuboid figure because its length is different. 

Volume of cuboid = length * width * height = 2m * 1m * 2m = 4m^3.

Or 200cm * 100cm * 200cm = 4000000cm³.

We know that, 1cm³ = 1ml so 4000000cm³ = 4000000ml

As 1000ml = 1litre,  then 4000000ml is 4000000/1000 = 4000 litres is capacity of tank.

问题3：一张矩形纸被卷成半径为10cm的圆柱体，圆柱体的体积是多少?矩形的宽度是7厘米。

解决方案：

Width of the rectangle becomes the height of the cylinder and the radius of the cylinder is given as 10 cm.

So the value of cylinder is V = 22/7 * r * r * h

V = 22/7 * 10 * 10 * 7 

v = 22 * 10 * 10

v = 2200cm³.

问题4：如果半径为9厘米，高度为14厘米，那么圆锥的体积是多少?

解决方案：

The volume of cone = 1/3 * pi * r * r * h 

Hence, volume is 1/3 * 22/7 * 9 * 9 * 14

1 * 22 * 3 * 9 * 2 = 1,188cm³.