测量是数学的一个分支,涉及几何形状,其面积,体积和其他相关参数的研究。将一对彼此相对的平行边和另一对不平行的非平行边的四边闭合图形称为梯形。
梯形的性质
- 它是一个四面闭合图形,内角之和为360°。
- 一对平行的边,应该彼此相对。
- 一对不平行的侧面。
- 相邻边的角度之和为180°。
- 梯形的对角线在相交处彼此一分为二。
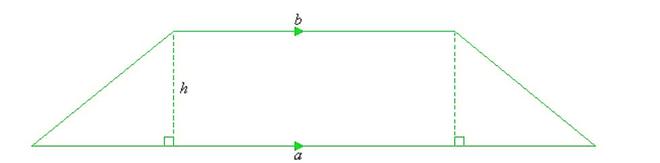
梯形的基本术语
Base of a Trapezium: The pair of parallel sides which are opposite to each other are called base. You can call as b1 and b2 respectively.
Height of a Trapezium: The perpendicular distance between the two parallel lines is called as Height of the trapezium.
梯形面积公式
如果给出了梯形的底边和高度,则可以借助以下公式来计算梯形的面积:

梯形的面积= 1/2 x(基数之和)x(梯形的高度)
梯形面积的推导
梯形的面积等于两个三角形的面积与矩形的面积之和。以下是计算梯形面积的推导:
既然我们知道:
Area of trapezium = Area of triangle 1 + area of rectangle + Area of triangle 2
让我们假设三角形1的底为B1,三角形2的底为B2,两个三角形的高度均为h。对于矩形,假设其宽度和高度分别为b1和h。
就是说
A = (B1 x h / 2) + b1h + (B2 x h / 2)
A = (B1 x h + 2b1h + B2 x h) / 2
简化方程式,重新排列各项,并将因式分解为:
A = h / 2[b1 + (B1 + b1 + B2] ….(i)
If we assume the longer base of the trapezoid be b2, then
b2 = B1 + b1 + B2 …..(ii)
Substituting (ii) in equation (i),
A = h / 2(b1 + b2)
因此,底面为b1,b2和高度为h的梯形的面积为;
A = h/2(b1+b2)
也可以写成如下
Area of Trapezium = 1 / 2 x (sum of bases) x (Height of trapezium)
= 1 / 2 x ( b1 + b2) x h
基于公式的样本问题
问题1:计算梯形的面积,其中底值分别为10和5,梯形的高度为2 m。
解决方案:
Since we know that,
Area of trapezium = 1/2 x (sum of bases) x (Height of trapezium)
= 1/2 x (10 + 5) x 2
= 15 m^2
问题2:给定梯形的面积为120 m ^ 2,梯形的高度为6m,底边之一为4m。计算另一个碱基的长度。
解决方案:
Area of trapezium = 1/2 x(sum of bases) x ( Height of trapezium)
Let value of other base is b2
Putting all the given values in the above formula, we got
120 = 1/2 x(4+b2) x 6
120 = (4+b2) x3
40 = (4+b2)
b2 = 36 meter
问题3:给定梯形的面积为220 m ^ 2,一个底面为6m,另一个底面为4m。计算梯形的高度。
解决方案:
Area of trapezium = 1/2 x (sum of bases) x ( Height of trapezium)
Let value of height be h.
Putting all the given values in the above formula, we got
220 = 1/2 x (4 + 6) x h
220 = 10 x h
22 = h
h = 22m