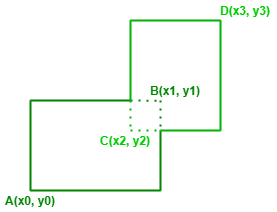
给定两个数组X[]和Y[] ,每个数组的长度为4 ,其中(X[0], Y[0])和(X[1], Y[1])表示一个数组的左下角和右上角矩形和(X[2], Y[2])和(X[3], Y[3]) 分别代表另一个矩形的左下角和右上角,任务是找到矩形的外边界的周长两个矩形的并集如下所示。
例子:
Input: X[] = {-1, 2, 0, 4}, Y[] = {2, 5, -3, 3}
Output: 26
Explanation: Required Perimeter = 2 * ( (4 – (-1)) + (5 – (-3)) ) = 2*(8 + 5) = 26.
Input: X[] = {-3, 1, 1, 4}, Y[] = {-2, 3, 1, 5}
Output: 26
Explanation: Required Perimeter = 2 * ( (4 – (-3)) + (5 – (-2)) ) = 2*(7 + 7) = 28.
处理方法:按照以下步骤解决问题:
- 检查给定点形成的矩形是否相交。
- 如果发现相交,那么周长可以通过公式2*((X[1] – X[0]) + (X[3] – X[2]) + (Y[1] – Y[ 0]) + (Y[3] – Y[2])) 。
- 否则,分别打印两次X和Y坐标之间最大差异的总和,即2 * (max(X[]) – min(X[]) + max(Y[]) – min(Y[])) 。
下面是上述方法的实现:
C++
// C++ program for the above approach
#include
using namespace std;
// Function to check if two
// rectangles are intersecting or not
bool doIntersect(vector X,
vector Y)
{
// If one rectangle is to the
// right of other's right edge
if (X[0] > X[3] || X[2] > X[1])
return false;
// If one rectangle is on the
// top of other's top edge
if (Y[0] > Y[3] || Y[2] > Y[1])
return false;
return true;
}
// Function to return the perimeter of
// the Union of Two Rectangles
int getUnionPerimeter(vector X,
vector Y)
{
// Stores the resultant perimeter
int perimeter = 0;
// If rectangles do not interesect
if (!doIntersect(X, Y)) {
// Perimeter of Rectangle 1
perimeter
+= 2 * (abs(X[1] - X[0])
+ abs(Y[1] - Y[0]));
// Perimeter of Rectangle 2
perimeter
+= 2 * (abs(X[3] - X[2])
+ abs(Y[3] - Y[2]));
}
// If the rectangles intersect
else {
// Get width of combined figure
int w = *max_element(X.begin(),
X.end())
- *min_element(X.begin(),
X.end());
// Get length of combined figure
int l = *max_element(Y.begin(),
Y.end())
- *min_element(Y.begin(),
Y.end());
perimeter = 2 * (l + w);
}
// Return the perimeter
return perimeter;
}
// Driver Code
int main()
{
vector X{ -1, 2, 4, 6 };
vector Y{ 2, 5, 3, 7 };
cout << getUnionPerimeter(X, Y);
} Java
// Java program for the above approach
import java.util.*;
class GFG
{
// Function to check if two
// rectangles are intersecting or not
static boolean doIntersect(int []X,
int []Y)
{
// If one rectangle is to the
// right of other's right edge
if (X[0] > X[3] || X[2] > X[1])
return false;
// If one rectangle is on the
// top of other's top edge
if (Y[0] > Y[3] || Y[2] > Y[1])
return false;
return true;
}
// Function to return the perimeter of
// the Union of Two Rectangles
static int getUnionPerimeter(int []X,
int []Y)
{
// Stores the resultant perimeter
int perimeter = 0;
// If rectangles do not interesect
if (!doIntersect(X, Y)) {
// Perimeter of Rectangle 1
perimeter
+= 2 * (Math.abs(X[1] - X[0])
+ Math.abs(Y[1] - Y[0]));
// Perimeter of Rectangle 2
perimeter
+= 2 * (Math.abs(X[3] - X[2])
+ Math.abs(Y[3] - Y[2]));
}
// If the rectangles intersect
else {
// Get width of combined figure
int w = Arrays.stream(X).max().getAsInt()
- Arrays.stream(X).min().getAsInt();
// Get length of combined figure
int l = Arrays.stream(Y).max().getAsInt()
- Arrays.stream(Y).min().getAsInt();
perimeter = 2 * (l + w);
}
// Return the perimeter
return perimeter;
}
// Driver Code
public static void main(String[] args)
{
int []X = { -1, 2, 4, 6 };
int []Y = { 2, 5, 3, 7 };
System.out.print(getUnionPerimeter(X, Y));
}
}
// This code is contributed by 29AjayKumarPython3
# Python3 program for the above approach
# Function to check if two
# rectangles are intersecting or not
def doIntersect(X, Y):
# If one rectangle is to the
# right of other's right edge
if (X[0] > X[3] or X[2] > X[1]):
return False
# If one rectangle is on the
# top of other's top edge
if (Y[0] > Y[3] or Y[2] > Y[1]):
return False
return True
# Function to return the perimeter of
# the Union of Two Rectangles
def getUnionPerimeter(X, Y):
# Stores the resultant perimeter
perimeter = 0
# If rectangles do not interesect
if (not doIntersect(X, Y)):
# Perimeter of Rectangle 1
perimeter += 2 * (abs(X[1] - X[0]) + abs(Y[1] - Y[0]))
# Perimeter of Rectangle 2
perimeter += 2 * (abs(X[3] - X[2]) + abs(Y[3] - Y[2]))
# If the rectangles intersect
else:
# Get width of combined figure
w = max(X) - min(X)
# Get length of combined figure
l = max(Y) - min(Y)
perimeter = 2 * (l + w)
# Return the perimeter
return perimeter
# Driver Code
if __name__ == '__main__':
X = [ -1, 2, 4, 6]
Y = [ 2, 5, 3, 7 ]
print (getUnionPerimeter(X, Y))
# This code is contributed by mohit kumar 29.C#
// C# program for the above approach
using System;
using System.Linq;
public class GFG
{
// Function to check if two
// rectangles are intersecting or not
static bool doIntersect(int []X,
int []Y)
{
// If one rectangle is to the
// right of other's right edge
if (X[0] > X[3] || X[2] > X[1])
return false;
// If one rectangle is on the
// top of other's top edge
if (Y[0] > Y[3] || Y[2] > Y[1])
return false;
return true;
}
// Function to return the perimeter of
// the Union of Two Rectangles
static int getUnionPerimeter(int []X,
int []Y)
{
// Stores the resultant perimeter
int perimeter = 0;
// If rectangles do not interesect
if (!doIntersect(X, Y))
{
// Perimeter of Rectangle 1
perimeter
+= 2 * (Math.Abs(X[1] - X[0])
+ Math.Abs(Y[1] - Y[0]));
// Perimeter of Rectangle 2
perimeter
+= 2 * (Math.Abs(X[3] - X[2])
+ Math.Abs(Y[3] - Y[2]));
}
// If the rectangles intersect
else
{
// Get width of combined figure
int w = X.Max()
- X.Min();
// Get length of combined figure
int l = X.Max()
- Y.Min();
perimeter = 2 * (l + w);
}
// Return the perimeter
return perimeter;
}
// Driver Code
public static void Main(String[] args)
{
int []X = { -1, 2, 4, 6 };
int []Y = { 2, 5, 3, 7 };
Console.Write(getUnionPerimeter(X, Y));
}
}
// This code contributed by shikhasingrajputJavascript
输出:
24时间复杂度: O(1)
辅助空间: O(1)
如果您希望与专家一起参加现场课程,请参阅DSA 现场工作专业课程和学生竞争性编程现场课程。