热膨胀

当谈到液体时，可以观察到，当温度计放在微温的水中时，温度计中的水银会上升。当我们从热水中取出温度计时，水银水平会下降。同样，当气球在凉爽的房间中充气一半并放入温水中时，它会膨胀到最大尺寸。相反，当一个完全充气的气球浸入冷水中时，由于内部空气的收缩，它会开始收缩。

值得注意的是，一些带有金属盖的密封瓶拧得非常紧，以至于必须将盖子浸入热水中几分钟才能打开盖子。这会使金属盖膨胀，并使盖子更容易拧开。

热膨胀

Most substances expand when heated and contract when cooled. When a body’s temperature changes, its dimensions change as well. The term “thermal expansion” refers to the expansion of a body’s size as its temperature rises. 

在固体中可以发生三种类型的膨胀，

1. 线性膨胀
2. 区域表面或表面扩张
3. 音量扩展

线性膨胀

Linear expansion refers to the increment in the length of a solid on heating. If the substance is in the form of a long rod, a little variation in temperature, ΔT will cause it to deform, the fractional change in length, Δl/l, is directly proportional to ΔT.

线性展开的表达式是，

$\dfrac{\Delta l}{l} = \alpha _{l}\cdot\Delta T$

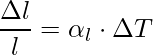
其中α _l是给定固体的线性膨胀系数。

α的单位在 CGS 中是每摄氏度 ( °C ^-1 )，在 SI 系统中是每开尔文 (K ^-1 )。

某些材料的线性膨胀系数值如下表所示。

Materials	αl (10–5 K–1)
Aluminum	2.5
Brass	1.8
Iron	1.2
Copper	1.7
Silver	1.9
Gold	1.4
Glass (pyrex)	0.32
Lead	0.29

表面扩张或面积扩张

Superficial or Area expansion refers to the increment in the surface area of the substance on heating. There is a little variation in temperature, ΔT will cause it to deform, the fractional change in surface area, ΔA/A, is directly proportional to ΔT.

面积扩展的表达式是，

$\dfrac{\Delta A}{A} = \alpha _{A}\cdot\Delta T$

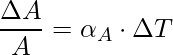
这里， α _A是给定固体的面积膨胀系数。

音量扩展

The volume expansion refers to the fractional change in the volume of the substance. There is a little variation in temperature, ΔT will cause it to deform, the fractional change in the volume, ΔV/V, is directly proportional to ΔT. 

面积扩展的表达式是，

$\dfrac{\Delta V}{V} = \alpha _{V}\cdot\Delta T$

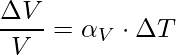
这里α _V也是物质的一个特性，但它不会保持不变，它通常取决于温度。

体积膨胀系数只有在高温下才会保持不变。例如，酒精（乙基）具有比汞更高的体积膨胀系数，因此对于相同的温度升高膨胀更多。

下面的图表显示了作为温度函数的铜的体积膨胀系数。

铜的体积膨胀系数随温度的变化。

水的异常膨胀

The anomalous expansion of water is an abnormal property of water whereby it expands instead of contracting when the temperature goes from 4 °C to 0°C, and it becomes less dense. 

• 当加热到 0 到 4 摄氏度之间时，水会表现出不寻常的行为。当一定量的水从室温冷却时，它的体积会下降，直到温度接近 4°C。
• 温度低于4°C，水的体积增加，因此密度降低。这意味着水的最大密度为 4 °C。该属性对水体有重大影响。湖泊和池塘首先在顶部结冰。
• 水在大气中失去能量，变得更稠密，随着温度接近 4°C 的湖水下沉；温度较低的水向底部上升。当顶部较冷的水冷却到 4 摄氏度以下时，它会变得不那么稠密并沉降到表面，在那里结冰。

水的热膨胀。

样题

问题 1：定义术语热膨胀。

解决方案：

Most substances expand when heated and contract when cooled.  When a body’s temperature changes, its dimensions change as well. The term “thermal expansion” refers to the expansion of a body’s size as its temperature rises.

There are three types of expansions that can take place in solids,

• Linear expansion
• Area superficial or superficial expansion
• Volume expansion

问题 2：当温度升高 100 °C 时，必须对长度为 10 cm 的钢丝的末端施加多大的压力才能保持其长度不变。 （钢的杨氏模量为 2 x 10 ¹¹ Nm ^-2 ，热膨胀系数为 1.1 x 10 ^-5 K ^-1 ）。

解决方案：

Given, 

ΔT = 100 °C,

Y = 2 × 1011 N m^–2

α = 1.1 × 10^–5 K^–1

The expression for thermal strain is 

 ΔF/A=Y(Δl/l)

          = YαΔT

Thermal stress in a rod is the pressure due to the thermal strain.

Substitute the value in the above expression.

Thermal strain=(2 × 10¹¹ Nm^-2)× (1.1 × 10^–5 K^-1)×( 100 °C) 

                       = 2.2 × 10⁸ Pa

问题3：两根电线由相同的材料制成并且具有相同的体积。然而，导线1具有横截面积A，而导线2具有横截面积3A。如果在施加力 F 时导线 1 的长度增加了 Dx，那么需要多大的力才能将导线 2 拉伸相同的量？

解决方案：

Young’s modulus is the same for same substance.

The area of the cross-section for the wire 1 is A and wire 2 is 3A.

The volume of wire 1 and 2 is

 V₁ = V₂

 (A × l₁ )= (3A × l₂)

l₂ = l₁/3

Y = (F/A)/(Δl/l) 

or F₁ = YA(Δl₁/l₁)

Similarly,

F₂ = Y3A(Δl₂/l₂)

Wire 2 is stretch by the same amount therefore, 

Δl₁ = Δl₂ = x 

F₂ = Y3Ax /(l₁/3) 

F₂ = 9(YAx/l₁) 

F₂ = 9F₁

问题 4：定义术语区域扩展。

解决方案：

Superficial or Area expansion refers to the increment in the surface area of the substance on heating. There is a little variation in temperature, ΔT will cause it to deform, the fractional change in surface area, ΔA/A, is directly proportional to ΔT.

The expression for the Area expansion is

$\frac{\Delta A}{A} = \alpha _{A}\cdot\Delta T$

Here, α_A is the coefficient of area expansion of the given solid.

问题 5：推导面积膨胀系数 ( α _A ) 和线性膨胀系数 ( α _l ) 之间的关系。

解决方案：

Consider a cube with a length of l that expands evenly in all directions as its temperature rises by T. The original area will be length square and the new area, after a temperature increase is,

A+ΔA=(l+Δl)²

A+ΔA=l²+(Δl)²+2lΔl

The terms in (Δl)² have been neglected since Δl is small compared to l.

A+ΔA≈l²+2lΔl

or A+ΔA=A+(2AΔl)/l

or ΔA≈ 2AΔl/l

Rearrange the above equation,

or ΔA/A≈ 2(Δl/l)

or α_A=2α_l

Therefore, the coefficient of area expansion is twice the coefficient of linear expansion.