从给定的二叉搜索树创建一个波数组

给定一个二叉搜索树，任务是从给定的二叉搜索树创建一个波数组。如果 arr[0] >= arr[1] <= arr[2] >= arr[3] <= arr[4] >= ...，则数组arr[0..n-1]称为波数组

例子：

Input:

Output: 4 2 8 6 12 10 14
Explanation: The above mentioned array {4, 2, 8, 6, 12, 10, 14} is one of the many valid wave arrays.

Input:

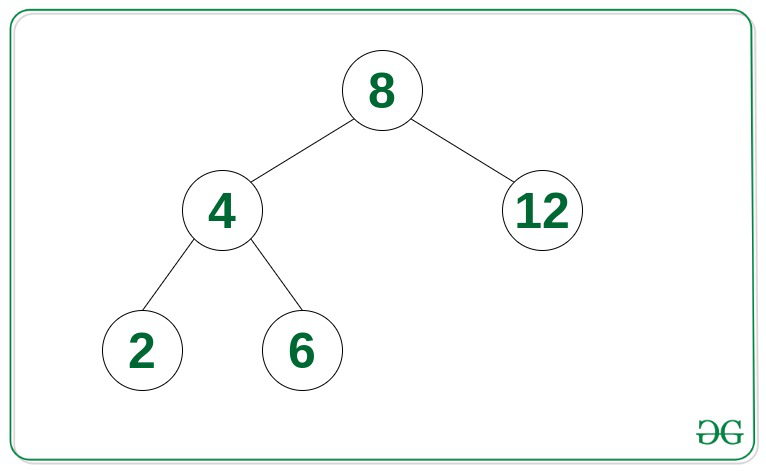
Output: 4 2 8 6 12 

方法：给定问题可以通过观察二叉搜索树的中序遍历以非递减顺序给出节点来解决。因此，将给定树的中序遍历存储到向量中。由于向量包含按排序顺序排列的元素，因此可以使用本文中讨论的方法，通过将[0, N)范围内的所有元素交换相邻元素来将其转换为波数组。

下面是上述方法的实现：

C++

// C++ program for the above approach
#include 
using namespace std;
 
// Node of the Binary Search tree
struct Node {
    int data;
    Node* right;
    Node* left;
 
    // Constructor
    Node(int data)
    {
        this->data = data;
        this->left = NULL;
        this->right = NULL;
    }
};
 
// Function to convert Binary Search
// Tree into a wave Array
void toWaveArray(Node* root)
{
    // Stores the final wave array
    vector waveArr;
 
    stack s;
    Node* curr = root;
 
    // Perform the Inorder traversal
    // of the given BST
    while (curr != NULL || s.empty() == false) {
 
        // Reach the left most Node of
        // the curr Node
        while (curr != NULL) {
 
            // Place pointer to a tree node
            // in stack before traversing
            // the node's left subtree
            s.push(curr);
            curr = curr->left;
        }
        curr = s.top();
        s.pop();
 
        // Insert into wave array
        waveArr.push_back(curr->data);
 
        // Visit the right subtree
        curr = curr->right;
    }
 
    // Convert sorted array into wave array
    for (int i = 0;
         i + 1 < waveArr.size(); i += 2) {
        swap(waveArr[i], waveArr[i + 1]);
    }
 
    // Print the answer
    for (int i = 0; i < waveArr.size(); i++) {
        cout << waveArr[i] << " ";
    }
}
 
// Driver Code
int main()
{
    Node* root = new Node(8);
    root->left = new Node(4);
    root->right = new Node(12);
    root->right->left = new Node(10);
    root->right->right = new Node(14);
    root->left->left = new Node(2);
    root->left->right = new Node(6);
 
    toWaveArray(root);
 
    return 0;
}

Java

// Java program for the above approach
import java.util.*;
 
class GFG{
 
// Node of the Binary Search tree
static class Node {
    int data;
    Node right;
    Node left;
 
    // Constructor
    Node(int data)
    {
        this.data = data;
        this.left = null;
        this.right = null;
    }
};
 
// Function to convert Binary Search
// Tree into a wave Array
static void toWaveArray(Node root)
{
   
    // Stores the final wave array
    Vector waveArr = new Vector<>();
 
    Stack s = new Stack<>();
    Node curr = root;
 
    // Perform the Inorder traversal
    // of the given BST
    while (curr != null || s.isEmpty() == false) {
 
        // Reach the left most Node of
        // the curr Node
        while (curr != null) {
 
            // Place pointer to a tree node
            // in stack before traversing
            // the node's left subtree
            s.add(curr);
            curr = curr.left;
        }
        curr = s.peek();
        s.pop();
 
        // Insert into wave array
        waveArr.add(curr.data);
 
        // Visit the right subtree
        curr = curr.right;
    }
 
    // Convert sorted array into wave array
    for (int i = 0;   i + 1 < waveArr.size(); i += 2) {
        int t = waveArr.get(i);
        waveArr.set(i, waveArr.get(i+1));
        waveArr.set(i+1, t);
         
    }
 
    // Print the answer
    for (int i = 0; i < waveArr.size(); i++) {
        System.out.print(waveArr.get(i)+ " ");
    }
}
 
// Driver Code
public static void main(String[] args)
{
    Node root = new Node(8);
    root.left = new Node(4);
    root.right = new Node(12);
    root.right.left = new Node(10);
    root.right.right = new Node(14);
    root.left.left = new Node(2);
    root.left.right = new Node(6);
 
    toWaveArray(root);
 
}
}
 
// This code is contributed by umadevi9616

Python3

# Python program for the above approach
 
# Node of the Binary Search tree
class Node:
    def __init__(self, data):
        self.data = data;
        self.right = None;
        self.left = None;
         
# Function to convert Binary Search
# Tree into a wave Array
def toWaveArray(root):
 
    # Stores the final wave array
    waveArr = [];
 
    s = [];
    curr = root;
 
    # Perform the Inorder traversal
    # of the given BST
    while (curr != None or len(s) != 0):
 
        # Reach the left most Node of
        # the curr Node
        while (curr != None):
 
            # Place pointer to a tree Node
            # in stack before traversing
            # the Node's left subtree
            s.append(curr);
            curr = curr.left;
         
        curr = s.pop();
 
        # Insert into wave array
        waveArr.append(curr.data);
 
        # Visit the right subtree
        curr = curr.right;
     
    # Convert sorted array into wave array
    for i in range(0,len(waveArr)-1, 2):
        t = waveArr[i];
        waveArr[i] = waveArr[i + 1];
        waveArr[i + 1]= t;
 
    # Print the answer
    for i in range(len(waveArr)):
        print(waveArr[i], end=" ");
 
# Driver Code
if __name__ == '__main__':
    root = Node(8);
    root.left = Node(4);
    root.right = Node(12);
    root.right.left = Node(10);
    root.right.right = Node(14);
    root.left.left = Node(2);
    root.left.right = Node(6);
 
    toWaveArray(root);
 
 
# This code is contributed by Rajput-Ji

C#

// C# program for the above approach
using System;
using System.Collections.Generic;
 
public class GFG{
 
// Node of the Binary Search tree
public class Node {
    public int data;
    public Node right;
    public Node left;
 
    // Constructor
    public Node(int data)
    {
        this.data = data;
        this.left = null;
        this.right = null;
    }
};
 
// Function to convert Binary Search
// Tree into a wave Array
static void toWaveArray(Node root)
{
   
    // Stores the readonly wave array
    List waveArr = new List();
 
    Stack s = new Stack();
    Node curr = root;
 
    // Perform the Inorder traversal
    // of the given BST
    while (curr != null || s.Count!=0 ) {
 
        // Reach the left most Node of
        // the curr Node
        while (curr != null) {
 
            // Place pointer to a tree node
            // in stack before traversing
            // the node's left subtree
            s.Push(curr);
            curr = curr.left;
        }
        curr = s.Peek();
        s.Pop();
 
        // Insert into wave array
        waveArr.Add(curr.data);
 
        // Visit the right subtree
        curr = curr.right;
    }
 
    // Convert sorted array into wave array
    for (int i = 0;   i + 1 < waveArr.Count; i += 2) {
        int t = waveArr[i];
        waveArr[i]= waveArr[i+1];
        waveArr[i+1]= t;
         
    }
 
    // Print the answer
    for (int i = 0; i < waveArr.Count; i++) {
        Console.Write(waveArr[i]+ " ");
    }
}
 
// Driver Code
public static void Main(String[] args)
{
    Node root = new Node(8);
    root.left = new Node(4);
    root.right = new Node(12);
    root.right.left = new Node(10);
    root.right.right = new Node(14);
    root.left.left = new Node(2);
    root.left.right = new Node(6);
 
    toWaveArray(root);
}
}
 
// This code is contributed by umadevi9616

Javascript

        // JavaScript Program to implement
        // the above approach
        class Node {
            constructor(data) {
                this.data = data;
                this.left = this.right = null;
            }
        }
        // Function to convert Binary Search
        // Tree into a wave Array
        function toWaveArray(root) {
            // Stores the final wave array
            let waveArr = [];
 
            let s = [];
            let curr = root;
 
            // Perform the Inorder traversal
            // of the given BST
            while (curr != null || s.length != 0) {
 
                // Reach the left most Node of
                // the curr Node
                while (curr != null) {
 
                    // Place pointer to a tree node
                    // in stack before traversing
                    // the node's left subtree
                    s.push(curr);
                    curr = curr.left;
                }
                curr = s[s.length - 1];
                s.pop();
 
                // Insert into wave array
                waveArr.push(curr.data);
 
                // Visit the right subtree
                curr = curr.right;
            }
 
            // Convert sorted array into wave array
            for (let i = 0;
                i + 1 < waveArr.length; i += 2) {
                let temp = waveArr[i]
                waveArr[i] = waveArr[i + 1]
                waveArr[i + 1] = temp
            }
 
            // Print the answer
            for (let i = 0; i < waveArr.length; i++) {
                document.write(waveArr[i] + " ");
            }
        }
 
        // Driver Code
        let root = new Node(8);
        root.left = new Node(4);
        root.right = new Node(12);
        root.right.left = new Node(10);
        root.right.right = new Node(14);
        root.left.left = new Node(2);
        root.left.right = new Node(6);
 
        toWaveArray(root);
 
     // This code is contributed by Potta Lokesh
 
    

输出：

4 2 8 6 12 10 14

时间复杂度： O(N)
辅助空间： O(N)