3D中的一对线之间的角度

给定3D平面中三个点A(x1，y1，z1)，B(x2，y2，z2)和C(x3，y3，z3)的坐标，其中B是线AB和BC的交点是找到线AB和BC之间的夹角。

例子：

Input: x1 = 1, y1 = 3, z1 = 3; x2 = 3, y2 = 4, z2 = 5; x3 = 5, y3 = 6, z3 = 9;
Output: 54.6065

Input: x1 = 10, y1 = 10, z1 = 10; x2 = 0, y2 = 0, z2 = 0; x3 = 15, y3 = 10, z3 = 15;
Output: 56.4496

方法：

1. 根据给定的坐标，找到线AB和线BC的方程，它们的方向比为：

   AB = (x1 – x2)i + (y1 – y2)j + (z1 – z2)k
   BC = (x3 – x2)i + (y3 – y2)j + (z3 – z2)k

2. 使用AB和BC线两个方向比率的cosθ公式，可得出AB和BC线之间的夹角余弦为：

   $cos \Theta = \frac{(AB. BC)}{\left | AB \right |\left | BC \right |}$
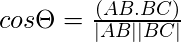
   where,
   AB.BC is the dot product of direction ratios AB and BC.
   |AB| is the magnitude of line AB
   |BC| is the magnitude of line BC

   假设有两个方向比率：

   A = ai + bj + ck
   B = xi + yj + zk
   

   然后

   Dot Product(A.B) = a*x + b*y + c*z
   magnitude of A = |A| =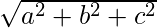 $\sqrt{a^{2} + b^{2} + c^{2}}$
   magnitude of B = |B| = $\sqrt{x^{2} + y^{2} + z^{2}}$

3. 计算出的角度的余弦值以弧度表示余弦值。为了找到角度，将余弦值乘以(180 /Π) 。

下面是上述方法的实现：

C++

// C++ program for the above approach
  
#include "bits/stdc++.h"
#define PI 3.14
using namespace std;
  
// Function to find the angle between
// the two lines
void calculateAngle(
    int x1, int y1, int z1,
    int x2, int y2, int z2,
    int x3, int y3, int z3)
{
    // Find direction ratio of line AB
    int ABx = x1 - x2;
    int ABy = y1 - y2;
    int ABz = z1 - z2;
  
    // Find direction ratio of line BC
    int BCx = x3 - x2;
    int BCy = y3 - y2;
    int BCz = z3 - z2;
  
    // Find the dotProduct
    // of lines AB & BC
    double dotProduct
        = ABx * BCx
          + ABy * BCy
          + ABz * BCz;
  
    // Find magnitude of
    // line AB and BC
    double magnitudeAB
        = ABx * ABx
          + ABy * ABy
          + ABz * ABz;
    double magnitudeBC
        = BCx * BCx
          + BCy * BCy
          + BCz * BCz;
  
    // Find the cosine of
    // the angle formed
    // by line AB and BC
    double angle = dotProduct;
    angle /= sqrt(
        magnitudeAB * magnitudeBC);
  
    // Find angle in radian
    angle = (angle * 180) / PI;
  
    // Print the angle
    cout << abs(angle) << endl;
}
  
// Driver Code
int main()
{
  
    // Given coordinates
    // Points A
    int x1 = 1, y1 = 3, z1 = 3;
  
    // Points B
    int x2 = 3, y2 = 4, z2 = 5;
  
    // Points C
    int x3 = 5, y3 = 6, z3 = 9;
  
    // Function Call
    calculateAngle(x1, y1, z1,
                   x2, y2, z2,
                   x3, y3, z3);
  
    return 0;
}

Java

// Java program for the above approach
import java.util.*;
  
class GFG{
      
// Function to find the angle 
// between the two lines
static void calculateAngle(int x1, int y1, int z1,
                           int x2, int y2, int z2,
                           int x3, int y3, int z3)
{
      
    // Find direction ratio of line AB
    int ABx = x1 - x2;
    int ABy = y1 - y2;
    int ABz = z1 - z2;
  
    // Find direction ratio of line BC
    int BCx = x3 - x2;
    int BCy = y3 - y2;
    int BCz = z3 - z2;
  
    // Find the dotProduct
    // of lines AB & BC
    double dotProduct = ABx * BCx +
                        ABy * BCy + 
                        ABz * BCz;
  
    // Find magnitude of
    // line AB and BC
    double magnitudeAB = ABx * ABx + 
                         ABy * ABy + 
                         ABz * ABz;
    double magnitudeBC = BCx * BCx + 
                         BCy * BCy + 
                         BCz * BCz;
  
    // Find the cosine of the 
    // angle formed by line
    // AB and BC
    double angle = dotProduct;
    angle /= Math.sqrt(magnitudeAB * magnitudeBC);
  
    // Find angle in radian
    angle = (angle * 180) / 3.14;
  
    // Print the angle
    System.out.printf("%.4f", Math.abs(angle));
}
  
// Driver code
public static void main(String[] args)
{
  
    // Given coordinates
    // Points A
    int x1 = 1, y1 = 3, z1 = 3;
  
    // Points B
    int x2 = 3, y2 = 4, z2 = 5;
  
    // Points C
    int x3 = 5, y3 = 6, z3 = 9;
  
    // Function Call
    calculateAngle(x1, y1, z1,
                   x2, y2, z2,
                   x3, y3, z3);
}
}
  
// This code is contributed by offbeat

Python3

# Python3 program for the above approach
import math
  
# Function to find the angle 
# between the two lines
def calculateAngle(x1, y1, z1, 
                   x2, y2, z2,
                   x3, y3, z3):
                         
    # Find direction ratio of line AB
    ABx = x1 - x2;
    ABy = y1 - y2;
    ABz = z1 - z2;
  
    # Find direction ratio of line BC
    BCx = x3 - x2;
    BCy = y3 - y2;
    BCz = z3 - z2;
  
    # Find the dotProduct
    # of lines AB & BC
    dotProduct = (ABx * BCx + 
                  ABy * BCy + 
                  ABz * BCz);
  
    # Find magnitude of
    # line AB and BC
    magnitudeAB = (ABx * ABx + 
                   ABy * ABy + 
                   ABz * ABz);
    magnitudeBC = (BCx * BCx + 
                   BCy * BCy +
                   BCz * BCz);
  
    # Find the cosine of
    # the angle formed
    # by line AB and BC
    angle = dotProduct;
    angle /= math.sqrt(magnitudeAB * 
                       magnitudeBC);
  
    # Find angle in radian
    angle = (angle * 180) / 3.14;
  
    # Print angle
    print(round(abs(angle), 4))
  
# Driver Code
if __name__=='__main__': 
  
    # Given coordinates
    # Points A
    x1, y1, z1 = 1, 3, 3;
  
    # Points B
    x2, y2, z2 = 3, 4, 5;
  
    # Points C
    x3, y3, z3 = 5, 6, 9;
   
    # Function Call
    calculateAngle(x1, y1, z1,
                   x2, y2, z2,
                   x3, y3, z3);
  
# This code is contributed by AbhiThakur

C#

// C# program for the above approach
using System;
class GFG{
      
// Function to find the angle 
// between the two lines
static void calculateAngle(int x1, int y1, 
                           int z1, int x2, 
                           int y2, int z2,
                           int x3, int y3, 
                           int z3)
{
      
    // Find direction ratio of line AB
    int ABx = x1 - x2;
    int ABy = y1 - y2;
    int ABz = z1 - z2;
  
    // Find direction ratio of line BC
    int BCx = x3 - x2;
    int BCy = y3 - y2;
    int BCz = z3 - z2;
  
    // Find the dotProduct
    // of lines AB & BC
    double dotProduct = ABx * BCx +
                        ABy * BCy + 
                        ABz * BCz;
  
    // Find magnitude of
    // line AB and BC
    double magnitudeAB = ABx * ABx + 
                         ABy * ABy + 
                         ABz * ABz;
    double magnitudeBC = BCx * BCx + 
                         BCy * BCy + 
                         BCz * BCz;
  
    // Find the cosine of the 
    // angle formed by line
    // AB and BC
    double angle = dotProduct;
    angle /= Math.Sqrt(magnitudeAB * 
                       magnitudeBC);
  
    // Find angle in radian
    angle = (angle * 180) / 3.14;
  
    // Print the angle
    Console.Write(String.Format("{0:F4}", Math.Abs(angle)));
}
  
// Driver code
public static void Main()
{
  
    // Given coordinates
    // Points A
    int x1 = 1, y1 = 3, z1 = 3;
  
    // Points B
    int x2 = 3, y2 = 4, z2 = 5;
  
    // Points C
    int x3 = 5, y3 = 6, z3 = 9;
  
    // Function Call
    calculateAngle(x1, y1, z1,
                   x2, y2, z2,
                   x3, y3, z3);
}
}
  
// This code is contributed by Code_Mech

输出：

54.6065