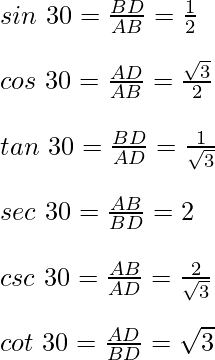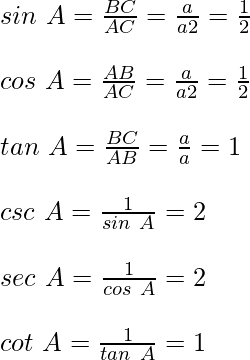一些特定角度的三角比

三角学是关于三角形的，或更确切地说，是关于直角三角形的角度和边之间的关系的。在本文中，我们将讨论直角三角形的边相对于其锐角的比率，称为角度的三角比，并找到特定角度的三角比：0°，30°，45°，60°，和90°。

考虑以下三角形：

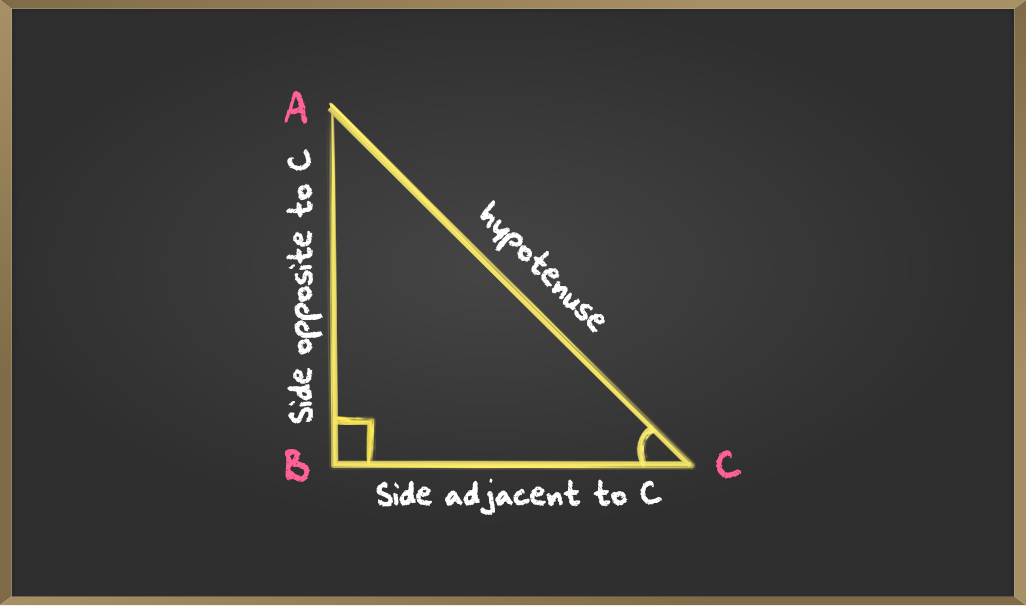
BA边与角度∠BCA相反，因此我们将BA称为∠C的相反边，AC是斜边，另一边BC是∠C的相邻边。

角C的三角比

Sine: Sine of ∠C is the ratio between BA and AC that is the ratio between the side opposite to C and the hypotenuse.

$sin\, C = \frac{BA}{AC}$
 
Cosine: Cosine of ∠C is the ratio between BC and AC that is the ratio between the side adjacent to C and the hypotenuse.

$cos\, C = \frac{BC}{AC}$
 
Tangent: Tangent of ∠C is the ratio between BA and BC that is the ratio between the side opposite and adjacent to C

$tan\, C = \frac{BA}{BC}$
 
Cosecant: Cosecant of ∠C is the reciprocal of sin C that is the ratio between the hypotenuse and the side opposite to C.

$csc\, C = \frac{BA}{AC}$
 
Secant: Secant of ∠C is the reciprocal of cos C that is the ratio between the hypotenuse and the side adjacent to C.
$sec\, C = \frac{BA}{AC}$
 
Cotangent: Cotangent of ∠C is the reciprocal of tan C that is the ratio between the side adjacent to C and side opposite to C.
$cot\, C = \frac{BA}{AC}$

查找角度0°，30°，45°，60°，90°的三角比

A.角度为0°和90 °

如果角度A = 0°，则对边的长度将为零，斜边=相邻边；如果A = 90°，则斜边=对边。因此，通过对三角比使用上述公式，以及斜边的长度是否为a 。

if A = 0°
 
$\\ sin A = \frac{BC}{AC} = \frac{0}{a} = 0 \\\quad\\ cos A = \frac{AB}{AC} = \frac{a}{a} =1 \\\quad\\ tan A = \frac{BC}{AB} = \frac{a}{a} = 0 \\\quad\\ csc A = \frac{AC}{BC} = not\, defined \\\quad\\ sec A = \frac{AC}{AB} = 1 \\\quad\\ cot A = \frac{AB}{BC} = not\, defined \\\quad\\$

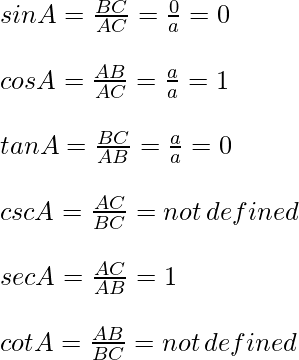
if A = 90°
 
$\\ sin A = \frac{AB}{AC} = \frac{0}{a} = 1 \\\quad\\ cos A = \frac{BC}{AC} = \frac{a}{a} = 0 \\\quad\\ tan A = \frac{AB}{BC} = \frac{a}{a} = not\, defined \\\quad\\ csc A = \frac{AC}{AB} = 1 \\\quad\\ sec A = \frac{AC}{BC} = not\, defined \\\quad\\ cot A = \frac{BC}{AB} = 0$

此处csc 0，cot 0，tan 90和sec 90未定义为特定角度，该角度除以未定义的0。

B.对于30°和60 °的角度

考虑等边三角形ABC。由于等边三角形中的每个角度均为60°，因此，

∠A=∠B=∠C= 60°。

∆ABD是一个直角三角形，与D成直角，∠BAD= 30°，∠ABD= 60°，

这里的ΔADB和ΔADC相似，因为它们是同等三角形(CPCT)的对应部分。

$In\, \Delta ABD\;,AB=a\,,BD=\frac{a}{2} \\and\,AB^2=BD^2+AD^2\\ \quad\\\implies AD^2=AB^2-BD^2 \\ \quad\\\implies AD^2=a^2-(\frac{a}{2})^2\\ \quad\\ \implies AD^2=a^2-\frac{a^2}{4} \\ \quad\\ \implies AD^2=\frac{3a^2}{4} \\\quad\\ \implies AD= \frac{\sqrt{3} a}{2}$

现在我们知道了AB，BD和AD的值，因此角度30的三角比为

$sin\ 30=\frac{BD}{AB}=\frac{1}{2} \\ \quad\\cos\ 30=\frac{AD}{AB}=\frac{\sqrt{3}}{2} \\ \quad\\tan\ 30=\frac{BD}{AD}=\frac{1}{\sqrt{3}} \\\quad\\sec\ 30=\frac{AB}{BD}=2 \\\quad\\csc\ 30=\frac{AB}{AD}=\frac{2}{\sqrt{3}} \\\quad\\cot\ 30=\frac{AD}{BD}=\sqrt{3}$

角度60°

$sin\ 60=\frac{AD}{AB}=\frac{\sqrt{3}}{2} \\ \quad\\cos\ 60=\frac{BD}{AB}=\frac{1}{2} \\\quad\\tan\ 60=\frac{AD}{BD}=\sqrt{3} \\\quad\\sec\ 60=\frac{AB}{AD}=\frac{2}{\sqrt{3}} \\\quad\\csc\ 60=\frac{AB}{BD}=2 \\\quad\\cot\ 60=\frac{BD}{AD}=\frac{1}{\sqrt{3}}$

C.角度为45°

在直角三角形中，如果一个角度为45°，则另一个角度也为45°，因此使其成为等腰直角三角形

如果边BC = a的长度，则AB = a的长度，AC(斜边)的长度为a√2，则

$sin\ A = \frac{BC}{AC} = \frac{a}{a√2} = \frac{1}{√2}\\ \quad\\ cos\ A = \frac{AB}{AC} = \frac{a}{a√2} = \frac{1}{√2}\\ \quad\\ tan\ A = \frac{BC}{AB} = \frac{a}{a} = 1\\ \quad\\ csc\ A = \frac{1}{sin\ A} = √2\\ \quad\\ sec\ A = \frac{1}{cos\ A} = √2\\ \quad\\ cot\ A = \frac{1}{tan\ A} = 1\\$

结果：

∠A	0°	30°	45°	60°	90°
sin A	0	1/2	1/√2	√3/2	1
cos A	1	√3/2	1/√2	1/2	0
tan A	0	1/√3	1	√3	Not defined
sec A	Not defined	2	√2	2/√3	1
csc A	1	2/√3	√2	2	Not defined
cot A	Not defined	√3	1	1/√3	0