水平和垂直切割后蛋糕的最大面积

给定两个正整数h和w，表示形成矩形的高度h和宽度w 。此外，还有两个整数数组horizontalCuts和verticalCuts ，其中水平切割[i]是从矩形顶部到第i 个水平切割的距离，同样， verticalCuts[j]是从矩形左侧到第j 个垂直切割的距离切。任务是找到矩形的最大面积 你切在阵列horizontalCuts和verticalCuts提供的每个水平和垂直位置之后。由于答案可能是一个巨大的数字，因此返回此模 10^9 + 7。

例子 ：

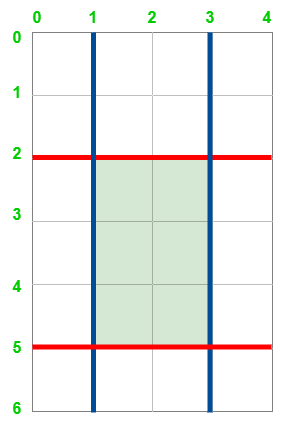
最大面积 = 6

Input: h = 6, w = 4, horizontalCuts = [2, 5], verticalCuts = [1, 3]
Output: 6
Explanation: The figure above represents the given rectangle. Red lines are the horizontal cuts and blue lines are vertical cuts. After the rectangle is cut, the green piece of rectangle has the maximum area.

Input: h = 5, w = 4, horizontalCuts = [3, 1], verticalCuts = [1]
Output: 9
 

方法：这个问题可以通过观察来解决——

• 如果horizontalCuts 垂直于任何VerticalCut，则所有垂直切片都穿过所有horizontalCuts。
• 接下来，矩形的最大面积必须被至少一个垂直和一个水平的切割所包围。

由上面的观察可知，我们需要分别求出两个横切和两个纵切之间的最大距离，并将它们相乘，求出矩形的面积。请按照以下步骤解决问题：

• 对horizontalCuts和verticalCuts数组进行排序。
• 初始化两个变量，比如MaxHorizontal和MaxVertical为 0，它们将分别存储矩形的最大水平和垂直长度。
• 使用变量i在范围[1, horizontalCuts.size()-1] 中迭代并执行以下步骤：
  • 将MaxHorizontal的值修改为max(MaxHorizontal, horizontalCuts[i] – horizontalCuts[i-1]) 。
  • 将MaxVertical的值修改为max(MaxVertical, verticalCuts[i] – verticalCuts[i-1]) 。
• 打印MaxHorizontal*MaxVertical作为答案。

下面是上述方法的实现：

C++

// C++ Program for the above approach
#include 
using namespace std;
const int mod = 1e9 + 7;
 
class Solution {
public:
    // Returns the maximum area of rectangle
    // after Horizontal and Vertical Cuts
    int maxArea(int h, int w, vector& horizontalCuts,
                vector& verticalCuts)
    {
 
        // Sort the two arrays
        sort(horizontalCuts.begin(), horizontalCuts.end());
        sort(verticalCuts.begin(), verticalCuts.end());
 
        // Insert the right bound h and w
        // in their respective vectors
        horizontalCuts.push_back(h);
        verticalCuts.push_back(w);
 
        int maxHorizontal = 0;
        int maxVertical = 0;
 
        // Find the maximum Horizontal Length possible
        for (int i = 1; i < horizontalCuts.size(); i++) {
            int diff
                = horizontalCuts[i] - horizontalCuts[i - 1];
            maxHorizontal = max(maxHorizontal, diff);
        }
 
        // Find the maximum vertical Length possible
        for (int i = 1; i < verticalCuts.size(); i++) {
            int diff
                = verticalCuts[i] - verticalCuts[i - 1];
            maxVertical = max(maxVertical, diff);
        }
 
        // Return the maximum area of rectangle
        return (int)((long)maxHorizontal * maxVertical
                     % mod);
    }
};
 
// Driver Code
int main()
{
    // Class Call
    Solution ob;
   
    // Given Input
    vector hc = { 2, 5 }, vc = { 1, 3 };
    int h = 6, v = 4;
    // Function Call
    cout << (ob.maxArea(6, 4, hc, vc));
    return 0;
}

Java

// Java program for above approach
import java.awt.*;
import java.util.*;
class GFG{
 
    final int mod = (int) (1e9 + 7);
 
    // Returns the maximum area of rectangle
    // after Horizontal and Vertical Cuts
    int maxArea(int h, int w, ArrayList horizontalCuts,
                    ArrayList verticalCuts)
    {
 
        // Sort the two arrays
        Collections.sort(horizontalCuts);
        Collections.sort(verticalCuts);
 
        // Insert the right bound h and w
        // in their respective vectors
        horizontalCuts.add(h);
        verticalCuts.add(w);
 
        int maxHorizontal = 0;
        int maxVertical = 0;
 
        // Find the maximum Horizontal Length possible
        for (int i = 1; i < horizontalCuts.size(); i++) {
            int diff
                    = horizontalCuts.get(i) - horizontalCuts.get(i-1);
            maxHorizontal = Math.max(maxHorizontal, diff);
        }
 
        // Find the maximum vertical Length possible
        for (int i = 1; i < verticalCuts.size(); i++) {
            int diff
                    = verticalCuts.get(i) - verticalCuts.get(i - 1);
            maxVertical = Math.max(maxVertical, diff);
        }
 
        // Return the maximum area of rectangle
        return (int)((long)maxHorizontal * maxVertical
                % mod);
        }
 
    // Driver Code
    public static void main(String[] args)
    {
       
        // Class Call
        GFG ob = new GFG();
 
        // Given Input
        ArrayList hc = new ArrayList<>();
        hc.add(2);
        hc.add(5);
        ArrayList vc = new ArrayList<>();
        vc.add(1);
        vc.add(3);
 
        int h = 6, v = 4;
       
        // Function Call
        System.out.println(ob.maxArea(6, 4, hc, vc));
    }
}
 
//This code is contributed by hritikrommie.

Python3

# python 3 Program for the above approach
mod = 1000000007
 
# Returns the maximum area of rectangle
# after Horizontal and Vertical Cuts
def maxArea(h, w, horizontalCuts,
            verticalCuts):
 
    # Sort the two arrays
    horizontalCuts.sort()
    verticalCuts.sort()
 
    # Insert the right bound h and w
    # in their respective vectors
    horizontalCuts.append(h)
    verticalCuts.append(w)
 
    maxHorizontal = 0
    maxVertical = 0
 
    # Find the maximum Horizontal Length possible
    for i in range(1, len(horizontalCuts)):
 
        diff = horizontalCuts[i] - horizontalCuts[i - 1]
        maxHorizontal = max(maxHorizontal, diff)
 
    # Find the maximum vertical Length possible
    for i in range(1,
                   len(verticalCuts)):
        diff = verticalCuts[i] - verticalCuts[i - 1]
        maxVertical = max(maxVertical, diff)
 
    # Return the maximum area of rectangle
    return (int)(maxHorizontal * maxVertical
                 % mod)
 
 
# Driver Code
if __name__ == "__main__":
 
    # Given Input
    hc = [2, 5]
    vc = [1, 3]
    h = 6
    v = 4
     
    # Function Call
    print(maxArea(6, 4, hc, vc))
 
    # This code is contributed by ukasp.

C#

// C# Program for the above approach
using System;
using System.Collections;
using System.Collections.Generic;
class GFG
{
    static int mod = 1000000007;
     
    // Returns the maximum area of rectangle
    // after Horizontal and Vertical Cuts
    static int maxArea(int h, int w, List horizontalCuts, List verticalCuts)
    {
        // Sort the two arrays
        horizontalCuts.Sort();
        verticalCuts.Sort();
      
        // Insert the right bound h and w
        // in their respective vectors
        horizontalCuts.Add(h);
        verticalCuts.Add(w);
      
        int maxHorizontal = 0;
        int maxVertical = 0;
      
        // Find the maximum Horizontal Length possible
        for(int i = 1; i < horizontalCuts.Count; i++)
        {
      
            int diff = horizontalCuts[i] - horizontalCuts[i - 1];
            maxHorizontal = Math.Max(maxHorizontal, diff);
        }
      
        // Find the maximum vertical Length possible
        for(int i = 1; i < verticalCuts.Count; i++)
        {
            int diff = verticalCuts[i] - verticalCuts[i - 1];
            maxVertical = Math.Max(maxVertical, diff);
        }
      
        // Return the maximum area of rectangle
        return (int)(maxHorizontal * maxVertical % mod);
    }
     
  static void Main ()
  {
    // Given Input
    List hc = new List(new int[]{ 2, 5 });
    List vc = new List(new int[]{ 1, 3 });
     
    // Function Call
    Console.WriteLine(maxArea(6, 4, hc, vc));
  }
}
 
// This code is contributed by suresh07.

Javascript

        // JavaScript program for the above approach
 
        const mod = 1e9 + 7;
 
        class Solution {
 
            // Returns the maximum area of rectangle
            // after Horizontal and Vertical Cuts
            maxArea(h, w, horizontalCuts, verticalCuts) {
 
                // Sort the two arrays
                horizontalCuts.sort(function (a, b) { return a - b; })
                verticalCuts.sort(function (a, b) { return a - b; })
 
                // Insert the right bound h and w
                // in their respective vectors
                horizontalCuts.push(h);
                verticalCuts.push(w);
 
                let maxHorizontal = 0;
                let maxVertical = 0;
 
                // Find the maximum Horizontal Length possible
                for (let i = 1; i < horizontalCuts.length; i++) {
                    let diff
                        = horizontalCuts[i] - horizontalCuts[i - 1];
                    maxHorizontal = Math.max(maxHorizontal, diff);
                }
 
                // Find the maximum vertical Length possible
                for (let i = 1; i < verticalCuts.length; i++) {
                    let diff
                        = verticalCuts[i] - verticalCuts[i - 1];
                    maxVertical = Math.max(maxVertical, diff);
                }
 
                // Return the maximum area of rectangle
                return parseInt(maxHorizontal * maxVertical
                    % mod);
            }
        }
 
        // Driver Code
 
        // Class Call
        let ob = new Solution();
 
        // Given Input
        let hc = [2, 5], vc = [1, 3];
        let h = 6, v = 4;
        // Function Call
        document.write(ob.maxArea(6, 4, hc, vc));
 
    // This code is contributed by Potta Lokesh
 
    

输出

6

时间复杂度： O(NlogN)
辅助空间： O(1)

如果您希望与专家一起参加现场课程，请参阅DSA 现场工作专业课程和学生竞争性编程现场课程。