表达式树的评估

给定一个简单的表达式树，由基本的二元运算符组成，即 + 、 – 、* 和 / 以及一些整数，计算表达式树。

例子：

Input: Root node of the below tree

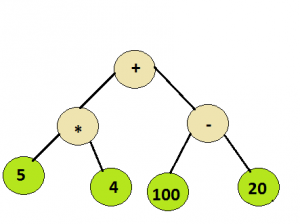
Output:100

Input: Root node of the below tree

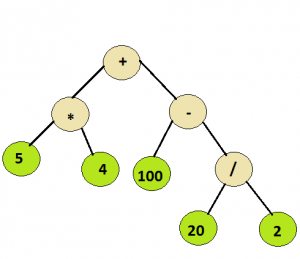
Output: 110

方法：解决此问题的方法基于以下观察：

As all the operators in the tree are binary, hence each node will have either 0 or 2 children. As it can be inferred from the examples above, all the integer values would appear at the leaf nodes, while the interior nodes represent the operators.

Therefore we can do inorder traversal of the binary tree and evaluate the expression as we move ahead.

为了评估语法树，可以遵循递归方法。

Algorithm:

• Let t be the syntax tree
• If  t is not null then      
  • If t.info is operand then  
    • Return  t.info
  • Else
    • A = solve(t.left)
    • B = solve(t.right)
    • return A operator B, where operator is the info contained in t

下面是上述方法的实现：

C++

// C++ program to evaluate an expression tree
#include 
using namespace std;
 
// Class to represent the nodes of syntax tree
class node
{
public:
    string info;
    node *left = NULL, *right = NULL;
    node(string x)
    {
        info = x;
    }
};
 
// Utility function to return the integer value
// of a given string
int toInt(string s)
{
    int num = 0;
         
    // Check if the integral value is
    // negative or not
    // If it is not negative, generate the number
    // normally
    if(s[0]!='-')
        for (int i=0; ileft && !root->right)
        return toInt(root->info);
 
    // Evaluate left subtree
    int l_val = eval(root->left);
 
    // Evaluate right subtree
    int r_val = eval(root->right);
 
    // Check which operator to apply
    if (root->info=="+")
        return l_val+r_val;
 
    if (root->info=="-")
        return l_val-r_val;
 
    if (root->info=="*")
        return l_val*r_val;
 
    return l_val/r_val;
}
 
//driver function to check the above program
int main()
{
    // create a syntax tree
    node *root = new node("+");
    root->left = new node("*");
    root->left->left = new node("5");
    root->left->right = new node("-4");
    root->right = new node("-");
    root->right->left = new node("100");
    root->right->right = new node("20");
    cout << eval(root) << endl;
 
    delete(root);
 
    root = new node("+");
    root->left = new node("*");
    root->left->left = new node("5");
    root->left->right = new node("4");
    root->right = new node("-");
    root->right->left = new node("100");
    root->right->right = new node("/");
    root->right->right->left = new node("20");
    root->right->right->right = new node("2");
 
    cout << eval(root);
    return 0;
}

Java

// Java program to evaluate expression tree
import java.lang.*;
 
class GFG{
     
Node root;
 
// Class to represent the nodes of syntax tree
public static class Node
{
    String data;
    Node left, right;
 
    Node(String d)
    {
        data = d;
        left = null;
        right = null;
    }
}
 
private static int toInt(String s)
{
    int num = 0;
 
    // Check if the integral value is
    // negative or not
    // If it is not negative, generate
    // the number normally
    if (s.charAt(0) != '-')
        for(int i = 0; i < s.length(); i++)
            num = num * 10 + ((int)s.charAt(i) - 48);
             
    // If it is negative, calculate the +ve number
    // first ignoring the sign and invert the
    // sign at the end
    else
    {
        for(int i = 1; i < s.length(); i++)
          num = num * 10 + ((int)(s.charAt(i)) - 48);
        num = num * -1;
    }
    return num;
}
 
// This function receives a node of the syntax
// tree and recursively evaluate it
public static int evalTree(Node root)
{
     
    // Empty tree
    if (root == null)
        return 0;
 
    // Leaf node i.e, an integer
    if (root.left == null && root.right == null)
        return toInt(root.data);
 
    // Evaluate left subtree
    int leftEval = evalTree(root.left);
 
    // Evaluate right subtree
    int rightEval = evalTree(root.right);
 
    // Check which operator to apply
    if (root.data.equals("+"))
        return leftEval + rightEval;
 
    if (root.data.equals("-"))
        return leftEval - rightEval;
 
    if (root.data.equals("*"))
        return leftEval * rightEval;
 
    return leftEval / rightEval;
}
 
// Driver code
public static void main(String[] args)
{
     
    // Creating a sample tree
    Node root = new Node("+");
    root.left = new Node("*");
    root.left.left = new Node("5");
    root.left.right = new Node("-4");
    root.right = new Node("-");
    root.right.left = new Node("100");
    root.right.right = new Node("20");
    System.out.println(evalTree(root));
 
    root = null;
 
    // Creating a sample tree
    root = new Node("+");
    root.left = new Node("*");
    root.left.left = new Node("5");
    root.left.right = new Node("4");
    root.right = new Node("-");
    root.right.left = new Node("100");
    root.right.right = new Node("/");
    root.right.right.left = new Node("20");
    root.right.right.right = new Node("2");
    System.out.println(evalTree(root));
}
}
 
// This code is contributed by Ankit Gupta

Python3

# Python program to evaluate expression tree
 
# Class to represent the nodes of syntax tree
 
 
class node:
    def __init__(self, value):
        self.left = None
        self.data = value
        self.right = None
 
# This function receives a node of the syntax tree
# and recursively evaluate it
 
 
def evaluateExpressionTree(root):
 
    # empty tree
    if root is None:
        return 0
 
    # leaf node
    if root.left is None and root.right is None:
        return int(root.data)
 
    # evaluate left tree
    left_sum = evaluateExpressionTree(root.left)
 
    # evaluate right tree
    right_sum = evaluateExpressionTree(root.right)
 
    # check which operation to apply
    if root.data == '+':
        return left_sum + right_sum
 
    elif root.data == '-':
        return left_sum - right_sum
 
    elif root.data == '*':
        return left_sum * right_sum
 
    else:
        return left_sum // right_sum
 
 
# Driver function to test above problem
if __name__ == '__main__':
 
    # creating a sample tree
    root = node('+')
    root.left = node('*')
    root.left.left = node('5')
    root.left.right = node('-50')
    root.right = node('-')
    root.right.left = node('100')
    root.right.right = node('20')
    print (evaluateExpressionTree(root))
 
    root = None
 
    # creating a sample tree
    root = node('+')
    root.left = node('*')
    root.left.left = node('5')
    root.left.right = node('4')
    root.right = node('-')
    root.right.left = node('100')
    root.right.right = node('/')
    root.right.right.left = node('20')
    root.right.right.right = node('2')
    print (evaluateExpressionTree(root))
 
# This code is contributed by Harshit Sidhwa

C#

// C# program to evaluate expression tree
using System;
 
public class GFG
{
 
    // Class to represent the nodes of syntax tree
    public class Node {
        public
       String data;
              public
       Node left, right;
 
        public Node(String d) {
            data = d;
            left = null;
            right = null;
        }
    }
 
    private static int toInt(String s) {
        int num = 0;
 
        // Check if the integral value is
        // negative or not
        // If it is not negative, generate
        // the number normally
        if (s[0] != '-')
            for (int i = 0; i < s.Length; i++)
                num = num * 10 + ((int) s[i] - 48);
 
        // If it is negative, calculate the +ve number
        // first ignoring the sign and invert the
        // sign at the end
        else {
          for (int i = 1; i < s.Length; i++)
            num = num * 10 + ((int) (s[i]) - 48);
          num = num * -1;
        }
        return num;
    }
 
    // This function receives a node of the syntax
    // tree and recursively evaluate it
    public static int evalTree(Node root) {
 
        // Empty tree
        if (root == null)
            return 0;
 
        // Leaf node i.e, an integer
        if (root.left == null && root.right == null)
            return toInt(root.data);
 
        // Evaluate left subtree
        int leftEval = evalTree(root.left);
 
        // Evaluate right subtree
        int rightEval = evalTree(root.right);
 
        // Check which operator to apply
        if (root.data.Equals("+"))
            return leftEval + rightEval;
 
        if (root.data.Equals("-"))
            return leftEval - rightEval;
 
        if (root.data.Equals("*"))
            return leftEval * rightEval;
 
        return leftEval / rightEval;
    }
 
    // Driver code
    public static void Main(String[] args) {
 
        // Creating a sample tree
        Node root = new Node("+");
        root.left = new Node("*");
        root.left.left = new Node("5");
        root.left.right = new Node("-4");
        root.right = new Node("-");
        root.right.left = new Node("100");
        root.right.right = new Node("20");
        Console.WriteLine(evalTree(root));
 
        root = null;
 
        // Creating a sample tree
        root = new Node("+");
        root.left = new Node("*");
        root.left.left = new Node("5");
        root.left.right = new Node("4");
        root.right = new Node("-");
        root.right.left = new Node("100");
        root.right.right = new Node("/");
        root.right.right.left = new Node("20");
        root.right.right.right = new Node("2");
        Console.WriteLine(evalTree(root));
    }
}
 
// This code is contributed by umadevi9616

Javascript

// javascript program to evaluate expression tree
    var root;
 
    // Class to represent the nodes of syntax tree
     class Node {
        constructor(val) {
            this.data = val;
            this.left = null;
            this.right = null;
        }
    }
 
     function toInt( s) {
        var num = 0;
  
        // Check if the integral value is
        // negative or not
        // If it is not negative, generate
        // the number normally
        if (s.charAt(0) != '-')
            for (i = 0; i < s.length; i++)
                num = num * 10 + ( s.charCodeAt(i) - 48);
 
        // If it is negative, calculate the +ve number
        // first ignoring the sign and invert the
        // sign at the end
        else {
          for (i = 1; i < s.length; i++)
              num = num * 10 + (s.charCodeAt(i) - 48);
          num = num * -1;
        }
        return num;
    }
 
    // This function receives a node of the syntax
    // tree and recursively evaluate it
    function evalTree(root) {
 
        // Empty tree
        if (root == null)
            return 0;
 
        // Leaf node i.e, an integer
        if (root.left == null && root.right == null)
            return toInt(root.data);
 
        // Evaluate left subtree
        var leftEval = evalTree(root.left);
 
        // Evaluate right subtree
        var rightEval = evalTree(root.right);
 
        // Check which operator to apply
        if (root.data === ("+"))
            return leftEval + rightEval;
 
        if (root.data === ("-"))
            return leftEval - rightEval;
 
        if (root.data === ("*"))
            return leftEval * rightEval;
 
        return leftEval / rightEval;
    }
 
    // Driver code
     
 
        // Creating a sample tree
        var root = new Node("+");
        root.left = new Node("*");
        root.left.left = new Node("5");
        root.left.right = new Node("-4");
        root.right = new Node("-");
        root.right.left = new Node("100");
        root.right.right = new Node("20");
        document.write(evalTree(root));
 
        root = null;
 
        // Creating a sample tree
        root = new Node("+");
        root.left = new Node("*");
        root.left.left = new Node("5");
        root.left.right = new Node("4");
        root.right = new Node("-");
        root.right.left = new Node("100");
        root.right.right = new Node("/");
        root.right.right.left = new Node("20");
        root.right.right.right = new Node("2");
        document.write("<br/>"+evalTree(root));
 
// This code is contributed by gauravrajput1

输出

60
110

时间复杂度： O(n)，因为每个节点都被访问一次。