直接替代的限制

极限是微积分的基石。它们是我们到达特定点时函数似乎正在采取的值。它们有助于计算函数的变化率。导数的概念是有限制的。它们还帮助我们定义连续性和可区分性的概念。因此，了解他们的直觉和不同方法来计算不同类别功能的限制变得至关重要。

限制

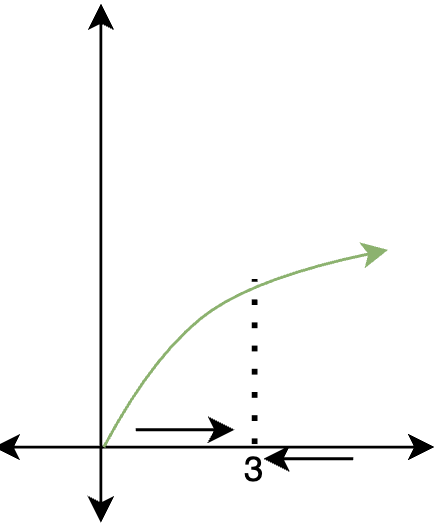
从几何上讲，函数在特定点的极限可以很容易地通过它的图来估计。例如，在下面给出的图表中，x = 3。函数似乎取值 1。在这种情况下，我们从哪一侧接近并不重要——左侧和右侧.

对于函数f(x)，x = a 处的极限表示为，

$\lim_{x \to a} f(x)$

使用直接替换的限制

计算极限的替换规则是一种找到极限的方法，只需将 x 的值替换为我们要计算极限的点即可。考虑一个函数f(x)，目标是找到函数在 x = a 处的极限。在这种方法中，在函数f(x) 的表达式中，x 被简单地替换为“a”。

$\lim_{x \to a}f(x) = f(a)$

让我们通过一个例子来看看这个方法，

考虑到 f(x) = x ² ，

$\lim_{x \to 1}f(x) = f(1)$

⇒ $\lim_{x \to 1}x^2 = f(1)$

⇒ $1^2 = f(1)$

⇒ $1 = f(1)$

通常可以用这个规则计算函数的极限，正式说明这一点。

If f(x) is an expression built from the polynomials, roots, absolute values, exponential, logarithms, trigonometric functions, and/or inverse trigonometric functions by using composition of functions and the operations such as x, +, -, / then for any a for which f(a) is defined, 

$\lim_{x \to a}f(x) = f(a)$

直接替换的未定义限制

此方法无法计算某些限制。例如，考虑一个函数f(x) =  $\frac{x}{ln(x)}$ ，计算此函数在 x = 1 处的极限。

$\lim_{x \to 1}f(x)$

⇒ $\lim_{x \to 1}\frac{x}{ln(x)}$

⇒ $\frac{\lim_{x \to 1} x}{\lim_{x \to 1} ln(x)}$

⇒ $\frac{1}{0}$

此限制未定义。在这种情况下，直接替换法。

函数的极限

有时可以使用直接替换来计算涉及三角函数的函数的极限。例如，假设我们有一个函数f(x)，我们想计算该函数在 x = 0 处的极限。让我们用一个例子来看看。

示例：计算$\lim_{x \to 0}f(x)$

f(x) = sin(x) + sin(x)cos(x)

解决方案：

$\lim_{x \to 0}f(x)$

⇒ $\lim_{x \to 0}(sin(x) + sin(x)cos(x))$

⇒$\lim_{x \to 0}(sin(x)(1 + cos(x))$

⇒$\lim_{x \to 0}sin(x) \times \lim_{x \to 0}(1 + cos(x))$

⇒0 × (1 + 1)

⇒ 0 

分段函数的极限

在使用分段函数时，替换规则通常在函数定义发生变化的地方不起作用。它以稍微修改的方式用于这些功能。让我们解决一个示例问题以进一步理解这一点，

示例：计算的值$\lim_{x \to 1}f(x)$ .

$f(x)= \begin{cases} x^2-1,& \text{if } x\geq 1\\ x, & \text{otherwise} \end{cases}$

解决方案：

At x = 1, the function definition is changing. So it is not advised to directly apply the rule. In such functions, one should look for the limit from both sides. 

Left-hand Side Limit 

$\lim_{x \to 1^{-}}f(x)$

⇒$\lim_{x \to 1^-} x$

⇒1

Right-hand Side Limit 

$\lim_{x \to 1^{+}}f(x)$

⇒$\lim_{x \to 1^+} x^2-1$

⇒0

In this case, limits from both sides are different. 

让我们看看这些概念上的一些问题

示例问题

问题1：计算$\lim_{x \to 0}f(x)$

f(x) = x ² + x + 1

解决方案：

$\lim_{x \to 0}f(x)$

⇒ $\lim_{x \to 0}(x^2 + x + 1)$

⇒$(0^2 + 0 + 1)$

⇒ 1

问题2：计算$\lim_{x \to 1}f(x)$

f(x) = $\frac{x^2 + x + 1}{x + 1}$

解决方案：

$\lim_{x \to 1}\frac{x^2 + x + 1}{x + 1}$

⇒ \$\frac{\lim_{x \to 1}x^2 + x + 1}{\lim_{x \to 1}x + 1}$

⇒$\frac{1^2 + 1 + 1}{1 + 1}$

⇒ $\frac{3}{2}$

问题3：计算值$\lim_{x \to 1}f(x)$ .

$f(x)= \begin{cases} sin(x),& \text{if } x\geq 1\\ cos(x), & \text{otherwise} \end{cases}$

解决方案：

At x = 1, the function definition is changing. So it is not advised to directly apply the rule. In such functions, one should look for the limit from both sides. 

Left-hand Side Limit 

$\lim_{x \to 1^{-}}cos(x)$

⇒$cos(1)$

Right-hand Side Limit 

$\lim_{x \to 1^{+}}sin(x)$

⇒$sin(1)$

In this case, limits from both sides are different. 

问题4：计算$\lim_{x \to 1}f(x)$

f(x) = $\frac{e^x + x + 1}{log(x) + 1}$

解决方案：

$\lim_{x \to 1}\frac{e^x + x + 1}{log(x) + 1}$

⇒ \$\frac{\lim_{x \to 1}e^x + x + 1}{\lim_{x \to 1}log(x) + 1}$

⇒$\frac{e^1 + 1 + 1}{log(1) + 1}$

⇒ $\frac{e^1 + 2}{0 + 1}$

⇒ e + 2

问题 5：计算$\lim_{x \to 1}f(x)$

f(x) = $\frac{e^{sin(x)} + tan(x) + 1}{log(x) + 1}$

解决方案：

$\lim_{x \to 1}\frac{e^{sin(x)} + tan(x) + 1}{log(x) + 1}$

⇒ \$\frac{\lim_{x \to 1}e^{sin(x)} + tan(x) + 1}{\lim_{x \to 1}log(x) + 1}$

⇒$\frac{e^{sin(1)} + 1 + 1}{log(1) + 1}$

⇒ $\frac{e^{sin(1)} + 2}{0 + 1}$

⇒ e^sin(1) + 2

问题 6：计算$\lim_{x \to 0}f(x)$使用替换规则。

f(x) = $\frac{sin^2(x) + cos(x) - 1}{1 - cos(x)}$

解决方案：

$\lim_{x \to 0}\frac{sin^2(x) + cos(x) - 1}{1 - cos(x)}$

⇒ \$\frac{\lim_{x \to 0}sin^2(x) + cos(x) - 1}{\lim_{x \to 0}1 - cos(x)}$

⇒$\frac{sin^2(0) + cos(0) - 1}{1 - cos(0)}$

This is 0/0 form, so the limit is undefined. The substitution rule cannot be used here. 

问题7：计算值$\lim_{x \to 1}f(x)$ .

$f(x)= \begin{cases} e^x,& \text{if } x\geq 1\\ e^{-x}, & \text{otherwise} \end{cases}$

解决方案：

At x = 1, the function definition is changing. So it is not advised to directly apply the rule. In such functions, one should look for the limit from both sides. 

Left-hand Side Limit 

$\lim_{x \to 1^{-}}e^{-x}$

⇒$\frac{1}{e}$

Right-hand Side Limit 

$\lim_{x \to 1^{+}}e^{x}$

⇒$e$

In this case also, limits from both sides are different.