使用MATLAB的根轨迹

在控制系统中，根源分析是一种图形化策略，用于查看系统基础如何随特定系统边界的变化而变化，通常是在反馈系统内部进行附加。

控制系统中根源的目的如下：

1. 寻找稳定性
2. 检查点是否在根源上
3. 查找系统增益，即“ k”或系统参数

根轨迹的构造规则：

规则1：在实轴上将存在一个点，如果该点右侧的极点和零点之和必须为奇数，则根轨迹分支。

规则2：渐近线：它们是根轨迹分支，从实轴开始并接近无穷大。

渐近线数“ N = P – Z”

这里“ P”是极数，“ Z”是零数

规则3：渐近线的角度

规则4：质心：渐近线在实轴上的交点称为质心

规则5：断点(BP)：有两种类型

1. 突破点(BAP)
2. 突破点(BIP)

规则6：具有假想轴的根轨迹交点(IP)。

规则7：

a)偏离角：计算为复共轭极或虚极

b)到达角：计算复共轭零或虚零

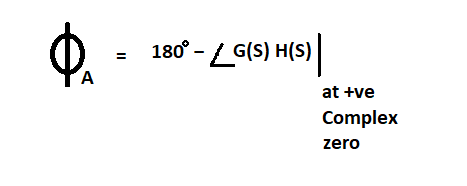
代码 ：

% Row of 1×2
NUM = [1 10]; 
  
% Row of 1×4
DEN = [1 6 8 0]; 
  
% Row of 1×2
poly1 = [1 2]; 
  
% Row of 1×2
poly2 = [1 4]; 
  
% convolves vectors poly1 and poly2, 
% multiplying the polynomials whose coefficients 
% are the elements of poly1 and poly2
poly = conv(poly1, poly2);  
  
% returns the roots of the polynomial 
% represented by DEN as a column vector
roots(DEN); 
  
% Continuous time transfer function
sys = tf(NUM, DEN);
  
% GUI for per-forming Root Locus analysis
rltool(sys); 

输出 ：