📌  相关文章
📜  液体溶液的蒸气压

📅  最后修改于: 2022-05-13 01:57:12.371000             🧑  作者: Mango

液体溶液的蒸气压

当液体在密闭容器中蒸发时，一些液体会蒸发，而蒸汽会充满剩余空间。由于蒸气离开容器，它们在液体表面上方以蒸气状态冷凝。液体由于汽化而转化为蒸气，并且液体的体积减小。随着蒸发的进行，气相中气态分子的数量不断增加。这些分子在密闭空间中随意游荡，有的与液体表面碰撞而凝结。冷凝过程在与蒸发相反的方向进行。结果，蒸发和冷凝同时发生。

液体溶液的蒸气压

在给定温度下，液体/溶液的蒸气压是蒸气与液体/溶液平衡时施加的压力。

蒸气压α逸出倾向

液体溶液的蒸气压和拉乌尔定律（Raoult's law for volatile solutes）

根据拉乌尔定律，挥发性液体溶液中各组分的蒸气分压与其摩尔分数精确成正比。

考虑一个包含两种挥发性成分 1 和 2 的溶液，每种成分的摩尔分数分别为 x 1和 x 2 。假设它们在一定温度下的蒸气分压分别为p 1和p 2 ，它们的纯态蒸气压分别为p 1 0和p 2 0 。因此，对于组件 1，根据拉乌尔定律，

p 1 α x 1

和

p 1 = p 1 0 x 1

同样，对于组件 2

p 2 = p 2 0 x 2

根据道尔顿分压定律，容器中溶液相的总压力 (p total ) 等于溶液各组分的分压之和，如下所示：

p总计= p 1 + p 2

代入 p 1和 p 2的值，我们得到

p总计= x 1 p 1 0 + x 2 p 2 0

= ( 1 – x 2 )p 1 0 + x 2 p 2 0

= p 1 0 + ( p 2 0 – p 1 0 )x 2

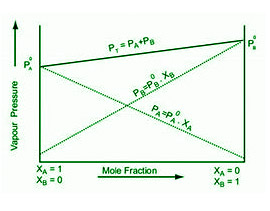
在恒定温度下，理想溶液的蒸气压和摩尔分数图。组分的分压由虚线 I 和 II 表示。该图清楚地表明 p 1和 p 2分别与 x 1和 x 2成比例。总蒸气压由图中的线 III 表示。

  • 气相中的摩尔分数

使用道尔顿分压定律，如果 y 1和 y 2是气相中组分 1 和 2 的摩尔分数，则：

p 1 = y 1 p总计

p 2 = y 2 p总计

一般来说，

p i = y i p总计

  • 蒸气压与液-液溶液关系的结论

从关系可以得出以下结论。任何一种组分 A 或 B 的摩尔分数决定了总蒸气压。该等式也可以写成，例如，

P总= P B 0 + (P A 0 – P B 0 ) X A

以下任何成分都可以绘制为总压力和摩尔分数之间的线性图。根据组分 A 和 B 的纯蒸气压，总蒸气压相对于组分 A 的摩尔分数升高或降低。

如果组分为蒸气形式，则可以确定总蒸气压。

P A = y A P总计

P B = y B P总计

气相中组分A和B的摩尔分数分别为y A和y B 。

  • 作为亨利定律的特例，拉乌尔定律

二元液-液溶液中的一种成分具有足够的挥发性，它以气体的形式存在，其溶解度受亨利定律的限制。根据亨利定律，气相中气体的分压与溶液中气体的摩尔分数成正比，表示为P = K H X

溶液的分压为P，溶剂的摩尔分数为X。比例常数（亨利常数）用K H表示。

拉乌尔定律定义如下：

P = P 0 X

溶液的分压为P，溶剂的摩尔分数为X。P 0表示纯溶剂的蒸气压。比较亨利定律和拉乌尔定律的方程，我们得到：

K H = P 0

因此，拉乌尔定律是亨利定律的一个子集。

液体中固体溶液的蒸气压和拉乌尔定律

当将非挥发性溶质添加到溶剂中制成溶液时，从表面逸出的溶剂分子数量减少，从而降低了蒸气压。溶液中存在的非挥发性溶质的量，无论其组成如何，都会影响溶剂蒸气压的降低。在其最一般的形式中，拉乌尔定律指出，溶液中每种挥发性成分的蒸气分压与其每种溶液的摩尔分数成正比。

我们将溶剂 1 和溶质 2 称为二元溶液。当溶质不挥发时，只有溶剂分子存在于气相中，它们对蒸气压有贡献。令 p 1表示溶剂的蒸气压， x 1表示其摩尔分数， p 1 0表示纯态的蒸气压。然后，根据拉乌尔定律，

p 1 α x 1

p 1 = x 1 p 1 0 = p总计

理想和非理想的解决方案

理想的解决方案：理想的解决方案是每个组件在所有温度和浓度情况下都遵循拉乌尔规则。

理想解决方案的性质-

  • ΔH混合= 0
  • ΔV混合= 0
  • A - A 和 B - B 之间的分子间吸引力几乎是 A - B 之间的吸引力。

例如苯和甲苯的溶液，正己烷和正庚烷的溶液。

非理想溶液：术语“非理想溶液”是指在整个浓度范围内不遵守拉乌尔定律的溶液。

  • 显示正偏离拉乌尔定律的解决方案-
    • 溶剂-溶质 (A – B) 类型的力弱于溶质-溶质 (B – B) 和溶剂-溶剂 (A – A) 力。
    • 蒸气压比法律预测的要高。
    • ΔH混合> 0
    • ΔV混合> 0

例如：乙醇和丙酮、二硫化碳和丙酮。

  • 显示负偏离拉乌尔定律的解决方案-
    • 溶剂-溶质 (A – B) 类型的力比溶质-溶质 (B – B) 和溶剂-溶剂 (A – A) 力强。
    • 蒸气压低于法律预测。
    • ΔH混合< 0
    • ΔV混合< 0

例如：苯酚和苯胺、氯仿和丙酮等。

示例问题

问题 1：温度对溶液的蒸气压有什么影响？

回答：

问题 2：纯氯仿 CHCl 3和二氯甲烷 CH 2 Cl 2的蒸气压分别为 200 mm Hg 和 415 mm Hg。在常温 298 K 下，含有 30 g CHCl 3和 42 g CH 2 Cl 2的溶液的总蒸气压是多少？在气相中，确定两种组分的摩尔分数。

回答：

问题 3：不挥发溶质的类型会影响溶液的蒸气压吗？

回答：

问题 4：如何计算液体的蒸气压？

回答：

问题5：哪一个的蒸气压最高？

回答：