菱形公式的面积

面积、体积、长度等概念。任何二维或三维图形/形状的测量都属于测量主题。测量是几何学的一个分支，它处理二维或三维图形的长度、周长、面积、体积等的测量。 2D形状包括圆形、三角形、正方形、矩形、菱形、梯形等。3D图形包括立方体、球体、圆柱体、长方体、金字塔等。这篇文章是关于寻找2D形状菱形的面积。

菱形面积

菱形有四个长度相等且全等的边。菱形的面积是菱形包围的空间量。菱形的面积可以通过三种方式计算。

• 方法一

当给定对角线的长度时，可以使用以下公式计算菱形的面积，

Area = (1/2) × length of diagonal₁ × length of diagonal₂

• 方法二

菱形的面积是使用底边和高度计算的，其中底边是任意边的长度，高度是从所选底边到对边的垂直距离。

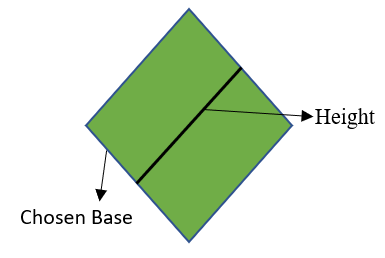
公式由下式给出：

Area of rhombus = length of base × height

• 方法3

当菱形任意边的长度与任意内角一起给出时，菱形的面积由以下公式计算，

Area of rhombus = length of a side² × sin(angle)

Note: The sine values for particular angles are,

	0°	30°	45°	60°	90°
Sinθ	0	1/2	1/√2	√3/2	1

示例问题

问题1：对角线长度为4cm、5cm的菱形的面积是多少？

解决方案：

Given lengths of diagonals

D₁ = 4cm

D₂ = 5cm

Using approach 1,

Area of rhombus = (1/2) × 4 × 5

= (1/2) × 20

= 10

So the area of rhombus is 10cm².

问题2：求对角线长度为7cm和7cm的菱形面积。

解决方案：

Given lengths of diagonals

D₁ = 7cm

D₂ = 7cm

Using approach 1,

Area of rhombus = (1/2) × 7 × 7

= (1/2) × 49

= 24.5

So the area of given rhombus is 24.5cm².

问题3：求一个菱形的面积，高4cm，底长6cm。

解决方案：

Given,

Base length = 6cm

height = 4cm

Area of rhombus = base × height

= 6 × 4

= 24cm²

So area of given rhombus is 24cm².

问题4：求菱形高6cm、底长8cm的面积。

解决方案：

Given,

Base length = 8cm

Height = 6cm

Using approach 2,

Area of rhombus = base × height

= 8 × 6

= 48cm²

So area of given rhombus is 24cm².

问题5：求菱形边长为5cm，内角之一为45°的菱形面积。

解决方案：

Given,

Side length = 5cm

Interior angle = 45°

Using Approach 3,

Area of rhombus = 5² × sin(45°)

= 25 × (1/√2)

= 17.68 cm²

So the area of given rhombus is 17.68cm²

问题6：求菱形的边长为4cm，内角为60°的区域。

解决方案：

Given,

Side length = 4cm

Interior angle = 60°

Using approach 3,

Area of rhombus = 4² × sin(60°)

= 16 × (1/(√3/2))

= 18.47 cm² (approximately)

So the area of given rhombus is 18.47cm²