懒惰的餐饮者问题

给定一个整数n，表示可以在一块薄煎饼上切的块数，求出可以制作n个切块的最大块数。
例子 ：

Input :  n = 1
Output : 2
With 1 cut we can divide the pancake in 2 pieces

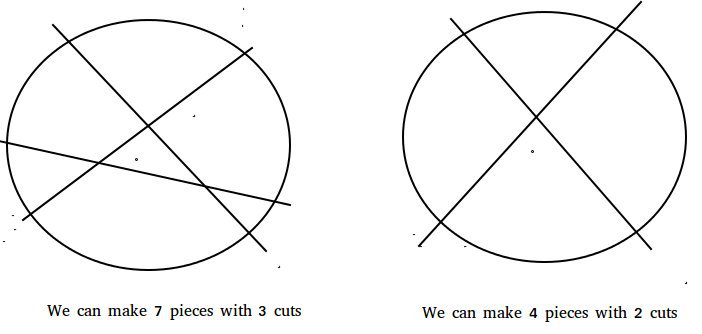
Input :  2
Output : 4
With 2 cuts we can divide the pancake in 4 pieces

Input : 3
Output : 7
We can divide the pancake in 7 parts with 3 cuts

Input : 50
Output : 1276

Let f(n) denote the maximum number of pieces
that can be obtained by making n cuts.
Trivially,
f(0) = 1                                 
As there'd be only 1 piece without any cut.

Similarly,
f(1) = 2

Proceeding in similar fashion we can deduce 
the recursive nature of the function.
The function can be represented recursively as :
f(n) = n + f(n-1)

Hence a simple solution based on the above 
formula can run in O(n). 

我们可以优化上面的公式。

We now know ,
f(n) = n + f(n-1) 

Expanding f(n-1) and so on we have ,
f(n) = n + n-1 + n-2 + ...... + 1 + f(0)

which gives,
f(n) = (n*(n+1))/2 + 1

因此，通过这种优化，我们可以回答O(1)中的所有查询。
下面是上述想法的实现：

C++

// A C++ program to find the solution to
// The Lazy Caterer's Problem
#include 
using namespace std;
 
// This function receives an integer n
// and returns the maximum number of
// pieces that can be made form pancake
// using n cuts
int findPieces(int n)
{
    // Use the formula
    return (n * ( n + 1)) / 2 + 1;
}
 
// Driver Code
int main()
{
    cout << findPieces(1) << endl;
    cout << findPieces(2) << endl;
    cout << findPieces(3) << endl;
    cout << findPieces(50) << endl;
    return 0;
}

Java

// Java program to find the solution to
// The Lazy Caterer's Problem
import java.io.*;
 
class GFG
{
    // This function returns the maximum
    // number of pieces that can be made
    //  form pancake using n cuts
    static int findPieces(int n)
    {
        // Use the formula
        return (n * (n + 1)) / 2 + 1;
    }
     
    // Driver program to test above function
    public static void main (String[] args)
    {
        System.out.println(findPieces(1));
        System.out.println(findPieces(2));
        System.out.println(findPieces(3));
        System.out.println(findPieces(50));
    }
}
 
// This code is contributed by Pramod Kumar

Python3

# A Python 3 program to
# find the solution to
# The Lazy Caterer's Problem
 
# This function receives an
# integer n and returns the
# maximum number of pieces
# that can be made form
# pancake using n cuts
def findPieces( n ):
 
    # Use the formula
    return (n * ( n + 1)) // 2 + 1
 
# Driver Code
print(findPieces(1))
print(findPieces(2))
print(findPieces(3))
print(findPieces(50))
 
# This code is contributed
# by ihritik

C#

// C# program to find the solution
// to The Lazy Caterer's Problem
using System;
 
class GFG
{
    // This function returns the maximum
    // number of pieces that can be made
    // form pancake using n cuts
    static int findPieces(int n)
    {
        // Use the formula
        return (n * (n + 1)) / 2 + 1;
    }
     
    // Driver code
    public static void Main ()
    {
        Console.WriteLine(findPieces(1));
        Console.WriteLine(findPieces(2));
        Console.WriteLine(findPieces(3));
        Console.Write(findPieces(50));
    }
}
 
// This code is contributed by Nitin Mittal.

Javascript

 
// Javascript program to find the solution to
// The Lazy Caterer's Problem
 
    // This function returns the maximum
    // number of pieces that can be made
    //  form pancake using n cuts
    function findPieces(n)
    {
        // Use the formula
        return (n * (n + 1)) / 2 + 1;
    }
   
 
// Driver Code
     
        document.write(findPieces(1) + "<br/>");
        document.write(findPieces(2) + "<br/>");
        document.write(findPieces(3) + "<br/>");
        document.write(findPieces(50));
         

输出 ：

2
4
7
1276