📌  相关文章
📜  惠更斯波浪理论

📅  最后修改于: 2022-05-13 01:57:36.920000             🧑  作者: Mango

惠更斯波浪理论

克里斯蒂安·惠更斯提出了惠更斯原理。 1678 年，他改变了我们对光及其特性的看法。您可能听说过光的直线理论，该理论指出光以直线传播。检查各种光学现象的最重要方法之一是惠更斯原理。原理是一种分析方法，可用于解决远场极限、近场衍射和反射中的波传播问题。

当你在房间里打开一扇窗户时，光线会通过它进入并在整个空间中传播。你知道为什么会这样吗？这是因为光具有波动字符，并在房间内向各个方向传播。让我们看一下惠更斯原理以更好地理解这一点。

惠更原理

然而，这种解释并不能解释为什么首先会发生折射。其次，它无法解释光如何沿其路径传递能量。惠更斯原理，也称为惠更斯-菲涅耳原理，强调波的传播行为如下：

  • 二次源产生的小波与原始源的小波相当。
  • 新的波前由任何给定时间前向小波上的公切线确定。
  • 球面小波的总和就是波前。

波前和波法线

考虑“空气”中的点光源“S”。该光源发出的光波向各个方向传播。如果“c”是空气中的光速，则每个波将在时间 t 内传播“ct”并到达半径为“ct”的球体表面。以源“S”为焦点。这种类型的表面被称为球面波表面。

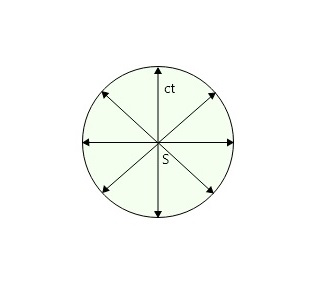
波面

波前是波同时到达的所有介质点的轨迹，因此所有点处于同一相位（见下图），例如，灯泡在有限距离处发出的光。当点光源位于其焦点时，平面波前从凸透镜中出现。此外，如果球面波前足够大，则表面的一小部分可以被认为是平面波前。例如，波前是由阳光引起的。线性源（例如狭缝）产生圆柱形波前。例如，光是由荧光管发出的。

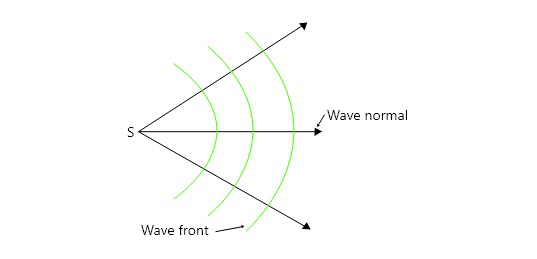
波前和波正常

波法线是在光传播方向上绘制到波前表面的垂线。换句话说，波前垂直于表面传输光能。

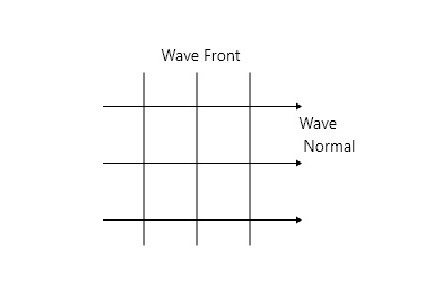
波正常

波前类型——波前分为三种类型，即

  1. 球面波前：考虑“S”点光源。 S产生的光波可以向任何方向移动。如果 c 是光速，那么每个波将在时间 t 后到达半径为 ct、中心为 S 的球体表面。这个球面被称为时间 t 的球面波前，它是从一个有限距离的点源推导出来的。例如，考虑一个灯泡在有限距离内发出的光。
  2. 平面波前：球面波前在距点源很远的距离处是如此巨大，以至于它的一小部分可以被认为是平面波前。它源自无限远的点源。例如，考虑由阳光引起的波前。
  3. 圆柱形波前：如果光源是线性的，即狭缝，那么我们得到一个圆柱形波前。它是从保持有限距离的线性源获得的。例如，光是由荧光管发出的。

惠更斯的球面波前构造

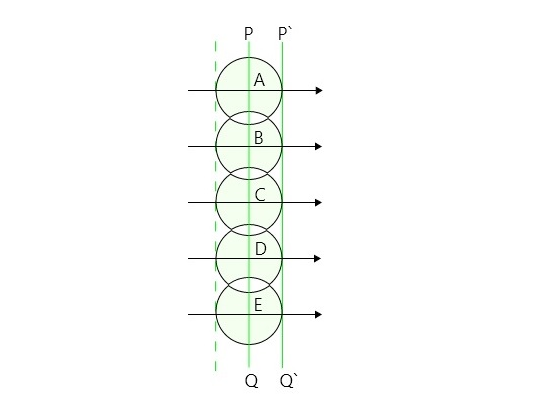
令 PQ 为在任何时刻由于点源 (S) 产生的球面波前的横截面。这可以称为初级波前。现在考虑 PQ 上的 A、B、C、D、E 点。它们充当二次源并根据惠更斯原理发出二次小波。

如果 c 是各向同性介质中的光速，则在时间 t 中，每个波将描述一个距离“ct”。以 A、B、C、D、E 为圆心，将跟踪每个半径 ct。每个圆圈将代表一个次级波前。

绘制到这些次级波阵面的公共切向表面（包络线）P'Q' 表示时间't' 之后波阵面的（新）位置。不存在向后移动的二次波。

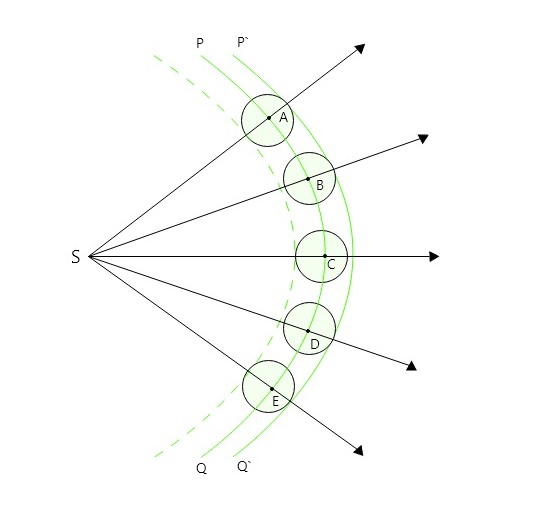
惠更斯球面波前

惠更斯的平面波前构造

令 PQ 是由于点源（S）在任何时刻和非常远的距离处垂直于纸面的平面波前，这可以称为主波前。现在考虑 PQ 上的 A、B、C、D 点。它们充当次级惠更斯源并根据惠更斯原理发出次级小波。

如果“c”是各向同性介质中的光速，那么在时间“t”中，每个波将描述一个距离“ct”。以 A、B、C、D 为中心，将跟踪每个半径为 'ct 的球体，每个球体将代表一个次级波前。绘制到这些次级波前的公共切面（包络线）即P'Q'代表时间't'之后波前的新位置。

向后移动的二次波不存在，因此用虚线表示。

惠更斯平面波前

惠更原理的优点

  • 它可以解释折射、干涉、反射、衍射等定律。
  • 波动理论预测，光在光密介质中的速度小于光稀介质中的光速，这一点由福柯通过实验证明。

惠更原理的缺点

  • 它无法解释光的直线传播。光的直线传播描述了光以直线传播。
  • 它无法解释光的偏振现象以及拉曼效应、康普顿效应和光电效应等现象。后来修改为光是横波。
  • 惠更斯假设存在一种假设的介质 [以太]。 Michelson 和 Morley 用他们基于光束干涉原理的设备进一步研究了假想的以太的性质。实验得出结论，当地球穿过它时，没有以太阻力。这个和其他实验证明以太不存在。

示例问题

问题1：陈述惠更斯光波理论的任何三个假设？

回答：

问题二：惠更斯光波理论的优缺点

回答：

问题 3：定义波前和波法线。

回答：

问题 4：给出平面波前和球面波前的区别？

回答：

问题 5：解释圆柱波前是如何产生的并举例说明？

回答：