拐点

考虑下图中给出的两个函数，而这两个函数看起来很相似。它们的不同之处在于它们的弯曲，注意其中一个看起来向上打开，另一个看起来向下闭合。这种趋势称为凹度，第一个图形向上凹，第二个图形向下凹。寻找这些趋势有助于我们生成函数的曲线。

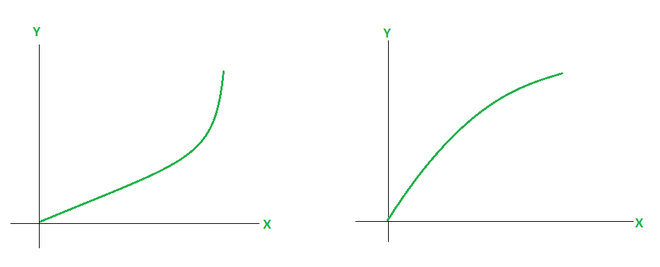
凹

如果该函数的图形位于在区间的任何点上绘制的任何切线之上，则称该函数在区间上是凹的。类似地，如果函数的图形位于在区间的任何点上绘制的任何切线下方，则称该函数是向下凹的。直观地说，凹度与增长率有关。众所周知，导数为我们提供了变化率，这意味着它们告诉我们函数是增加还是减少。凹度与变化率的变化率有关，即必然与双导有关。

在上图中，第一个函数以递增速率增加，而第二个函数以递减速率递增。从第一个函数中的事实也可以看出，切线变得越来越陡峭，反之亦然，对于第二个函数。下图显示了类似的场景，但具有递减函数。

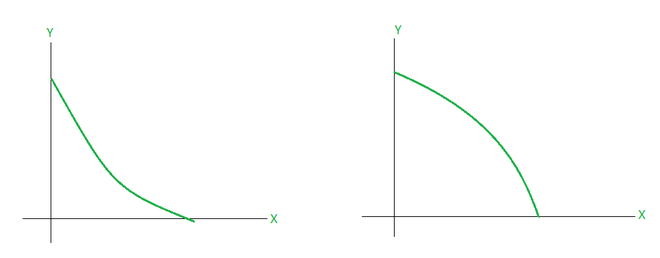
为了总结这些观察结果，下图显示了所有这些不同情况的综合比较。

为了形式化上述观察，

Concavity Test:

Consider a function f(x) which is differentiable and has derivatives and double derivatives as f’ and f” on the interval I. 

1. f(x) is considered concave up if f”(x) > 0 for all the points in the interval I.
2. (x) is considered concave down if f”(x) < 0 for all the points in the interval I.

拐点

有时在函数轨迹中，函数从向上凹到向下凹，反之亦然。现在，当函数经历这样的变化时，会有一个特定的点作为边界。这意味着如果在此点之前函数是凹的，那么在此点之后函数将与此相反。这个点称为拐点。该图显示了这一点。

从几何上讲，此时绘制的切线既不在图形上方，也不在图形下方。切线在这一点与函数的图形相交。

Point of inflection is the point where function goes from being concave upwards to concave downwards and vice-versa. Let’s say f(x) is the function which has a point x = c as it’s inflection point. Then, 

f”(c) = 0 or f”(c) does not exist. 

请注意，当 f”(c) = 0 时，这意味着 x = c 基本上是 f'(x) = 0 的临界点。因此，在这种情况下，可能存在三种情况：

1. 情况（i）： f”（x）的符号在拐点处由正变为负。这意味着函数从向上凹变为向下凹。
2. 情况（ii）： f”（x）的符号在拐点处由负变为正。这意味着函数从向下凹变为向上凹。
3. 情况（iii）：符号不变，这意味着在整个函数中二阶导数已经为零。在这种情况下，拐点将不存在。

这三种情况表明，如果某个点的二阶导数为零，则该点不一定是拐点。

Inflection Point Test

Consider a function f(x), to determine the inflection points. 

1. Find f”(x).
2. Solve the equation f”(x) = 0 and find all the critical points of f'(x).
3. Use the number line to see at which points the double derivative changes sign.

让我们看看这些概念的一些示例问题。

示例问题

问题1：找出给定函数在区间[0,1]之间的凹度。

f(x) = x ² + 4

解决方案：

To check for the concavity, let’s look at the second derivative of the function.. 

f(x) = x² + 4

Differentiating w.r.t x, 

f'(x) = 2x 

Differentiating w.r.t x again, 

f”(x) = 2 > 0 

This is greater than zero, the function is concave upwards in the given interval. 

问题2：找出给定函数在区间[2,4]之间的凹度。

f(x) = x ³ + 4

解决方案：

To check for the concavity, let’s look at the second derivative of the function.. 

f(x) = x³

Differentiating w.r.t x, 

f'(x) = 3x² 

Differentiating w.r.t x again, 

f”(x) = 6x > 0

This is greater than zero, the function is concave upwards in the given interval. 

问题 3：找出给定函数的拐点。

f(x) = x ³

解决方案：

To check for the point of inflection., let’s look at the second derivative of the function.. 

f(x) = x³ + 4

Differentiating w.r.t x, 

f'(x) = 3x² 

Differentiating w.r.t x again, 

f”(x) = 6x = 0 

Solving f”(x) = 0, it shows that x = 0 is the critical point of f'(x). 

Now let’s check the value of f”(x) before and after the critical point. 

for x < 0, f”(x) < 0.  

for x > 0, f”(x) > 0. 

Thus, the function changes from concave downward to concave upward. Thus, x = 0 is the inflection point. 

问题 4：找出给定函数的拐点。

f(x) = x ³ + x ² + 3

解决方案：

To check for the point of inflection., let’s look at the second derivative of the function.. 

f(x) = x³ + x² + 3

Differentiating w.r.t x, 

f'(x) = 3x² + 2x 

Differentiating w.r.t x again, 

f”(x) = 6x + 2 = 0 

Solving f”(x) = 0, it shows that x = $\frac{-1}{3}$ is the critical point of f'(x). 

Now let’s check the value of f”(x) before and after the critical point. 

for x < -1, f”(x) < 0.  

for x > 0, f”(x) > 0. 

Thus, the function changes from concave downward to concave upward. Thus, x = $\frac{-1}{3}$ is the inflection point. 

问题 5：找出给定函数的拐点。

f(x) = x ⁴ – 2x ² + 3x

解决方案：

To check for the point of inflection., let’s look at the second derivative of the function.. 

f(x) = x⁴ – 2x² + 3x

Differentiating w.r.t x, 

f'(x) = 4x³ – 4x + 3

Differentiating w.r.t x again, 

f”(x) = 12x² – 4x + 3= 0 

Solving f”(x) = 0, it shows that x = $\frac{-1}{3}$ is the critical point of f'(x). 

Now let’s check the value of f”(x) before and after the critical point. 

for x < -1, f”(x) < 0.  

for x > 0, f”(x) > 0. 

Thus, the function changes from concave downward to concave upward. Thus, x = $\frac{-1}{3}$ is the inflection point. 

问题 6：找出区间 [0,1] 的给定函数的拐点。

f(x) = $\frac{ x + 2}{x + 1}$

解决方案：

To check for the point of inflection., let’s look at the second derivative of the function.. 

f(x) = $\frac{ x + 2}{x + 1}$

Differentiating w.r.t x, 

f'(x) = $\frac{(x + 1) - (x + 2)}{(x + 1)^2}$

⇒ f'(x) = $\frac{-1}{(x + 1)^2}$

Differentiating w.r.t x again, 

f”(x) = $\frac{-2}{(x + 1)^3}$

Solving f”(x) = 0 for the given interval. There is no solution to this equation in the given interval. 

Thus, inflection point does not exist in [0,1].