如果菱形的一边长5cm，对角线长8cm，求另一条对角线的长度。

菱形也称为四边形四边形。它被认为是平行四边形的特例。菱形包含平行的对边和相等的对角。菱形也称为钻石或菱形钻石。菱形包含长度相等的菱形的所有边。此外，菱形的对角线以直角相互平分。

菱形的性质

菱形包含以下属性：

• 菱形包含所有相等的边。
• 菱形的对角线以直角相互平分。
• 菱形的对边本质上是平行的。
• 菱形的两个相邻角之和等于180 ^° 。
• 菱形内没有内切圆。
• 菱形周围没有外接圆。
• 菱形的对角线导致形成四个直角三角形。
• 这些三角形彼此全等。
• 菱形的对角相等。
• 当你连接菱形边的中点时，就形成了一个矩形。
• 当一半对角线的中点相连时，就形成了另一个菱形。

菱形的对角线

菱形有四个由顶点连接的边。在连接菱形的相对顶点时，会形成额外的边，从而形成菱形的对角线。因此，菱形可以有两条对角线，每条对角线都以 90° 角相交。

菱形对角线的性质

菱形的对角线具有以下性质：

• 对角线以直角相互平分。
• 菱形的对角线分成四个全等直角三角形。
• 菱形的对角线长度可能相等也可能不相等。

菱形对角线的计算

菱形对角线的长度可以通过以下方法计算：

由毕达哥拉斯定理

让我们假设 d ₁是菱形的对角线。

因为，我们知道，菱形中的所有相邻边对向它们之间的角度为 90 度。

因此，如果我们考虑菱形 ABCD，

在三角形中，我们有 BCD，

自从，

垂直² + 底边² = 斜边²

放置线段，

BC ² + CD ² = BD ²

现在，我们有，

在正方形菱形的情况下，所有边都相等，

我们知道，

Square Diagonal: a√2

where a is the length of the side of the square

在矩形菱形的情况下，我们有，

Rectangle Diagonal: √[l² + b²]

where,

• l is the length of the rectangle.
• b is the breadth of the rectangle.

如果菱形的一边长5cm，对角线长8cm，求另一条对角线的长度。

解决方案：

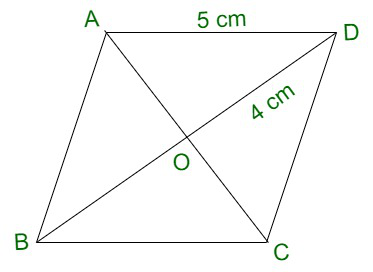
Since, we have,

Length of diagonal of the rhombus = 4 cm

Length of the side of the rhombus = 5 cm

We know, Diagonals of a rhombus bisect each other and they are perpendicular to each other. 

Let us consider half of the length of second diagonal to be x. 

Since, both the diagonals and a side form right angled triangle in case of a rhombus.

Therefore,

By Pythagoras theorem, we have, 

Perpendicular ² + Base ² = Hypotenuse ²

⇒ x² + 4² = 5²

⇒ x² = 25 – 16

⇒ x² = 9

⇒ x = √9

⇒ x = 3

Thus, we get, length of second Diagonal d₂ = 2x 

⇒ 2 × 3 = 6 cm

Therefore,

The length of second diagonal d₂ is 6 cm.

示例问题

问题 1. 菱形的面积是 10 平方厘米。如果对角线的长度是对角线的两倍。然后求两条对角线的长度？

解决方案：

Assume,

The shorter diagonal be d₁

The longer diagonal be d₂

As it is given in the problem that the longer diagonal is twice the shorter diagonal

Thus this can be written as 

d₂ = 2 × d₁……….equation (1)

The area of the Rhombus is

A = $\frac{d_1\times d_2}{2}$

10 = $\frac{d_1\times d_2}{2}$

10 = $\frac{d_1\times (2\times d_1)}{2}$ ……..from equation (1)

10 = $d_1^2$

d₁ = √10

d₂ = 2 × √10

d₂ = 2√10

问题 2. 计算边长为 34 厘米，对角线长为 32 厘米的菱形的面积。

解决方案：

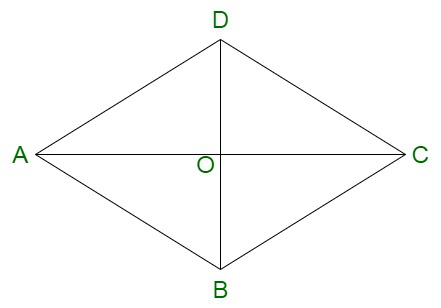
Here assume ABCD is a rhombus

Thus,

34 cm = AB = BC = CD = AD

Diagonal BD = 32 cm 

Here O is the diagonal intersection point

Thus, 

BO = OD = 16 cm

Further.

In the ∆ AOD,

AD² = AO² + OD²

⇒ 34² = AO² + 16²

⇒ 1156 = AO² + 256

⇒ AO² = 1156 – 256

⇒ AO² = 900

⇒ AO = 30 cm

As, 

AC = 2 × AO

= 2 × 30

AC = 60 cm

Area of rhombus = $\frac{d_1\times d_2}{2}$

= $\frac{32\times 60}{2}$

= 960 cm²

问题 3. 计算面积为 630 平方厘米、周长为 360 厘米的菱形的高度。

解决方案：

Here it is given that 

The perimeter of rhombus is 360 cm

Therefore, 

The side of rhombus = $\frac{Perimeter\ of\ Rhombus}{4}$ = $\frac{360}{4}$ = 90 cm

Now, 

The area of rhombus = base × height

⇒ 630 = 90 × h

⇒ h = $\frac{630}{90}$

⇒ h =7 cm

Hence, 

The altitude of the rhombus is 7 cm.

问题 4. 一栋建筑物的大厅由 4000 块菱形瓷砖组成，对角线分别为 80 厘米和 50 厘米。以每平方米20卢比的成本计算大厅所有瓷砖的抛光成本？

解决方案：

Here it is given that the length of the diagonals of each rhombus-shaped tile is 80 cm and 50 cm.

Thus,

The area of one tiles is; a = $\frac{d_1\times d_2}{2}$

a = $\frac{80\times50}{2}$

a = 2000 sq. cm

Changing the area is square metre

a = $\frac{2000}{10000}$

a = 0.2 sq. metre

Further the area for 4000 tiles will be; = 4000 × 0.2 sq.m

                                                            = 800 sq.m

Cost of buffing 1 sq.m is ₹ 20

Cost of buffing 800 sq.m will be; 800 × 20

= ₹1600

问题 5. 假设菱形玻璃的对角线是 15 厘米和 24 厘米。计算菱形玻璃的面积？

解决方案：

We know, 

Area of rhombus = $\frac{d_1\times d_2}{2}$

a = $\frac{15\times 24}{2}$

a = 180 sq.cm

Therefore, 

The area of the Rhombus shapes glass is 180 sq.cm.