📌  相关文章
📜  匀加速运动

📅  最后修改于: 2022-05-13 01:57:39.093000             🧑  作者: Mango

匀加速运动

当一个物体在平面或直线上移动时，使用三个参数来描述它的运动——距离、速度和加速度。距离或位移是不言自明的。速度代表位置的变化率，而加速度代表速度的变化率。这三个量都是向量。加速度可以是均匀的或不均匀的。匀加速具有恒定的值和方向。了解描述物体在匀加速下的运动的运动方程是很重要的。让我们详细看看它们。

加速

加速度定义为速度矢量的变化率。加速度可以是恒定的或变化的。在恒定加速度的情况下，其值由速度的净变化与所用总时间的比率给出。它也被称为平均加速度。在加速度随时间变化的情况下，计算瞬时加速度。

平均加速度：

\vec{a} = \frac{\vec{v_f} - \vec{v_i}}{\Delta t}

瞬时加速度：

\vec{a} = \frac{d\vec{v}}{dt} \\ = \vec{a} = \frac{dx}{dx}\hat{i} + \frac{dy}{dt}\hat{j}

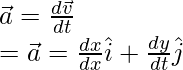
匀加速

匀加速度是不随时间变化的加速度。在这种情况下，速度的变化率保持不变。由于加速度是一个向量，所以在恒定加速度的情况下，即使运动的方向也保持不变。由于身体以恒定的加速度在一个方向上移动，因此可以删除矢量符号。

恒定加速度的一些示例包括

  1. 自由落体的物体。
  2. 一个球滚下无摩擦的斜坡。
  3. 已接合刹车的自行车。

匀加速运动方程

在这种情况下，保持加速度值不变。可以描述运动方程。假设一个物体的初始速度是“u”，现在施加一个恒定的力，使物体以恒定的加速度“a”移动，并且物体在时间“t”达到速度，同时覆盖距离“s”。

第一运动方程

在恒定加速度的情况下，其值由下式给出，

a = \frac{v - u}{t} \\ =at = v - u \\ =v = u + at

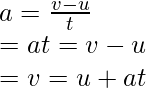
第二运动方程

瞬时速度由下式给出，

v = \frac{ds}{dt}

这个方程可以重新排列成以下形式，

ds = vdt

代入上式中的速度值，

ds = (u + at)dt

融合双方，

\int^{s_2}_{0}ds = \int^{t}_{0}(u + at)dt \\ s = \int^{t}_{0}udt + \int^{t}_{0}atdt \\ s = u[t]^{t}_{0} + a[\frac{t^2}{2}]^{t}_{0} \\ s= ut + \frac{1}{2}at^2

第三运动方程

瞬时加速度和瞬时速度由下式给出，

一个=  \frac{dv}{dt}

v =  \frac{ds}{dt}

交叉乘以这两个方程，

a\frac{ds}{dt} = v\frac{dv}{dt} \\ = \int^{s}_{0}a\frac{ds}{dt} = \int^{v}_{u}v\frac{dv}{dt} \\ = as = [\frac{v^2}{2}]^{v}_{u} \\ = as = \frac{v^2 - u^2}{2} \\ = 2as =v^2 - u^2

v 2 = u 2 + 2as

示例问题

问题 1：如果物体以 2 m/s 2的加速度运动。如果初始速度是 15m/s，那么 5 秒后的速度是多少。

回答：

问题 2：如果物体以 -5 m/s 2的加速度运动。如果初始速度为 30m/s，那么 5 秒内的距离 co 是多少。

回答：

问题 3：如果物体以 -5 m/s 2的加速度运动。如果初始速度是 40m/s，那么 5 秒后的速度是多少。

回答：

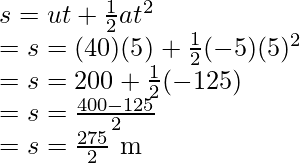
问题 4：如果物体以 10 m/s 2的加速度运动。如果初始速度是 20m/s，那么 2 秒内的速度是多少。

回答：

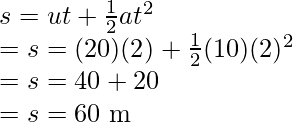
问题 5：一辆赛车在 2 秒内赶上 20m/s 的速度。在此过程中找到汽车所覆盖的距离。

回答：

问题 6：火箭在 5 秒内达到 50m/s 的速度。在这个过程中找到火箭所覆盖的距离。

回答：