这是用于能力倾向准备的TCS模型放置论文。这份安置文件将涵盖在TCS招聘活动中提出的才能问题，并且还将严格遵循在TCS面试中提出的问题模式。建议解决以下每个问题，以增加清除TCS面试的机会。
- 进行了考试，并对以下内容进行了分析。 4名男子能够在8天内定期工作5个小时检查一些试卷。 2个男人在20天内检查两倍于试卷的总小时数是多少?
Answer: 8 hours解决方案：
Assuming that 1 unit of work is done in 1 hour
Let’s calculate the total number of working hours:
=> 4 * 8 * 5 = 160 units
Now the work is doubled:
=> 160 * 2 = 320 units
Let ‘x’ be the number of hours taken by 2 men to complete the work in 20 days.
Therefore,
=> 2 * 20 * x = 320
=> x = 8 hours (Answer). - 从101到150的数字表示为101102103104105…146147148149150。用这个总数除以3得到的余数是多少?
Answer: 2解决方案：
The divisibility rule for 3 is that the sum of all digits of a number should be divisible by 3. Let’s calculate the sum of the digits:
There are 50 1’s (unit place) = 50
There are 10 1’s (tens place) = 10
There are 10 2’s (tens place) = 20
There are 10 3’s (tens place) = 30
There are 10 4’s (tens place) = 40
There is one 5 (tens place) = 5
For each number 1 to 9, there are 5 sets of sum 45(1+2+…+9) = 225
=> So sum of all digits = 380
=> 380 / 3 = 2 (Answer) - 如果字母以a，bb，ccc，dddd，eeeee，ffffff，…的顺序书写。第120个字母是什么?
Answer: O解决方案：
It can be seen that the letter are in AP sequence, So applying the formula we get,
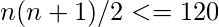
We find that n = 15 fits the equation
The 15th letter in the English alphabet = O
So 15th term contains O. - 有一个油箱的第1/7部分充满了燃料。如果将22升燃油倒入油箱，则指示器会升至油箱的1/5标记。那么水箱的总容量是多少?
Answer: 385解决方案：
Let the total capacity of the tank be ‘x’ liters.
According to the question,
=> x/7 + 22 = x/5
=> x/5 – x/7 = 22
=> x = 385 litres(Answer) - 满足条件的介于3到100之间的质数(不包括值)：
- 4x + 1
- 5年– 1
Answer: 2解决方案：
There are 23 prime numbers between 3 and 100 (excluding the values) of which all are odd.
For 5y – 1 to be odd, 5y must be even
For 5y to be even y should be even.
Taking y as 2 we get 5y – 1 as 9.
Now looking at all those prime numbers ending at 9 = 9, 19, 29, 39, 59, 79, 89
Out of these, the numbers satisfying both the equations(integer is assumed) are 9, 29 and 89.
We cannot consider 9 as it violates the constraint of number should be greater than 3.
Therefore answer is 2 (29 and 89) - 在给定的图中，找到正方形的面积与三角形的面积之比：
a)3：2
b)2：3
c)2：1
d)1：2Answer: c) 2:1解决方案：
Let the side of the square be ‘2’ units
Area of the square = (side)^2 =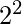
= 4 unit
Side of triangle, using Pythagoras Theorem =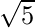
unit
Height of triangle = side of square (Using Pythgorean theorem)
Area of triangle = 1/2 * base * height
here, base = height = side
Area of triangle = 1/2 * (side)^2
=> 1/2 *2 * 2
=> 2 units
Therefore the ratio = 4:2
=> 2:1 (Answer) - 有一个仙女岛，住着骑士，K夫和间谍。您去那里遇到三个人，假设A，B和C，其中一个是骑士，一个是刀匠，一个是间谍。众所周知，骑士总是在讲真话，k徒总是在说谎，而间谍却可以说谎或讲真话。
- A说：“ C是个a夫。”
- B说：“ A是个骑士。”
- C说：“我是间谍。”
那么谁是骑士，谁的刀匠和谁的间谍呢?
Answer: A = Knight, B = Spy, C = Knave解决方案：
Let us say A is the Knight, then he speaks the truth and C is Knave who lied and finally B is Knave, who speaks the truth regarding A. So this condition holds.
Let us say B is the knight. then it contradicts the answer since a knight always speaks the truth and there cannot be two knights.
Same goes with C. - 在给定的2013、2020、2027，…………..，2300系列中找到理想平方的数量? (提示44 ^ 2 = 1936)
a2
b)1
c)3
d)以上都不是Answer: b) 1解决方案：
We can see that the series is in the form of AP with common difference of 7.
So the series is in the form of 2013 + 7d
The hint is actually a shortcut:
44^2 = 1936
45^2 = 2025
46^2 = 2116
47^2 = 2209
48^2 = 2304
Therefore among these numbers, we need to find which of them are in the form of 2013 + 7d
Only one number 2209 can be written in the form 2013 + 7*28.
Therefore the answer is 1. - 在7 ^ 1 + 7 ^ 2 + 7 ^ 3 + 7 ^ 4……。+ 7 ^ 204 + 7 ^ 205系列中，有多少个单位位置为3的数字?
Answer: 51Solution: According to the cyclicity of 7, the unit digit follows the pattern of 7, 9, 3, 1 and this repeats. So in every 4 numbers, we get one 3 in the unit place. Dividing 205 by 4 we get 51 which is the answer to the following question.
- 找出1728的除数个数(包括1和个数本身)。
Answer: 28解决方案：
There is a direct formula for this:
Number =
where p, q and r are prime numbers. Simply we need to prime factorize the Number.
Then, (a+1).(b+1).(c+1) is the number of divisors.
For 1728 =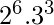
Therfore, (6+1).(3+1) = 28