TCS安置纸| MCQ 6

这是用于能力倾向准备的TCS模型放置论文。这份安置文件将涵盖在TCS招聘活动中提出的才能问题，并且还将严格遵循在TCS面试中提出的问题模式。建议解决以下每个问题，以增加清除TCS面试的机会。

1. 从安格斯发现的神秘卵中孵出的克鲁索迅速增长，安格斯不得不将其从家中转移到湖中。假设克鲁索出生后第一周的权重为5、15、30、135、405、1215和3645。找出奇数权重。
   a)3645
   b)135
   c)15
   d)30

   Answer: d) 30

   Solution:
   Looking at the series closely we find that the 3rd number is oddly placed.
   The series is in the form:
   5 * 3 = 15
   15 * 3 = 45
   45 * 3 = 135
   135 * 3 = 405 and so on

2. 假设f(1)= 0和f(m + n)= f(m)+ f(n)+4(9mn-1)。对于所有自然数(整数> 0)，m和n。 f(17)的值是多少?
   a)5436
   b)4831
   c)5508
   d)4832

   Answer: d) 4832

   Solution:
   We need to use f(1) to calculate the value of f(17)
   f(17) can be written as f(1+16)
   f(16) can be written as f(8+8)
   f(8) can be written as f(4+4)
   f(4) can be written as f(2+2)
   f(2) can be written as f(1+1)
   f(1) = 0, so f(2) = f(1+1) = f(1)+f(1)+4(9*1*1-1) = 32.
   or, f(4) = f(2+2) = f(2)+f(2)+4(9×2×2 – 1) = 32+32+4×35 = 204.
   or, f(8) = f(4+4) = f(4)+f(4)+4(9×4×4 – 1) = 204+204+4×143 = 980
   or, f(16) = f(8+8) = f(8)+f(8)+4(9×8×8 – 1) = 980+980+4×575 = 4260
   or, f(17) = f(1+16) = f(16)+f(1)+4(9×16×1 –1) = 4260+0+ 4×143 = 4832

3. 在P，Q和R之间分配的总和为Rs.3000。P获得Q和R的总和的2/3，R获得P和Q的总和的1/3，R的份额是多少?
   a)750
   b)850
   c)800
   d)700

   Answer: a) 750

   Solution:
   According to the question,
   case 1: P = 2(Q + R)/3
   or, (Q+R)/P = 3/2
   case 2: Also, R = (P+Q)/3
   or, (P+Q)/R = 3/1
   Simply using componendo-dividendo, we get,
   for case 1, (P+Q+R)/P = 3+2/2 = 5/2 = 20/8
   for case 2, (P+Q+R)/R = 3+1/1 = 4/1 = 20/5
   On solving we get, P = 8, Q = 7, R = 5
   or R’s share = 5/(8+7+5) * 3000 = 750

4. 在给定的系列11、23、47、83、131中，…下一个数字是什么?
   a)145
   b)178
   c)191
   d)176

   Answer: c) 191

   解决方案：

   The given series follows the order of multiple of 12
   23 – 11 = 12
   47 – 23 = 24
   83 – 47 = 36
   131 – 83 = 48
   x – 131 = 60
   or x = 191

5. 如果将数字除以357，则余数为5，如果将数字除以17，则余数将是多少?
   a)9
   b)3
   c)7
   d)5

   Answer: d) 5 

   Solution:
   Let the number be N when divided by 357 leaves remainder 5 and quotient q.
   So, N = 357k + 5 = 17 * 21 * k + 5
   So, 357 is exactly divisible by 17 so remainder is 5

6. 一条高36m的立杆在道路的某个边缘处以一定的高度折断。跌落的方式使杆的顶部触及道路的另一边缘。如果道路宽度为12m，那么杆断裂的高度是多少?
   a)12
   b)16
   c)24
   d)18

   Answer: b) 16

   Solution:
   Let the point at which the pole broke be ‘x’ from the ground, so the length of the broken piece be (36-x).
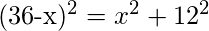
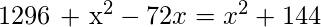
   So applying Pythagoras theorem we get, $$(36-x)^2 = x^2 + 12^2$$
   => $$1296 + x^2 - 72x = x^2 + 144$$
   => 72x = 1296 – 144
   => x = 16

7. 有一个由23人组成的大厅。他们在一起握手。那么，如果它们处于一对循环序列中，那么有多少次握手可能发生?
   a)23
   b)22
   c)253
   d)250

   Answer: c) 253

   Solution:
   Since there are 23 people, number of handshakes possible = 23C2 = 253 handshakes.

8. 在地下室，有一些自行车和汽车。在星期二，地下室有182个轮子。那里有几辆自行车?
   a)20
   b)19
   c)18
   d)16

   Answer: b) 19

   Solution:
   This is a very ambiguous question and must be calculated using the options.
   If there are 20 bicycles, there must be 20*2 = 40 wheels
   Remaining wheels = 182-40 = 142 wheels = 142/4 is not an integer so there cannot be 20 bicycles.
   Similarly checking for 19 bicycles = 19*2 = 38 wheels
   Remaining wheels = 182 – 38 = 144 = 144/4 = 36 cars hence this is the answer.

9. 有一个矩形地面17×8 m，被1.5 m宽度的路径包围。路径的深度为12厘米。填充沙子并找到所需的沙子量。
   a)5.5
   b)10.08
   c)6.05
   d)7.05

   Answer: b) 10.08

   Solution:
   Area of the inner rectangle = 17 * 8 = 136 meter-square
   Area of the outer rectangle = (17 + 2*1.5) * (8 * 2*1.5) = 220 meter-square
   So area of the remaining path = 220 – 136 = 84 meter-square
   So sand required to fill the path = 84 * (12/100) = 10.08 meter-square

10. 当数字272738和232342除以n(一个两位数字)时，分别剩下13和17的余数。求n的位数之和?
    a)5
    b)4
    c)7
    d)8

    Answer: c) 7

    Solution:
    So according to the question, (272738 – 13) and (232342 – 17) are exactly divisible by n.
    So if we find the HCF of these two numbers, we get n,
    The HCF of 272725 and 232325 is 25
    So the sum of the digits = 7.