带电粒子的流动称为电流。在当前的电流中,电荷流将是连续的。电流从较高电势传播到较低电势。
要使电流流过,需要一个由导电材料的闭环组成的电路。该电路由端对端连接并沿相同方向移动的电线组成。
当电子流通过导体时,它构成了电流。按照惯例,电流(I)的方向总是与电子或电荷的流动方向相反。
抵抗性
物理学家乔治·西蒙·欧姆(Georg Simon Ohm)发现了流动电流I之间的关系,该电流在金属线中流动,该金属线两端(两端)之间存在电位差。他指出:电路中给定金属线的端子两端的伏特V电位差与流过它的电流I成正比,前提是其温度保持不变。
这就是欧姆定律。换句话说,它可以写为:两端电位差∝电流
或者
∝
V = IR,其中R是比例常数,称为电阻。
电阻是一种抵抗(抵抗)电流流动的特性,或者可以说是导体中电子的流动。它控制流过电路的电流的大小(值)。
电阻的SI单位以欧姆为单位,用Ω表示。
在数学上,抗性可以定义为:
R = V / I
在这里,I是电流,V是电势差。
- 电流与电阻成反比。因此,电流越大,电阻越小,反之亦然。
- 诸如变阻器或可变电阻器之类的电阻设备允许改变电路中的电阻,从而使两端的电势差保持相同。
- 在导体中,电子被导体的原子吸引。对于良好的导体(金属,如铁,铜),电阻较低,而对于绝缘体(如木材,衣服,拖鞋),则电阻较高。其中具有一定电阻的导体称为电阻器。
欧姆定律实验
如欧姆定律所述:电阻两端的电势差与通过它的电流成正比,只要其温度保持不变即可。
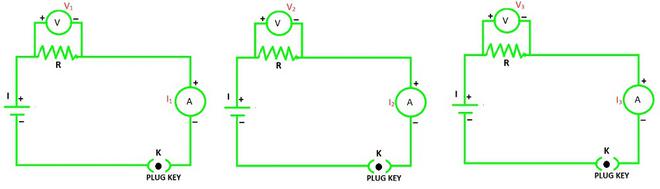
对于相同的电阻R,当采用三个不同的电压源时,所产生的电位差将始终与流经电路的电流成比例。这意味着:
V 1 ∝ I 1 ,
V 2 ∝ I 2 ,和
V 3 ∝ I 3
结果:
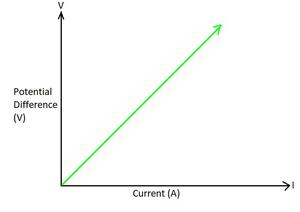
镍铬合金线的V–I图。
抵抗力取决于的因素
导体的电阻取决于:
- 它的长度(l),
- 其横截面积(A)和
- 其材料的性质。
均匀分布的金属导体的电阻与它的长度成正比,与它的横截面面积成反比。
因此,R 1和R 1 / A。
⇒R ∝ l / A
或者
⇒R =ρl/ A
其中ρ是比例常数,称为导体材料的电阻率。
- SI单位为Ω.m。
- 电阻率随温度(T)的变化而变化。
- 导体的电阻率值很低,而绝缘子的电阻率却很高。
- 具有比金属更高的电阻率的合金被用于电加热装置中,例如铁和烤面包机,铜和铝被用于电传输线,并且钨被用于电灯泡的灯丝。
- 因此,绝缘子的电阻率>合金的电阻率>导体的电阻率。
电阻组合
Resistors are used in various combinations. There are two methods of arranging the resistors in different combinations:
(i) Resistors in Series
(ii) Resistors in Parallel
Equivalent Resistance: The equivalent resistance of combinations of resistors in series is equal to the sum of their individual resistances in the circuit.
串联组合电阻:
当两个或多个电阻端对端连接并且相同的电流依次流过每个电阻时,它们被称为串联连接。在这种情况下,等效电阻或总电阻等于串联组合中存在的单个电阻的数量之和。
数学上,串联连接的任意数量的电阻(R 1 ,R 2 ,R 3 ,R 4 ,R 5 ,…..)的等效电阻为:
R eq = R 1 + R 2 + R 3 + R 4 + R 5 +……..
考虑以下电路中三个电阻(R 1 ,R 2和R 3 )与相应的电压源(V 1 ,V 2和V 3 )彼此串联连接的情况:

串联电阻
流过它的等效电流为I,通过电流表A和键K进行检测。
等效电位差等于每个电阻两端的电位差之和,即
V eq = V 1 + V 2 + V 3
通过每个电阻的电流I相同,即I = I 1 = I 2 = I 3
用等效的单个电阻R eq代替串联连接的三个电阻,以使两端的电位差V eq和流经电路的电流I保持不变。
将欧姆定律应用于电路:
V eq = IR eq
通过将欧姆定律分别应用于所有电阻器,如下所示:
V 1 = IR 1
V 2 =红外线2
V 3 =红外线3
因此,IR = IR 1 + IR 2 + IR 3
或者
Req = R 1 + R 2 + R 3
- 通过电路的电流在这里将保持不变。
- 等效电位差是每个电阻两端的电位差之和。
- 结果,等效电阻成为各个电阻的总和。
- 串联组合的唯一缺点是,如果串联组合中的任何电阻器被破坏或发生故障,则整个电路都将关闭。
- 需要串联组合以增加电阻并将高电位差分配到许多电阻上。
- 这种组合用于电阻箱,装饰灯等。
并联组合电阻:
当两个或多个电阻连接在两个点之间且每个点具有不同的电流方向时,它们被称为并联连接。当分支在这样的电路中的公共点处相交时,电流被分支出来并重新组合。
数学上,并联连接的任意数量的电阻(R 1 ,R 2 ,R 3 ,R 4 ,R 5 ,…..)的等效电阻为:
1 / R eq = 1 / R 1 + 1 / R 2 + 1 / R 3 + 1 / R 4 + 1 / R 5 + ……..
考虑以下电路中三个电阻(R 1 ,R 2和R 3 )与相应的电压源(V 1 ,V 2和V 3 )并联的情况:
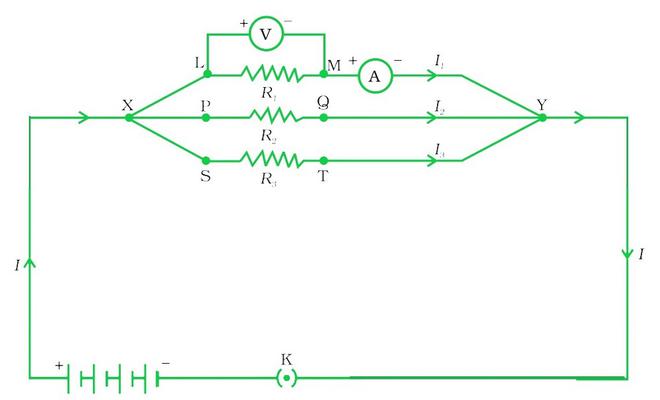
并联电阻
在这里,流经每个电阻的电流是不同的,因此,流经电路的等效电流为:
Ieq = I 1 + I 2 + I 3
将并联连接的三个电阻替换为R eq的并联电阻组合中的等效单个电阻。
现在,通过将欧姆定律应用于电阻的并联组合,如下所示:
当量= V / R当量
在将欧姆定律应用于单个电阻器时:
I 1 = V / R 1
I 2 = V / R 2
I 3 = V / R 3
因此,V / R eq = V / R 1 + V / R 2 + V / R 3
或者
1 / R eq = 1 / R 1 +1 / R 2 +1 / R 3
总之,可以说并联连接的一组电阻的等效电阻的倒数等于各个电阻的倒数之和。
- 流经电路的等效电流是流经电路各分支的单个电流的总和。
- 电路两个端点之间的电位差保持不变。
- 结果,电路的等效电阻的倒数是各个电阻的倒数之和。
- 在并联电路中,可以容易地连接或断开电阻器或某些其他组件,而不会干扰其他组件。
- 在并联组合中,电路中流动的电流分为不同的分支,因此每个组件都接收所需的电流量。
- 在此,等效电阻始终小于所有单个电阻。
- 如果其中一个组件发生故障或短路,则电路的其余组件将正常工作。
样本问题
问题1:如果电灯的电阻为1000Ω,则从220 V电源中汲取多少电流?
解决方案:
Given that,
The source voltage, V is 220 V.
The resistance of the lamp, R is 1000 Ω.
The formula to calculate the current drawn is:
I = V / R
Substitute the given values in the above expression as:
I = 220 V / 1000 Ω
= 0.22 A
Hence, the current drawn through the electric lamp is equal to 0.22 A.
问题2:如果灯泡灯丝的电阻为200Ω,那么一个灯泡会从220 V电源汲取多少电流?
解决方案:
Given that,
The source voltage, V is 220 V.
The resistance of the bulb, R is 200 Ω.
The formula to calculate the current drawn is:
I = V / R
Substitute the given values in the above expression as:
I = 220 V / 200 Ω
= 1.1 A
Hence, the current drawn through the electric bulb is equal to 1.1 A.
问题3:当从电源汲取6A电流时,电灯泡的端子之间的电位差为30V。如果电势差增加到120 V,灯泡将汲取什么电流?
Given that,
The potential difference across the electric bulb, V is 30 V.
The current drawn, I is 6 A.
The increased potential difference, R’ is 120 V.
According to Ohm’s law, the formula to calculate the resistance is:
R = V / I
= 30 V / 6 A
= 5 Ω
When the potential difference is increased to 120 V the current drawn is:
I’ = V / R’
Substitute the given values in the above expression as:
I’ = 120 V / 5 Ω
= 24 A
Hence, the current drawn through the electric bulb is equal to 24 A.
问题4:某根长度为l且横截面面积为A的给定材料的导线的电阻为4Ω。长度相同且长度为l / 2的相同材料的另一根导线的电阻为多少? 2A?
解决方案:
Consider the resistance of the first wire as:
R1 = ρl / A
where ρ is the resistivity, l is the length and A is the cross-sectional area of the first wire.
But it is given that the resistance, R1 is 4 Ω.
Therefore,
4 Ω = ρl / A ……(1)
Now, in case of second wire:
The length of the wire, l2 is l/2 and
The cross-sectional area, A2 is 2A.
Therefore, the resistance for second wire becomes:
R2 = ρl2 / A2
= ρ(l/2) / (2A)
= ρl / 4A
Substitute 4 Ω for ρl / A, from equation (1) in the above expression.
R2 = 4 Ω / 4
= 1 Ω
Hence, the resistance of the second wire is 1 Ω.
问题5:串联电阻为40Ω的电气设备和电阻为12Ω的导体串联连接的8 V电池。计算 :
(a)电路的总电阻,
(b)通过电路的电流,以及
(c)每个电阻的电位差。
解决方案:
The given problem can be represented diagrammatically as:
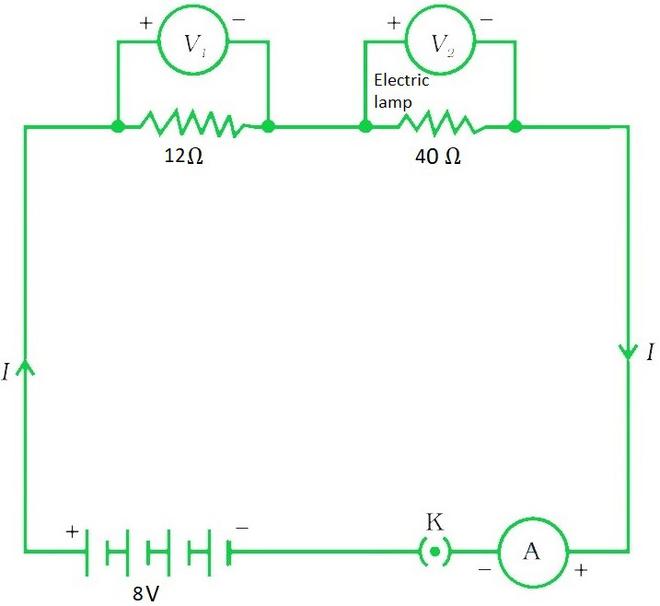
(a) Given that,
The resistance of electric lamp, R1 = 40 Ω,
The resistance of the conductor connected in series, R2 = 12 Ω.
Therefore, the total resistance of the circuit is:
R = R1 + R2
Substitute the given values in the above expression.
Req = 40 Ω + 12 Ω
= 52 Ω
Hence, the total resistance in the circuit is 52 Ω.
(b) Again, it is given in the problem that,
The total potential difference across the two terminals of the battery, V is 8 V.
Using Ohm’s law, the current flow in the circuit is:
I = V / Req
I = 8 V / 52 Ω
= 0.15 A
Hence, the current through the circuit is 0.15 A
(c) Again, apply Ohm’s law separately to the electric lamp and conductor, the potential difference across the electric lamp is:
V1 = I × R1
= 40 Ω × 0.15 A
= 6 V
And the potential difference across the conductor is,
V2 = I × R2
= 12 Ω × 0.15 A
= 1.8 V
问题6:考虑一个由三个电阻组成的电路:R 1 = 6Ω,R 2 = 18Ω 通过12 V的源电池将R 3 = 36Ω并联组合。计算:
(a)通过每个电阻的电流,
(b)电路中的总电流,以及
(c)总电路电阻。
解决方案:
The given problem can be represented diagrammatically as:
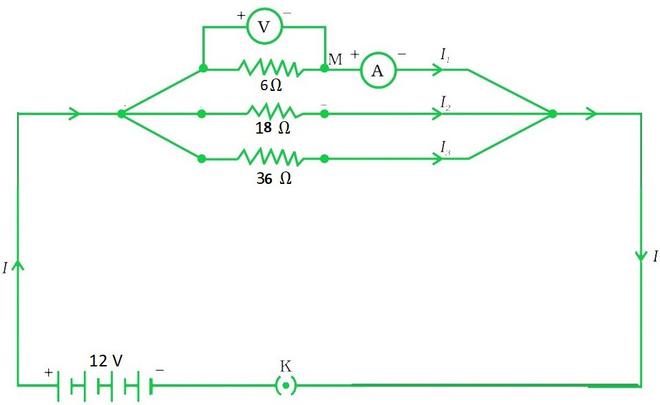
The resistances connected in parallel combination are: R1 = 6 Ω, R2 = 18 Ω, and R3 = 36 Ω.
The potential difference across the battery, V is 12 V.
Since, the circuit has resistors connected in parallel, then the potential difference across the terminals of the individual resistor will be same.
Hence, to calculate the current in the resistors, use Ohm’s law as:
I1 = V / R1
= 12 V / 6 Ω
= 2 A
Similarly,
I2 = V / R2
= 12 V / 18 Ω
= 0.66 A
And
I3 = V / R3
= 12 V / 36 Ω
= 0.33 A
Therefore, the total current in the circuit,
I = I1 + I2 + I3
= (2 + 0.66 + 0.33) A
= 2.99 A
Hence, the current through each resistor is 2 A, 0.66 A and 0.33 A and the total current in the circuit is 2.99 A.
The total resistance Req is:
1 / Req = 1 / R1 + 1 / R2 + 1 / R3
= 1/ 6 Ω + 1/ 18 Ω + 1/ 36 Ω
Req = 4 Ω
Hence, the total circuit resistance is 4 Ω.