📌  相关文章
📜  电阻组合问题

📅  最后修改于: 2022-05-13 01:57:38.577000             🧑  作者: Mango

电阻组合问题

电阻器是对电流提供电阻的器件。在我们的日常生活中，我们的设备在电路中有不止一个电阻。研究不同电阻排列的影响及其对电路的影响变得至关重要。通常在实际情况下，需要计算整个电路或有时部分电路所需的电阻。在这种情况下，计算等效电阻的知识会很有用，让我们详细了解这些概念。

电阻器和电阻

电阻器是限制电路中电流流动的电气设备。它是一种欧姆器件，这意味着它遵循欧姆定律 V = IR。大多数电路只有一个电阻器，但有时电路中可以存在多个电阻器。在这种情况下，流过电路的电流取决于组合的等效电阻。这些组合可以任意复杂，但可以分为两种基本类型：

  1. 系列组合
  2. 并联组合

系列组合

在下图中，三个电阻与电压为 V 的电池串联。在这些类型的组合中，电阻通常以一个接一个的顺序连接。通过每个电阻的电流是相同的。右图显示了三个电阻的等效电阻。在电阻串联组合的情况下，等效电阻由各个电阻的代数和给出。

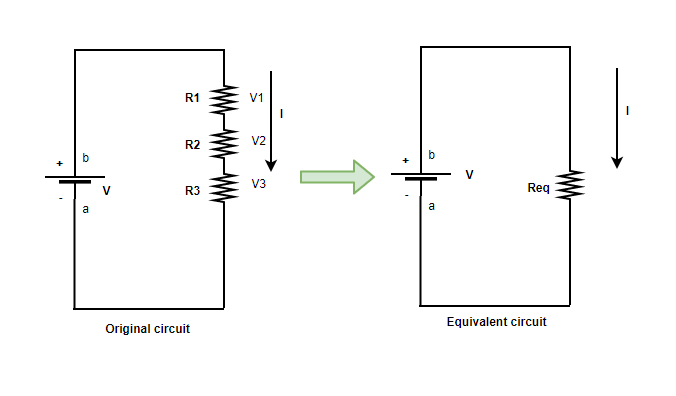
令 V 1 、V 2和 V 3为所有三个电阻上的电压。众所周知，流过它们的电流是相同的。

V = V 1 + V 2 + V 3

展开方程，

红外 = 红外1 + 红外2 + 红外3

并联组合

在下图中，显示了三个电阻器，它们与电压为 V 的电池并联。在这种连接中，电阻器通常连接在源自公共点的平行导线上。在这种情况下，通过每个电阻的电压是相同的。右图显示了三个电阻的等效电阻。

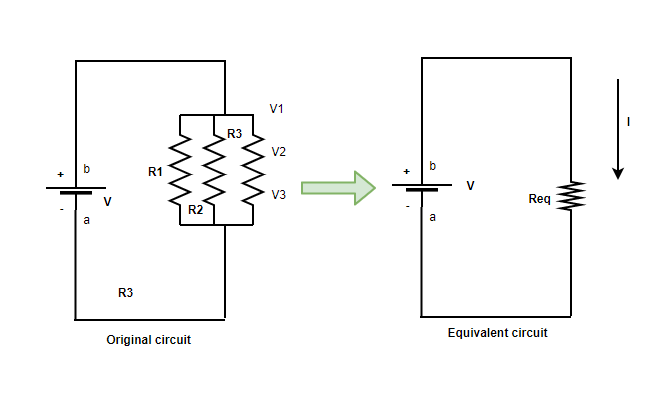
给定电路的等效电阻为，

\frac{1}{R} = \frac{1}{R_1} + \frac{1}{R_2} + \frac{1}{R_3}

示例问题

问题1：串联6、10、20欧三个电阻。求系统的等效电阻。

回答：

问题2： 1、1、2欧三个电阻并联。求系统的等效电阻。

回答：

问题 3：求下图所示系统的等效电阻。

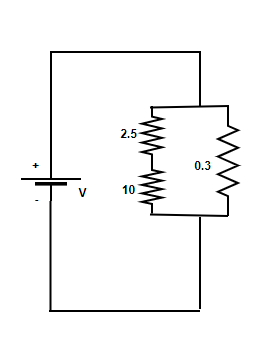
回答：

问题 4：求下图所示系统的等效电阻：

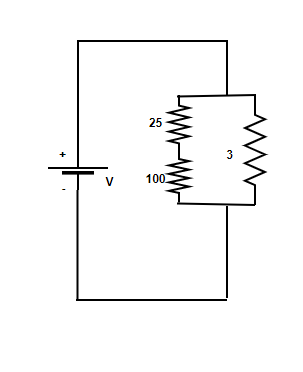
回答：

问题5：电加热器连接到电池。当另一个类似的电加热器与原来的电加热器串联时，电路的电阻如何变化？

  1. 抵抗力加倍。
  2. 阻力减半。
  3. 阻力保持不变。
  4. 阻力增加了三倍。

回答：

问题 6：找出下面给出的电路的等效电阻。

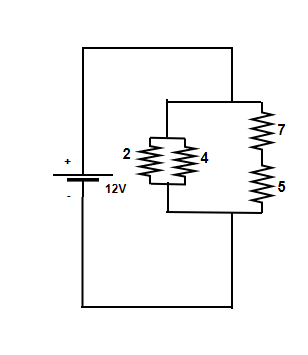
回答：