抛物线的焦点和方向

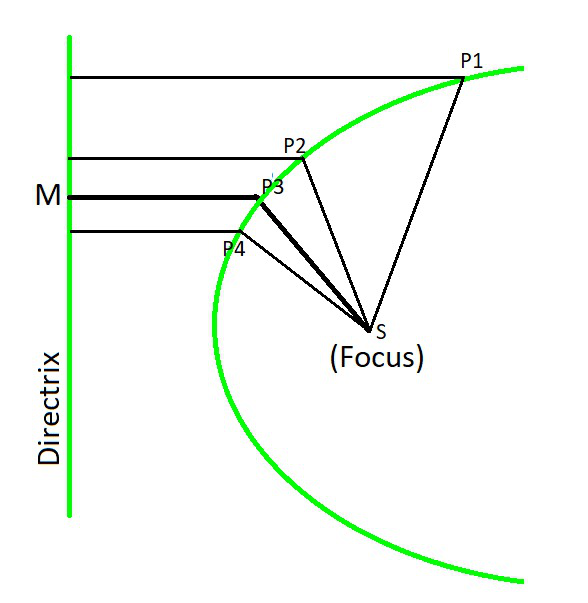
在数学中，抛物线是在平面中移动的点的轨迹，在该平面中，其距固定点的距离(称为焦点)始终等于在同一平面中距固定直线的距离(称为准线)。换句话说，抛物线是几乎呈U形的平面曲线，其中每个点都与固定点(称为焦点)和直线(称为准线)等距。抛物线只有一个焦点，而焦点永远不会放在准线上。如图所示，在下面图中，在其中P_的1M = P ₁ S，P ₂ M = P ₂ S，P ₃ M = P ₃ S和P_的4M = P ₄ S.

焦点和方向的抛物线方程

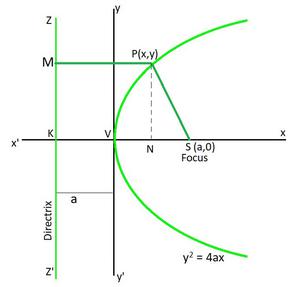
现在，我们将学习如何从焦点和准线找到抛物线方程。因此，令S为焦点，线ZZ’为准线。从S的垂直轴上绘制SK，然后在V处将SK等分。

VS = VK

V距焦点的距离= V距准线的距离

V位于抛物线上，因此，SK = 2a。

然后，VS = VK = a

让我们以V作为顶点，VK是垂直于ZZ’且平行于x轴的线。那么，焦点S的坐标为(h，k)，并且准线ZZ′的方程为x ＝ b。 PM与Directrix x = b垂直，并且点M将为(b，y)

让我们考虑抛物线上的点P(x，y)。现在，加入SP和PM。

我们知道P位于抛物线上

因此，SP = PM(帕拉波拉定义)

SP ² =下午²

(x – h) ² +(y – k) ² =(x – b) ² +(y – y) ²

x ² – 2hx + h ² +(yk) ² = x ² – 2bx + b ²

双方都加(2hx – b ² )，我们得到

x ² – 2hx + h ² + 2hx – b ² +(yk) ² = x ² – 2bx + b ² + 2hx – b ²

2(h – b)x =(yk) ² + h ² – b ²

将方程除以2(h – b)，我们得到

x = $\frac{(y-k)^2}{2(h-b)} + \frac{(h-b)(h+b)}{2(h-b)}$

x = $\mathbf{\frac{(y-k)^2}{2(h-b)} + \frac{(h+b)}{2}}$ ………………..(1)

同样，当Directrix y = b时，我们得到

y = $\mathbf{\frac{(x-h)^2}{2(k-b)} + \frac{(k+b)}{2}}$ ………………..(2)

当V为原点时，VS为长度a的x轴。那么，S的坐标将为(a，0)，且准线ZZ’为x = -a。

h = a，k = 0且b = -a

使用等式(1)，我们得到

x = $\frac{(y-0)^2}{2(a-(-a))} + \frac{(a+(-a))}{2}$

x = $\frac{y^2}{4a}$

y² = 4ax

它是抛物线的标准方程。

注意：抛物线在其轴上有两个真实的焦点，其中一个是焦点S，另一个位于无穷大。相应的方向也位于无穷大处。

抛物线的追踪y ² = 4ax，a> 0

给定方程可写为y =±2 $\sqrt{ax}$ ，我们从等式中观察到以下几点：

• 对称性：给定的方程式表明，对于x的每个正值，y都有两个相等且相反的值。
• 区域：给定的方程式表明，对于x的每个负值，y的值都是虚数的，这意味着曲线的任何部分都不位于y轴的左侧。
• 原点：原点是曲线穿过的点，原点处的切线为x = 0，即y轴。
• 所占部分：为x⇢∞，y⇢∞。因此，曲线延伸到y轴右侧的无穷大。

具有焦点和方向的抛物线的其他一些标准形式

抛物线方程的最简单形式是当顶点位于原点且对称轴与x轴或y轴一起时。此类抛物线类型为：

1. y ² = 4ax

这里，

• 顶点坐标：(0，0)
• 焦点坐标：(a，0)
• 准线方程：x = -a
• 轴方程：y = 0
• 子宫直肠长度：4a
• 点P(x，y)的焦距：a + x

2. x ² = 4ay

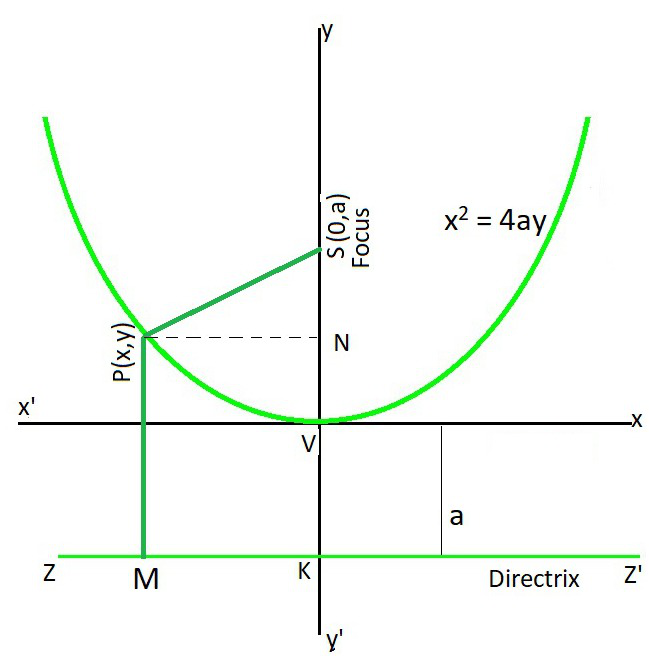
这里，

• 顶点坐标：(0，0)
• 焦点坐标：(-a，0)
• 准线方程：x = a
• 轴方程：y = 0
• 子宫直肠长度：4a
• 点P(x，y)的焦距：a – x

3. y ² = – 4ay

这里，

• 顶点坐标：(0，0)
• 焦点坐标：(0，a)
• 准线方程：y = -a
• 轴方程：x = 0
• 子宫直肠长度：4a
• 点P(x，y)的焦距：a + y

4. x ² = – 4ay

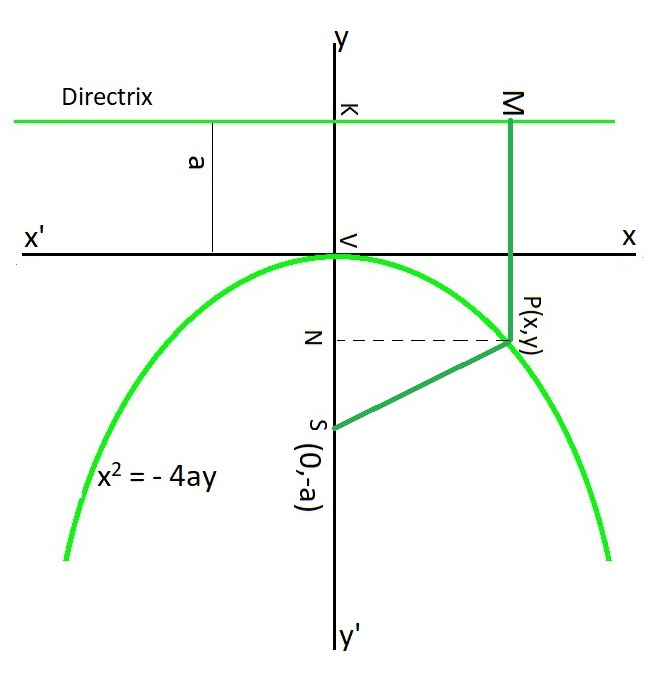
这里，

• 顶点坐标：(0，0)
• 焦点坐标：(0，-a)
• 准线方程：y = a
• 轴方程：x = 0
• 子宫直肠长度：4a
• 点P(x，y)的焦距：a – y

样本问题

问题1.找到抛物线方程，其焦点为(-4，2)，且准线为x + y = 3。

解决方案：

Let P (x, y) be any point on the parabola whose focus is (-4, 2) and the directrix x + y – 3 = 0. 

As we already know that the distance of a point P from focus = distance of a point P from directrix

So, √(x + 4)² + (y – 2)² = $|\frac{x+y-3}{\sqrt{1^2+1^2}}|$

On squaring both side we get

(x + 4)² + (y – 2)² = $|\frac{x+y-3}{\sqrt{2}}|^2$

2((x² + 16 + 8x) + (y²+ 4 – 4y)) = x² + y² + 9 +2xy – 6x – 6y

2(x² + 20 + 8x + y² – 4y) = x² + y² + 9 +2xy – 6x – 6y

2x² + 40 + 16x + 2y² – 8y = x² + y² + 9 +2xy – 6x – 6y

x² + y² + 2xy + 10x – 2y + 31 = 0

问题2。找到抛物线的方程，其焦点为(-4，0)，且方向数x + 6 = 0。

解决方案：

Let P (x, y) be any point on the parabola whose focus is (-4, 0) and the directrix x + 6 = 0. 

As we already know that the distance of a point P from focus = distance of a point P from directrix

So,  √(x + 4)² + (y )² = $|\frac{x+6}{\sqrt{1^2+1^2}}|$

On squaring both side we get

(x + 4)² + (y)² = $|\frac{x+6}{\sqrt{2}}|^2$

2x² + 32 + 16x + 2y² = x² + 36 + 12x

x² + 2y² – 4 + 14x = 0

问题3.求出抛物线方程的焦点为(4，0)，且方向数x = – 3。

解决方案：

Since the focus (4, 0) lies on the x-axis, the x-axis itself is the axis of the parabola. 

Hence, the equation of the parabola is of the form either

y² = 4ax or y²= – 4ax. 

Since the directrix is x = – 3 and the focus is (4, 0),

 the parabola is to be of the form y²= 4ax with a = 4. 

Hence, the required equation is

y² = 4(4)x 

y² = 16x

问题4.找到抛物线方程，其顶点位于(0，0)，聚焦在(0，4)。

解决方案：

Since the vertex is at (0, 0) and the focus is at (0, 5) which lies on y-axis, the y-axis is the axis of the parabola. 

Hence, the equation of the parabola is x²= 4ay. 

Hence, we have x² = 4(4)y, i.e., 

x² = 16y

方程中抛物线的焦点和方向

现在，我们将学习如何从方程式中找到抛物线的焦点和方向。

因此，当抛物线方程为

y – k = a(x – h) ²

在这里，a的值= 1 / 4C

因此焦点是(h，k + C)，顶点是(h，k)，方向数是y = k –C。

范例范例

问题1. y ² = 8x

解决方案：

The given parabola is of the form y² = 4ax, where

4a = 8

a = 2

The coordinates of the focus are (a,0),  i.e. (2,0) 

and, the equation of the directrix is 

x = -a, i.e. x = -2

问题^{2。y 2} – 8y – x + 19 = 0

解决方案：

By rearranging, we get

y² – 8y + 16 – x + 3 = 0

y² – 8y + 16 = x – 3

x = (y-4)² + 3

Comparing with eq(1), we conclude

k = 4

2(h-b) = 1 ……………(I)

$\frac{(h+b)}{2}$ = 3 ……………(II)

Solving (I) and (II), we get

h = $\frac{13}{4}$ and b = $\frac{11}{4}$

Hence, Focus is (h,k) = ($\frac{13}{4}$,4)

and, directrix x = b = $\frac{11}{4}$

问题3。找到以下方程式的焦点，方向线和顶点：y = x ² – 2x + 3

解决方案：

By rearranging, we get

y =x² – 2x + 4 – 1

y =(x-1)² + 2

Comparing with eq(4), we conclude

h = 1

y₁ = 2

2(k-b) = 1 ……………(I)

$\frac{(k+b)}{2}$ = 2 ……………(II)

Solving (I) and (II), we get

k =$\frac{9}{4}$

and b = $\frac{7}{4}$

Hence, Focus is (h,k) = ( 1, $\frac{9}{4}$), 

directrix y = b = $\frac{7}{4}$ 

and, vertex (h, y₁) = (1,2)