抛物线的对称轴

对称的字典含义是“这样的比例和平衡，既和谐又有吸引力。”但它在数学上有更准确的定义，一般是指在一定的变换下稳定的物体，如旋转、镜像或平移。对称的形式或图形是可以有一条线穿过它们的东西，以便线两侧的表示相互镜像。看看下面的三角形，当它被一条线段分割时，分成两个相同的形状，就像彼此的镜面反射一样。

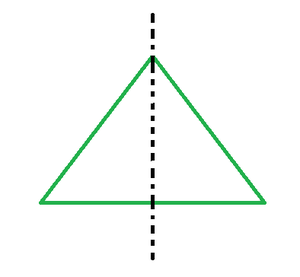
对称轴

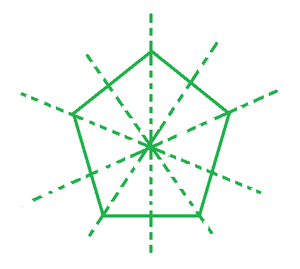
一条将物品分成相等两半的线，提供物体两侧的镜面复制品，称为对称轴。对称这个词意味着一种平衡感。对称性可用于多种情况和场景。对称性是几何学中的一个基本概念，它将一个形状分成两半，每一半都是另一半的完美反映，如下图所示。不同的形式有不同的对称线。正方形有四条对称线，长方形有两条对称线，圆形有无限条对称线，平行四边形没有。一个有 n 条边的正多边形有 n 个对称轴。

五边形的对称轴如下图所示：

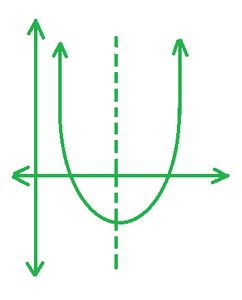
抛物线的对称轴

抛物线只有一条对称线。将抛物线分成两个对称部分的直线是对称轴。有四种不同类型的抛物线。它可能是水平的或垂直的，它可以朝左或朝右。抛物线的形状由它的对称轴决定。当其对称轴垂直时，抛物线是垂直的，反之亦然。

抛物线对称轴公式

对于具有 ax ² + bx + c 形式的方程的抛物线，可以使用以下公式计算对称轴：

x = −b/2a

where a and b are the coefficients of x² and x respectively and c is the constant.

公式的推导

The vertex of parabola is the only point from where the axis of symmetry passes. A vertical parabola’s quadratic equation is y = ax² + bx + c

The parabola is unaffected by the constant term ‘c.’

Consider the equation y = ax² + bx.

The axis of symmetry is the midpoint of its two x-intercepts. To find the x-intercept, substitute y = 0.

⇒ x(ax + b) = 0

⇒ x = 0 or, x = -b/a

Using the mid- point formula, we have:

⇒ x = $\frac{0+[-\frac{b}{a}]}{2}$

⇒ x = -b/2a

Hence proved.

示例问题

问题 1. 求抛物线的对称轴 y = x ² − 4x + 8。

解决方案：

Given: y = x² − 4x + 8

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 1, b = −4, c = 8

Axis of symmetry = −b/2a

= −(−4)/2(1)

⇒ x = 2

问题 2. 求抛物线 y = 4x ²的对称轴。

解决方案：

Given: y = 4x²

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 4, b = 0, c = 0

Axis of symmetry = −b/2a

= 0/2(4)

⇒ x = 0

问题 3. 求抛物线 y = 7x ²的对称轴。

解决方案：

Given: y = 7x²

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 7, b = 0, c = 0

Axis of symmetry = −b/2a

= 0/2(7)

⇒ x = 0

问题 4. 求抛物线的对称轴 y = x ² + 8x − 3。

解决方案：

Given: y = x² + 8x − 3

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 1, b = 8, c = –3

Axis of symmetry = −b/2a

= –8/2(1)

⇒ x = –4

问题 5. 求抛物线的对称轴 y = 2x ² + 12x。

解决方案：

Given: y = 2x² + 12x

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 2, b = 12, c = 0

Axis of symmetry = −b/2a

= −12/2(2)

⇒ x = −3

问题 6. 求抛物线的对称轴 y = 3x ² − 6x + 5。

解决方案：

Given: y = x² − 6x + 5

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 1, b = −6, c = 5

Axis of symmetry = −b/2a

= −(−6)/2(3)

⇒ x = 1

问题 7. 求抛物线 y = 9x ²的对称轴。

解决方案：

Given: y = 9x²

Compare the given equation to the standard form ax² + bx + c.

⇒ a = 9, b = 0, c = 0

Axis of symmetry = −b/2a

= 0/2(9)

⇒ x = 0