极限的性质

极限构成了微积分理论的基础。函数的限制用于定义函数的派生，检查函数的连续性，等等。在某个特定点上，函数极限的直觉值使我们对函数的逼近值有了一个认识。请注意，在计算限制时，我们并未计算该特定点的函数的确切值。我们对寻找函数接近的方向或点更感兴趣。让我们定义限制并更详细地查看属性。

限度

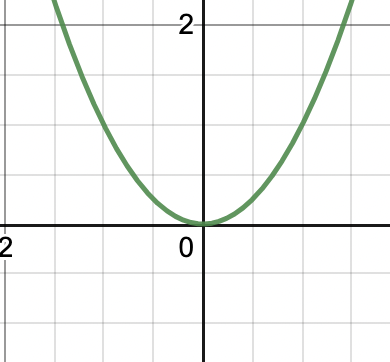
在微积分中使用极限来定义微分，连续性，积分，并且将其定义为函数的逼近值，而输入趋近于确定值。假设我们有一个函数f(x)= x ² 。在下面给出的图中，请注意，当x⇢0时，f(x)也趋于变为零。可以写成极限，因为$\lim_{x \to 0} f(x) = 0$ 。由于x趋于零，因此将其视为f(x)的极限。

In general, as x ⇢ a, f(x) ⇢ l, then l is called the limit of the function f(x). It can also be written as, 

$\lim_{x \to a}f(x) = l$

有时某些功能是不连续的，即当从两侧接近时，它们似乎正在接近两个不同的值。例如，让我们看下图所示的步骤函数。

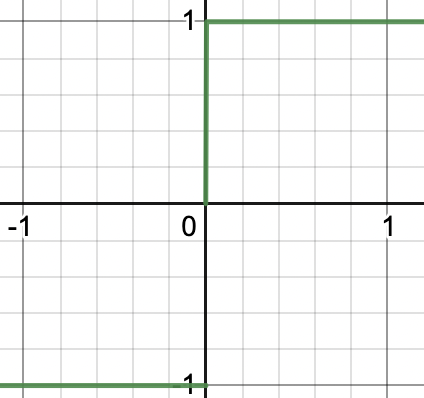
此函数可以定义为：

$f(x)= \begin{cases} 1,& \text{if } x > 0\\ 0,& \text{if } x = 0\\ -1,& \text{otherwise} \end{cases}$

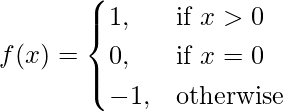
假设我们要接近零并看到函数的极限。这自然会引出我们可以采取的方向。左侧和右侧限制。右侧极限是从所需点的右侧接近它时所采用的函数的值。同样，左侧极限是从左侧接近时的函数值。

对于此特定函数，

左侧极限， $\lim_{x \to 0^-}f(x) = -1$

右侧极限$\lim_{x \to 0^+}f(x) = 1$

极限代数

假设我们有两个函数f(x)和g(x)。我们知道$\lim_{x \to a}f(x)$和$\lim_{x \to a}g(x)$存在。下面给出的属性描述了这两种功能以不同方式组合时极限的行为。这些内容未经证明就提供了，但是我们将看到一些有关这些属性的示例来进行验证。

属性1：

 Limit of sum of two functions is the sum of limits of both the functions. 

$\lim_{x \to a}[f(x) + g(x)] = \lim_{x \to a}f(x) + \lim_{x \to a}g(x)$

属性2：

 Limit of difference of two functions is the difference of limits of both the functions. 

$\lim_{x \to a}[f(x) - g(x)] = \lim_{x \to a}f(x) - \lim_{x \to a}g(x)$ 

属性3：

 Limit of product of two functions is the product of limits of both the functions. 

$\lim_{x \to a}[f(x).g(x)] = \lim_{x \to a}f(x). \lim_{x \to a}g(x)$ 

物业4：

 Limit of quotient of two functions is the quotient of limits of both the functions. 

$\lim_{x \to a}[\frac{f(x)}{g(x)}] = \frac{\lim_{x \to a}f(x)}{ \lim_{x \to a}g(x)}$ 

复合功能的极限

两个函数f(x)和g(x)的组成由(fog)(x)表示，这意味着函数g(x)的范围应位于函数f(x)的域中。现在，为了计算两个函数的组合的极限，我们使用以下属性：

$\lim_{x \to a}(f o g)(x) = \lim_{x \to a}f(g(x)) = f(\lim_{x \to a}g(x))$ 

让我们看一些关于这些概念的样本问题，

样本问题

问题1：给定函数f(x)= $\frac{1}{x^2}$ 。找$\lim_{x \to 0}\frac{1}{x^2}$ 。

解决方案：

Let’s see this limit graphically, 

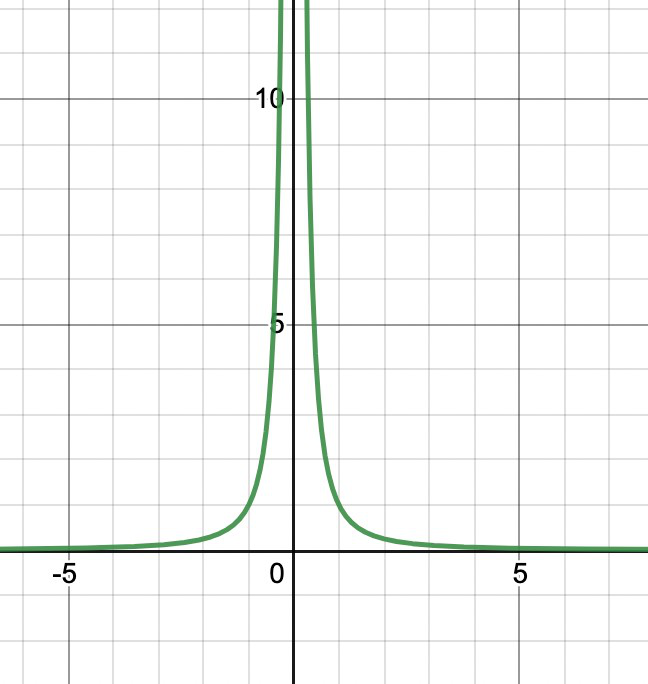
We can see from the graph while approaching the function from either of the sides towards zero. Values start going to infinity. 

$\lim_{x \to 0^-}f(x) = \lim_{x \to 0^+}f(x) = \infty$

问题2：当x⇢0时，求出函数f(x)= x + cos(x)的极限值。

解决方案：

The figure below shows the graph of the function, 

We know that f(x) is a combination of two different function. We can use the properties studied above, property 1 works for our case.

$\lim_{x \to a}[f(x) + g(x)] = \lim_{x \to a}f(x) + \lim_{x \to a}g(x)$

We know f(x) = x + cos(x). Let’s say h(x) = x and g(x) = cos(x) and using the above property we get. 

$\lim_{x \to 0}[h(x) + g(x)] = \lim_{x \to 0}h(x) + \lim_{x \to 0}g(x)$

= $\lim_{x \to 0}x + \lim_{x \to 0}cos(x)$

= $0 + 1$

= 1

问题3：找到函数f(x)的极限的值=(X ² + X 1)E ^X当x⇢0。

解决方案：

We know that f(x) is a combination of two different function. We can use the properties studied above, property 3 works for our case.

$\lim_{x \to a}[f(x).g(x)] = \lim_{x \to a}f(x). \lim_{x \to a}g(x)$

We know f(x) = (x² + x +1)e^x Let’s say h(x) = x² + x +1 and g(x) =e^x and using the above property we get. 

$\lim_{x \to 0}[h(x).g(x)] =( \lim_{x \to 0}h(x))(\lim_{x \to 0}g(x))$

= $\lim_{x \to 0}x^2 + x + 1 + \lim_{x \to 0}e^x$

= 1 + 1

= 2

问题4：求出函数f(x)的极限值= $\frac{cos(x)}{x^2 + x + 4}$当x⇢0时。

解决方案：

We know that f(x) is a combination of two different function. We can use the properties studied above, property 4 works for our case.

$\lim_{x \to a}[\frac{f(x)}{g(x)}] = \frac{\lim_{x \to a}f(x)}{ \lim_{x \to a}g(x)}$ 

We know f(x) = $\frac{cos(x)}{x^2 + x + 4}$ Let’s say g(x) = x² + x +4 and g(x) =cos(x) and using the above property we get. 

$\lim_{x \to a}[\frac{f(x)}{g(x)}] = \frac{\lim_{x \to a}f(x)}{ \lim_{x \to a}g(x)}$

= $\frac{\lim_{x \to 0}cos(x)}{\lim_{x \to 0}x^2 + x + 4}$

= $\frac{cos(0)}{0 + 0 + 4}$

= $\frac{1}{4}$

问题5：当x⇢0，f(x)=时，从左侧和右侧找到函数极限的值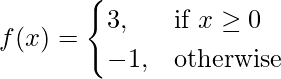$f(x)= \begin{cases} 3,& \text{if } x \geq 0\\ -1,& \text{otherwise} \end{cases}$ 。

解决方案：

Let’s see this limit graphically, 

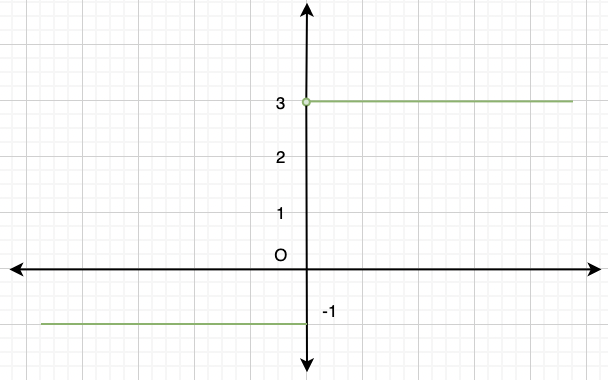
Notice in the graph that while approaching from the left-hand side, the functions seems to take value -1 and while approaching from the right-hand side, functions seems to taking value 3. 

Thus, 

$\lim_{x \to 0^-}f(x) = -1$

$\lim_{x \to 0^+}f(x) = 3$