指数函数和对数函数是在不同科学领域中广泛使用的一类函数。随着输入值的增加,指数函数迅速增加,对数函数趋于饱和。在下图中,可以注意到,随着x的幂增加,函数开始更快地增长,并且图表变得更陡峭。指数函数属于相似的函数类别。
下图表示对数和指数函数的图形。

指数函数的导数
让我们从查看指数函数,
y = e x
由于其区别,通常无法正常使用电力。因此,让我们使用极限来推导该值。使用极限的函数导数由下式给出:

现在这最后一个极限![]() 确切地是上面的导数f’(x)在x = 0时的定义,即f’(0)。因此,导数变为
确切地是上面的导数f’(x)在x = 0时的定义,即f’(0)。因此,导数变为
f’(x)= b x f’(0)= b x
因此,在自然指数函数的情况下,f(x)= e x
Note: In general exponential cases, for example, y = bx, where b is a real number. The derivative for this kind of function is
![]()
问题1:微分f(x)= 4e x – 5 x
回答:
The derivation of ex will remain ex, the derivative of 5x will become 5xln(5) as explained above.
Therefore, f'(x) = 4ex – 5xln(x)
问题2:差异化![]()
回答:
Here, following Quotient Rule, differentiate the function g(x):

问题3:当f(x)= 7 x + 2e x时,在x = 0处找到F’(x)的值
回答:
Differentiating: f'(x) = 7xln(7) + 2ex
at x=0, f'(0) = 70ln(7) + 2e0
= ln(7) + 2
= 3.945
对数函数的导数
现在,让我们看一下对数函数的导数是如何计算的。请注意,这些函数实际上是彼此相反的事实。
Note: If two functions are inverses of each other then,
![]()
众所周知的事实是自然指数和自然对数是彼此相反的。我们可以使用以上属性找到对数函数的导数。
假设f(x)= e x和g(x)= log e x。

让我们看一些有关这两个函数的导数的示例,
问题1:求微分:y(x)= x 5 – e x ln(x)
回答:
Differentiating using chain rule:
![Rendered by QuickLaTeX.com y'(x)= \frac{dy}{dx}x^5-[(\frac{dy}{dx}e^x)(ln(x))+(\frac{dy}{dx}ln(x))(e^x)]\\=5x^4-e^xln(x)-\frac{e^x}{x}](https://mangdo-1254073825.cos.ap-chengdu.myqcloud.com//front_eng_imgs/geeksforgeeks2021/Derivative%20of%20Exponential%20and%20Logarithmic%20Functions_8.jpg)
问题2:计算以下项的导数
(i)e -x (ii)sin(logx)(iii)e cos(x)
回答:
(i) Let y = e-x, to find the differentiation of this function we need to use chain rule.
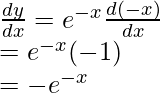
(ii) y = sin(logx). It also requires chain rule for differentiation.

(iii) y = ecos(x)
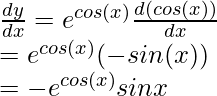
问题3:计算导数f(x)= 3e x + 10x 3 log(x)。
回答:
The derivative requires chain rule and the formulas studied above,
