📌  相关文章
📜  多边形面积–测定

📅  最后修改于: 2021-06-25 01:15:59             🧑  作者: Mango

测量是数学的一个分支，致力于研究不同的几何形状及其区域。它使用几何计算和代数方程来计算对象各个方面的度量。在几何中，多边形可以定义为具有直边的平面或平面二维封闭形状。它没有弯曲的侧面。

您能想到用直线封闭的二维形状吗?外观如何，如何定义此形状?我们将此形状称为多边形。要形成多边形，我们至少需要三个线段，因为多边形是封闭的形状。因此，最小的多边形是三角形，因为它具有三个边。

如果多边形具有4个边或线段，则称为四边形。如果多边形具有5个边或线段，则称为五角大楼。如果多边形具有6个边或线段，则称为六边形。如果多边形具有7个边或线段，则称为七边形，依此类推。

多边形部分

多边形的脸

面是指多边形的平面。多边形的名称可以通过其面孔来建议。

多边形的边缘

边是边界上将一个顶点(角点)连接到另一个顶点的线段。它们充当两个面的交点。面在直线的边缘相交。

边线-用于形成多边形的线段，用n表示。

内角-内角在多边形内部的相邻边之间形成并且彼此相等。内角的数量等于侧面均值的数量。例如，三角形具有3个内角，四边形具有4个内角，五角大楼具有5个内角，六边形具有6个内角。

外部角度-外部是通过扩展多边形的一侧而形成的，该扩展与相邻边之间的角度称为外部角度。

多边形顶点

两行或多行相交的点称为顶点。这是一个角落。边缘的交点表示顶点。这些边在点的顶点相交。

多边形是通过连接线而形成的，如果多边形具有三个边，则我们根据边的数量来命名多边形，如果多边形的边数为4，则称为三角形，然后称为四边形。

例如，三角形，四边形，五边形，六边形，七边形，八边形，九边形，十边形等。

凹面或凸面多边形

凸多边形没有指向内部的角度。所有内角的内角平均值始终小于180°。

如果任何内角大于180°，则多边形称为凹面。

几何中有两种类型的多边形：

1.正多边形

2.不规则多边形

正多边形

现在考虑具有相等边和相等角度的多边形。如何定义该多边形以及如何获取多边形的面积。由于该多边形的边和角度相等，因此我们可以将该多边形定义为规则多边形。规则的n边多边形具有n阶的旋转对称性。

正则多边形的所有顶点都位于一个共同的圆上，称为外接圆，因为它们是非循环点。正多边形是循环多边形。

正三角形-等边三角形

四边形–正方形

五角大楼–普通五角大楼

六角形-普通六角形

问题：正六边形的内外角是多少?

回答：

具有相等边和相等角度的多边形是规则多边形。

示例：如果多边形的周长为20 cm，Apothem为5 cm，则找到正多边形的面积。

解决方案：

不规则多边形

考虑一个具有不同角度和不同边的多边形。您无法找到此多边形的区域作为常规多边形。将这种类型的多边形定义为不规则多边形。

顶点–两行或多行相交的点称为顶点。边缘的交点表示顶点。

边线段，用于形成多边形，用n表示。这里在不规则多边形中每边的长度是不相等的。

外部角度-不规则多边形的外部角度之和也等于360°，但角度不相等。由于不规则多边形的内角测量值不同，因此每个外角的测量值也不同。

不规则多边形的面积

要查找不规则多边形的区域，请先将形状分成规则。然后，使用规则的多边形面积公式来查找每个多边形的面积。稍后添加所有规则多边形的面积以获得不规则多边形的面积。

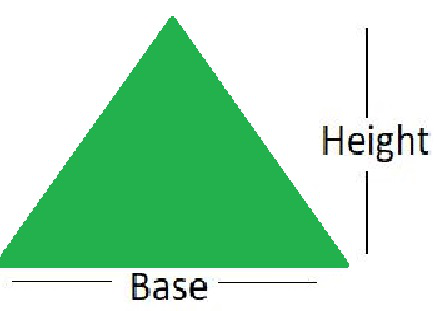
为了找到三角形的面积，我们使用以下公式：

三角形面积=(1/2)x底面x高度

要找到四边形的面积，我们可以通过构造对角线将四边形分为两个三角形，找到三角形的面积，然后将它们相加以找到给定四边形的面积。

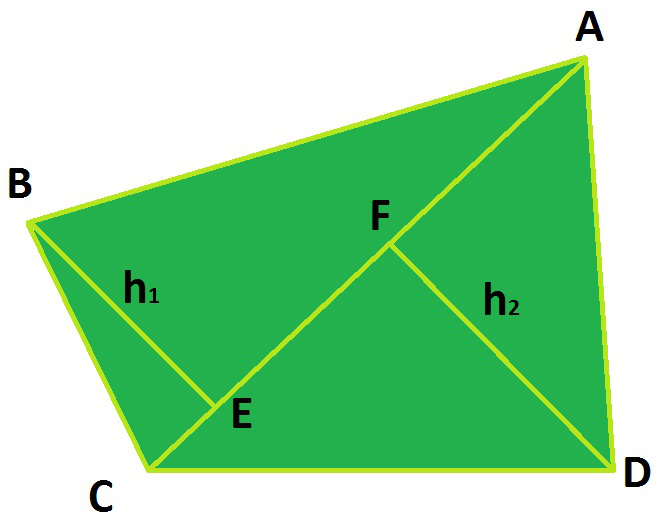
四边形的面积=(三角形的面积) 1 +(三角形的面积) 2

在上图中，对角AC将用作高度分别为h1和h2的两个三角形ABC和ADC的公共底。

同样，要找到五边形，六边形，七边形和八边形的面积或其他任何多边形的面积，我们会将多边形划分为不同的图形，然后将每个图形的面积相加，以计算该多边形的面积。

在这里，我们以一个五边形ABCDE为例，并将其分为四个部分来找到其面积。

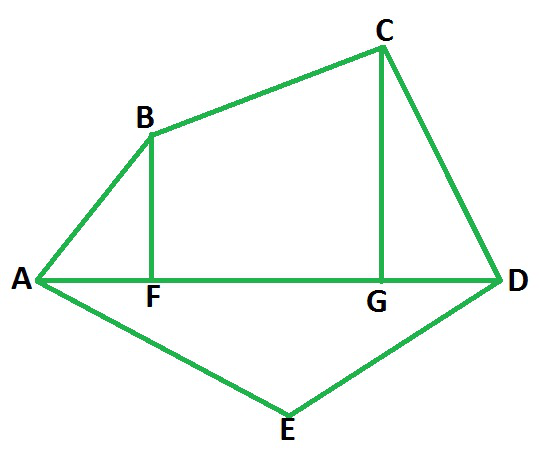
通过在其上构造一个对角线AD和两个垂直线BF和CG，将五边形ABCDE分为四个部分。所以，

类似地，我们可以通过将多边形划分为不同的部分来找到任何多边形的面积。

样本问题

现在，我们将做一些与查找多边形区域有关的问题，这将帮助我们以更好的方式清除该概念。

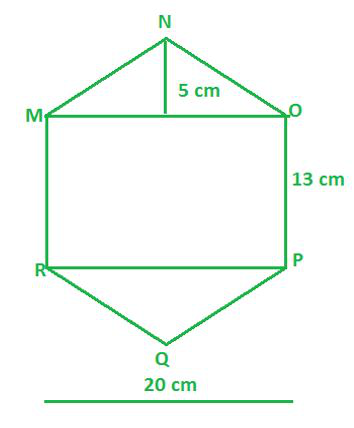
问题1：找到给定多边形的面积。

解决方案：

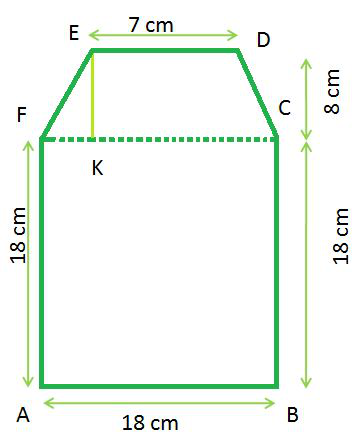
问题2：找到给定多边形的面积。

解决方案：

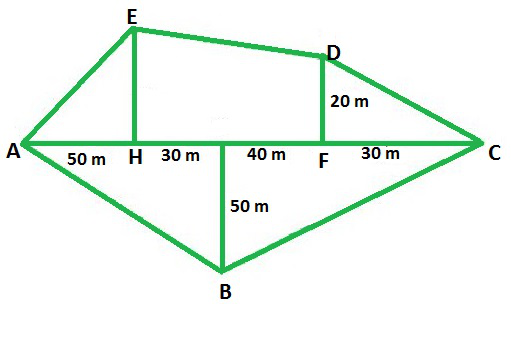
问题3：找到给定多边形的面积。

解决方案：

问题4：找到给定多边形的面积，对角AC的长度为18 cm。

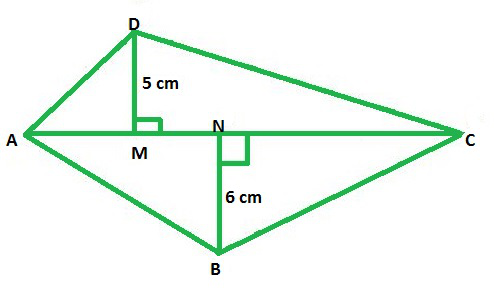
解决方案：

问题5：找到给定多边形的面积。

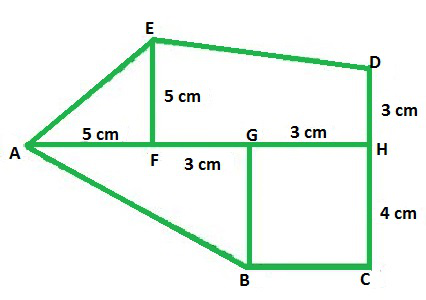
解决方案：

问题6：找到给定多边形的面积。

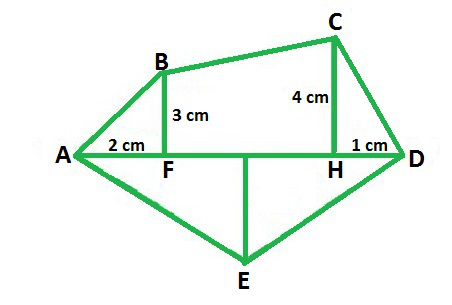
解决方案：