重力加速度

在跳伞过程中，当跳伞运动员从飞机上跳下来时，下降的速度突然增加，这是由于重力引起的加速度以及吸引人体的力(即重力)引起的。另外，当跳伞者打开降落伞时，降落伞会抵抗重力并降低速度，从而使人安全着陆。还观察到，当球沿向上方向运动时，其速度要比其下降时低。这完全是由于重力而产生的加速度。

地心引力

物体对地球的吸引力称为重力或重力。宇宙中的每个物体，无论大小，都会对其他物体施加力，因此，这种力称为万有引力。

例如：在地球表面上的任何两本书或任何两个物体之间作用的力。

此外，重力也被定义为以质量吸引两个物体的任何力。此外，这种引力之所以具有吸引力，是因为它总是试图将质量拉在一起，并且因为它是一种吸引力，所以它从未将它们推开。此外，包括您在内的每个物体都被整个宇宙中所有其他物体所吸引，这被称为牛顿万有引力定律或万有引力公式。

It is stated as:

$F_G=\dfrac{GMm}{d^2}$

where, F_G is the Gravitational constant, 

G is the Gravitational Constant \left(6.67\times10^{-11}\text{N}\cdot\text{m}^2/\text{kg}^2\right),

d is the distance between the two masses,

M and m are the masses of the two objects in contact.

The SI unit of the Gravitational force is Newton (N).

重力加速度

当物体掉落到地球上时，由于地球的重力，其加速度会发生变化。将该加速度称为重力引起的加速度。这是物体由于重力而获得的加速度。

由于重力(g)引起的加速度的SI单位为m /s² 。

由于重力引起的加速度具有大小和方向。因此，它是一个向量。

海平面在地球表面的标准值为9.8 m /s² 。

从数学上讲，由于重力而产生的加速度与物体的质量成正比，与与质心的距离成反比，因此给出：

$g\propto\dfrac{m}{r^2}$

或者

$g=\dfrac{Gm}{r^2}$

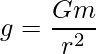
其中，G是引力常数，

m是身体的质量，

r是距中心的距离。

推导重力加速度公式

According to the second law of motion:

F = ma

But, in case of a free-falling body, the force is equal to the product of the mass of the body and acceleration due to gravity.

F = mg                                                                                                                   ……(1)

But, according to the universal law of gravitation:

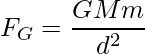$F_G=\dfrac{GMm}{d^2}$                                                                                         ……(2)

Now, from the equation (1) and (2),

$mg=\dfrac{Gm_1m_2}{d^2}$

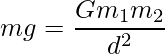
Consider for an ideal case the object is placed near to earth therefore the distance between earth and object will be radius of earth, so replace d with r and rearranging the above expression for g as:

$\begin{aligned}mg&=\dfrac{GMm}{r^2}\\g&=\dfrac{GM}{r^2}\end{aligned}$

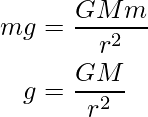
计算重力引起的加速度

The acceleration due to gravity is stated as:

$g=\dfrac{GM}{r^2}$

Here, substitute 6.67 × 10^-11 Nm² kg^-2 for G, 6 × 10²⁴ kg for M and 6.4 × 10⁶ m for r in the above expression to calculate g at the surface of Earth.

$\begin{aligned}g&=\dfrac{6.67\times10^{-11}\text{ N.m}^2/\text{ kg}^2\times6\times 10^{24}\text{ kg}}{\left(6.4\times10^6\text m\right)^2}\\&=9.8\text{ ms}^{-2}\end{aligned}$

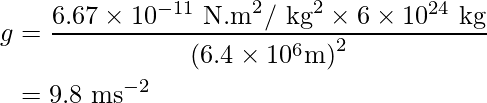
由于重力影响加速度的因素

• 地球的形状：众所周知，地球的形状不是球形，而是相当椭圆形的，因此重力在不同的地方会有所不同。由于地球的半径在极点处最小，因此在地球极处的吸引力最大，约为9.82 m / s ^2。由于地球的半径在赤道处最大，而地球赤道的引力最小，约为9.78 m / s ²

• 高度：当物体远离地球表面时，吸引力会随着地球与物体之间距离的增加而减小。
• 深度：将物体放入地球表面时，由于重力而产生的加速度变小。

深度D对g的影响

考虑质量为M的地球内部深度为D的质量为m的物体。

就密度而言，由于重力作用在地球表面产生的加速度为：

g = 4/3 xπρx RG

在深度D

g _D = 4/3 xπρx(RD)G

将两个方程相除，我们得到

g _d = gxπρx(RD)

Now two cases can be possible:

Case 1: If depth D is equal to the radius of the earth i.e. D = R, then:

g_d = 0

Case 2: If depth D = 0, i.e. the object is at the surface of earth, then

g_d = g

高度h对g的影响

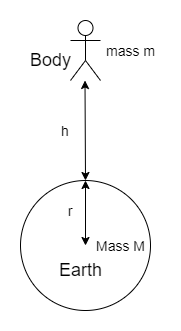
如果将物体放置在某个高度h，则d的值将变为(r + h)，因此，

g _h = GM /(r + h) ²

= GM / r ² (1 + h / r) ²

=(GM / r ² )/(1 + h / r) ²

= g /(1 + h / r) ² (因为g = GM / r ² )

当h远小于地球半径时，高度h处的g值由下式给出：

g _h = g /(1 – 2h / r)

样本问题

这是基于上面讨论的主题而解决的一些问题。

Problem 1: A body is placed at a height of 2 x 10⁶ m from the earth’s surface having a mass of 20 kg. Find the acceleration due to the gravity of the body?

Solution:

Given that,

The height of the body, h is 2 x 10⁶ m.

The mass of the body, m is 20 kg.

By the formula for g at a height h is:

g_h = g / (1+h/r)²

Here, g is the acceleration due to gravity, h is the height and r is the radius of the Earth.

Substitute 2 x 10⁶ m for h, 9.8 ms^-2 for g and 6.4 x 10⁶ m for r in the above expression to calculate g_h.

g_h = 9.8 /(1+(2×10⁶ / 6×10⁶))

     = 7.35 ms^-2 

Hence, the acceleration due to the gravity of the body at height h is 7.35 ms^-2.

Problem 2: A body of mass m is placed on the earth and then on the moon. Find the ratio of acceleration due to gravity on earth w.r.t. to the moon? (mass of earth = 5.98 x 10²⁴ Kg, mass of moon = 7.36 x 10²² Kg, radius of earth = 6.37 x 10⁶ m, radius of moon = 1.74 x 10⁶ m).

Solution:  

The acceleration due to gravity is:

g=GM/r²

Here, G is the Gradational constant. M is the mass and r is the radius of the Earth.

The acceleration due to gravity on Earth is:

g_e = G x M_e/r_e²                                                                                                         ……(1)

The acceleration due to gravity on Moon is:

g_m = G x M_m/r_m²                                                                                                      ……(2)

Divide equation (1) by equation (2) as:

g_e/g_m = (M_e x r_m²)/(M_m x r_e²)

Substitute the given values in the above expression, to calculate the ratio of acceleration due to gravity on earth w.r.t. to the moon. 

g_e/g_m = 6.062 ≈ 6

Hence, the ratio of acceleration due to gravity on earth w.r.t. to the moon is equal to 6:1.

Problem 3: A body is placed inside the earth at a depth d=1.5 x 10⁶ m. Find the acceleration due to the gravity of the body? Take the density of earth 5515 kg/m³.

Solution: 

The acceleration due to gravity in terms of density is:

g=4/3 x πρ x RG

Here, ρ is the density, R is the radius and G is the gravitational constant.

And, at depth d is:

g_d = 4/3 x πρ x (R-d)G

     = 4/3 x 5515 x (6 x 10⁶ – 1.5 x 10⁶) x 6.67 x 10^-11

     = 0.206 m/s² 

Hence, the acceleration due to the gravity of the body is equal to 0.206 m/s².

Problem 4: What will be the decrease in the value of g as a body moves a distance of R/2 above the earth’s surface?

Solution: 

The acceleration due to gravity at a height h is:

g_h = g / (1+h/R)²

Now, it is given that: h = R/2.

Therefore, the above expression becomes:

 g_h = g/(1+R/2R)²

     = g/ (3/2)²

     = 4/9 x g

Hence, the values of g decreases by 4/9 times.

Problem 5: A planet has a radius and mass are half those of the earth. Find the acceleration due to gravity on the planet?

Solution: 

Given that,

The radius of the planet, r_p = 2r.

The mass of the planet, M_p = 2M

The formula to calculate the acceleration due to gravity of the planet,

g_p = GM_p / r_p²

    = (2GM) / (2r)²

    = (1/2)  GM/r

    = 1/2 x g                        (Since, g=GM/r² )      

Hence, the acceleration due to gravity on the planet is half times the acceleration due to gravity on earth.