先决条件:深度优先搜索|父阵列表示
给定二叉树的父数组表示形式,我们需要找到二叉树中等腰三角形的数量。
考虑一个代表二叉树的父数组:
父数组: 
下面给出的是给定父数组的树表示形式。
二叉树: 
在二叉树中可以找到三种等腰三角形。这三种不同类型的等腰三角形可以作为三种不同的情况来处理。
情况1: Apex(与基本共享平等边的顶点)具有两个后继(直接/间接)。
这种情况可以表示为: 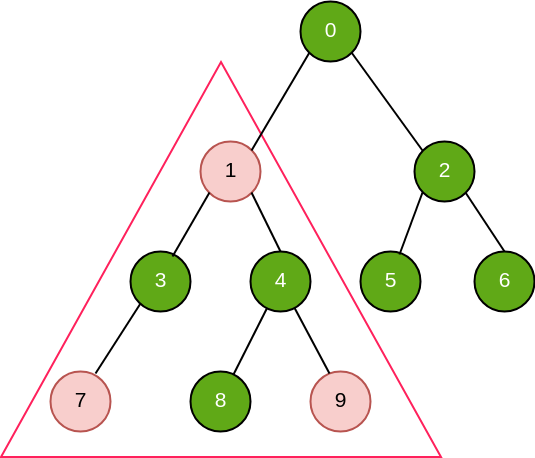
在给定的树中,有6个这样的等腰三角形,即; (0、1、2),(0、3、6),(1、3、4),(1、7、9),(4、8、9),(2、5、6)
Pseudo Code: count += min(left_down[i], right_down[i]);情况2: Apex具有左继任者(直接/间接),而顶点本身是其父代的右继任者(直接/间接)。
这种情况可以表示为: 
在给定的树中,有2个这样的等腰三角形,即; (1、8、4),(0、5、2)
Pseudo Code: count += min(left_down[i], left_up[i]);情况3: Apex具有右继任者(直接/间接),而顶点本身是其父代的左继任者(直接/间接)。
这种情况可以表示为: 
在给定的树中,有1个这样的等腰三角形,即; (0,1,4)
Pseudo Code: count += min(right_down[i], right_up[i]);Pseudo Code legend:
left_down[i] -> maximum distance of ith node from its farthest left successor
right_down[i] -> maximum distance of ith node from its farthest right successor
left_up[i] -> maximum distance of ith node from its farthest left predecessor
right_up[i] -> maximum distance of ith node from its farthest right predecessor
以下是计算给定二叉树中存在的等腰三角形数量的实现:
C++
/* C++ program for calculating number of
isosceles triangles present in a binary tree */
#include
using namespace std;
#define MAX_SZ int(1e5)
/* Data Structure used to store
Binary Tree in form of Graph */
vector* graph;
// Data varirables
int right_down[MAX_SZ];
int left_down[MAX_SZ];
int right_up[MAX_SZ];
int left_up[MAX_SZ];
/* Utility function used to
start a DFS traversal over a node */
void DFS(int u, int* parent)
{
if (graph[u].size() != 0)
sort(graph[u].begin(), graph[u].end());
if (parent[u] != -1) {
if (graph[parent[u]].size() > 1) {
/* check if current node is
left child of its parent */
if (u == graph[parent[u]][0]) {
right_up[u] += right_up[parent[u]] + 1;
}
// current node is right child of its parent
else {
left_up[u] += left_up[parent[u]] + 1;
}
}
/* check if current node is left and
only child of its parent */
else {
right_up[u] += right_up[parent[u]] + 1;
}
}
for (int i = 0; i < graph[u].size(); ++i) {
int v = graph[u][i];
// iterating over subtree
DFS(v, parent);
// left child of current node
if (i == 0) {
left_down[u] += left_down[v] + 1;
}
// right child of current node
else {
right_down[u] += right_down[v] + 1;
}
}
}
/* utility function used to generate
graph from parent array */
int generateGraph(int* parent, int n)
{
int root;
graph = new vector[n];
// Generating graph from parent array
for (int i = 0; i < n; ++i) {
// check for non-root node
if (parent[i] != -1) {
/* creating an edge from node with number
parent[i] to node with number i */
graph[parent[i]].push_back(i);
}
// initializing root
else {
root = i;
}
// Initializing necessary data variables
left_up[i] = 0;
right_up[i] = 0;
left_down[i] = 0;
right_down[i] = 0;
}
// root of the binary tree
return root;
}
// Driver Function
int main()
{
int n = 10;
/* Parent array used for storing
parent of each node */
int parent[] = { -1, 0, 0, 1, 1, 2, 2, 3, 4, 4 };
/* generateGraph() function generates a graph a
returns root of the graph which can be used for
starting DFS traversal */
int root = generateGraph(parent, n);
// triggering dfs for traversal over graph
DFS(root, parent);
int count = 0;
// Calculation of number of isosceles triangles
for (int i = 0; i < n; ++i) {
count += min(right_down[i], right_up[i]);
count += min(left_down[i], left_up[i]);
count += min(left_down[i], right_down[i]);
}
cout << "Number of isosceles triangles "
<< "in the given binary tree are " << count;
return 0;
} Java
/* JAVA program for calculating number of
isosceles triangles present in a binary tree */
import java.io.*;
import java.util.*;
@SuppressWarnings("unchecked")
class Isosceles_triangles {
static int MAX_SZ = (int)1e5;
/* Data Structure used to store
Binary Tree in form of Graph */
static ArrayList[] graph;
// Data varirables
static int[] right_down = new int[MAX_SZ];
static int[] left_down = new int[MAX_SZ];
static int[] right_up = new int[MAX_SZ];
static int[] left_up = new int[MAX_SZ];
/* Utility function used to
start a DFS traversal over a node */
public static void DFS(int u, int[] parent)
{
if (graph[u] != null)
Collections.sort(graph[u]);
if (parent[u] != -1) {
if (graph[parent[u]].size() > 1) {
/* check if current node is
left child of its parent */
if (u == graph[parent[u]].get(0)) {
right_up[u] += right_up[parent[u]] + 1;
}
// current node is right child of its parent
else {
left_up[u] += left_up[parent[u]] + 1;
}
}
/* check if current node is left and
only child of its parent */
else {
right_up[u] += right_up[parent[u]] + 1;
}
}
if (graph[u] == null)
return;
for (int i = 0; i < graph[u].size(); ++i) {
int v = graph[u].get(i);
// iterating over subtree
DFS(v, parent);
// left child of current node
if (i == 0) {
left_down[u] += left_down[v] + 1;
}
// right child of current node
else {
right_down[u] += right_down[v] + 1;
}
}
}
static int min(Integer a, Integer b)
{
return (a < b) ? a : b;
}
/* utility function used to generate
graph from parent array */
public static int generateGraph(int[] parent, int n)
{
int root = -1;
graph = (ArrayList[]) new ArrayList[n];
// Generating graph from parent array
for (int i = 0; i < n; ++i) {
// check for non-root node
if (parent[i] != -1) {
/* creating an edge from node with number
parent[i] to node with number i */
if (graph[parent[i]] == null) {
graph[parent[i]] = new ArrayList();
}
graph[parent[i]].add(i);
// System.out.println(graph);
}
// initializing root
else {
root = i;
}
// Initializing necessary data variables
left_up[i] = 0;
right_up[i] = 0;
left_down[i] = 0;
right_down[i] = 0;
}
// root of the binary tree
return root;
}
// Driver Function
public static void main(String[] args)
{
int n = 10;
/* Parent array used for storing
parent of each node */
int[] parent = new int[] { -1, 0, 0, 1, 1, 2, 2, 3, 4, 4 };
/* generateGraph() function generates a graph a
returns root of the graph which can be used for
starting DFS traversal */
int root = generateGraph(parent, n);
// System.exit(0);
// triggering dfs for traversal over graph
DFS(root, parent);
int count = 0;
// Calculation of number of isosceles triangles
for (int i = 0; i < n; ++i) {
count += min(right_down[i], right_up[i]);
count += min(left_down[i], left_up[i]);
count += min(left_down[i], right_down[i]);
}
System.out.println("Number of isosceles triangles "
+ "in the given binary tree are "
+ Integer.toString(count));
System.exit(0);
}
} Python3
''' Python3 program for calculating number of
isosceles triangles present in a binary tree '''
MAX_SZ = int(1e5)
''' Data Structure used to store
Binary Tree in form of Graph '''
graph = {}
# Data varirables
right_down = MAX_SZ*[0]
left_down = MAX_SZ*[0]
right_up = MAX_SZ*[0]
left_up = MAX_SZ*[0]
''' Utility function used to
start a DFS traversal over a node '''
def DFS(u, parent):
if u in graph:
graph[u].sort()
if parent[u] != -1:
if u in graph and len(graph[parent[u]]) > 1:
''' check if current node is
left child of its parent '''
if u == graph[parent[u]][0] :
right_up[u] += right_up[parent[u]] + 1
# current node is right child of its parent
else:
left_up[u] += left_up[parent[u]] + 1
else :
''' check if current node is left and
only child of its parent '''
right_up[u] += right_up[parent[u]] + 1
if u in graph:
for i in range(0, len(graph[u])):
v = graph[u][i]
# iterating over subtree
DFS(v, parent)
# left child of current node
if i == 0:
left_down[u] += left_down[v] + 1;
# right child of current node
else:
right_down[u] += right_down[v] + 1;
''' utility function used to generate
graph from parent array '''
def generateGraph(parent, n):
root = -1
# Generating graph from parent array
for i in range(0, n):
# check for non-root node
if parent[i] != -1:
''' creating an edge from node with number
parent[i] to node with number i '''
if parent[i] not in graph:
graph[parent[i]] = [i]
else :
graph[parent[i]].append(i)
# initializing root
else :
root = i
# root of the binary tree
return root;
# Driver Function
if __name__ == '__main__':
n = 10
''' Parent array used for storing
parent of each node '''
parent = [-1, 0, 0, 1, 1, 2, 2, 3, 4, 4]
''' generateGraph() function generates a graph a
returns root of the graph which can be used for
starting DFS traversal '''
root = generateGraph(parent, n)
# triggering dfs for traversal over graph
DFS(root, parent)
count = 0
# Calculation of number of isosceles triangles
for i in range(0, n):
count += min(right_down[i], right_up[i])
count += min(left_down[i], left_up[i])
count += min(left_down[i], right_down[i])
print("Number of isosceles triangles "
+ "in the given binary tree are "
+ str(count))C#
/* C# program for calculating number of
isosceles triangles present in a binary tree */
using System;
using System.Collections.Generic;
using System.Linq;
class Isosceles_triangles
{
static int MAX_SZ = (int)1e5;
/* Data Structure used to store
Binary Tree in form of Graph */
static List[] graph;
// Data varirables
static int[] right_down = new int[MAX_SZ];
static int[] left_down = new int[MAX_SZ];
static int[] right_up = new int[MAX_SZ];
static int[] left_up = new int[MAX_SZ];
/* Utility function used to
start a DFS traversal over a node */
public static void DFS(int u, int[] parent)
{
if (graph[u] != null)
graph[u].Sort();
if (parent[u] != -1)
{
if (graph[parent[u]].Count > 1)
{
/* check if current node is
left child of its parent */
if (u == graph[parent[u]][0])
{
right_up[u] += right_up[parent[u]] + 1;
}
// current node is right child of its parent
else
{
left_up[u] += left_up[parent[u]] + 1;
}
}
/* check if current node is left and
only child of its parent */
else
{
right_up[u] += right_up[parent[u]] + 1;
}
}
if (graph[u] == null)
return;
for (int i = 0; i < graph[u].Count; ++i)
{
int v = graph[u][i];
// iterating over subtree
DFS(v, parent);
// left child of current node
if (i == 0)
{
left_down[u] += left_down[v] + 1;
}
// right child of current node
else
{
right_down[u] += right_down[v] + 1;
}
}
}
static int min(int a, int b)
{
return (a < b) ? a : b;
}
/* utility function used to generate
graph from parent array */
public static int generateGraph(int[] parent, int n)
{
int root = -1;
graph = new List[n];
// Generating graph from parent array
for (int i = 0; i < n; ++i)
{
// check for non-root node
if (parent[i] != -1)
{
/* creating an edge from node with number
parent[i] to node with number i */
if (graph[parent[i]] == null)
{
graph[parent[i]] = new List();
}
graph[parent[i]].Add(i);
// Console.WriteLine(graph);
}
// initializing root
else
{
root = i;
}
// Initializing necessary data variables
left_up[i] = 0;
right_up[i] = 0;
left_down[i] = 0;
right_down[i] = 0;
}
// root of the binary tree
return root;
}
// Driver Function
public static void Main(String[] args)
{
int n = 10;
/* Parent array used for storing
parent of each node */
int[] parent = new int[] { -1, 0, 0, 1, 1, 2, 2, 3, 4, 4 };
/* generateGraph() function generates a graph a
returns root of the graph which can be used for
starting DFS traversal */
int root = generateGraph(parent, n);
// System.exit(0);
// triggering dfs for traversal over graph
DFS(root, parent);
int count = 0;
// Calculation of number of isosceles triangles
for (int i = 0; i < n; ++i)
{
count += min(right_down[i], right_up[i]);
count += min(left_down[i], left_up[i]);
count += min(left_down[i], right_down[i]);
}
Console.WriteLine("Number of isosceles triangles "
+ "in the given binary tree are "
+ count);
}
}
// This code is contributed by Rajput-Ji Number of isosceles triangles in the given binary tree are 9
时间复杂度: O(n)
辅助空间: O(n)
如果您希望与行业专家一起参加现场课程,请参阅《 Geeks现场课程》和《 Geeks现场课程美国》。