等腰三角形面积

几何和几何形式在数学和日常生活中一直很重要。从一个小盘子到一座大摩天大楼，我们总是被各种形式和大小的几何图形所包围。必须估计这些形状所占据的形状和表面积，以便轻松存储它们或满足使用它们的人的需求。在数学学科中，这种研究或计算被赋予了特定的名称。

什么是测量？

测量是计算各种几何形式的尺寸并测量其面积或数学能力的过程。测量也可用于根据其表面积和容量确定各种形式的尺寸。

三角形

三角形是通过联合三个点产生的二维形状，其中两个点是共线的。顾名思义，这种形式具有三个角度，总和始终为 180°。术语“Tri”是指包含形状的三个顶点、边和角。几何上写成“△”。 △ABC是下图中的一个三角形，B点和C点共线。 △ABC显然有AB、BC、CA三个边，还有∠ABC、∠BCA、∠CAB三个角。

三角形的类型

三角形分为四种类型：

• 不等边三角形：不等边三角形是一个三角形，其角度大小不同，所有三个边的长度都不同。
• 等腰三角形：等腰三角形定义为两条边等长的三角形。
• 等边三角形：等边三角形是所有边的长度相等且所有角的尺寸都相等的三角形。
• 直角三角形：直角三角形是90°角的三角形。这种类型的三角形有三个边，其中最长的是斜边，它与 90 度角相对。

等腰三角形

等腰三角形是具有两条相等边的三角形。与两条相等边相对的两个角也相等。换句话说，“等腰三角形是具有两条全等边的三角形”。

假设在三角形△ABC中，如果边AB和AC相等，则ABC是∠B=∠C的等腰三角形。等腰三角形由定理描述：“如果三角形的两条边全等，则它们的对角同样全等”。

等腰三角形面积

If the lengths of an isosceles triangle’s equal sides and base are known, the triangle’s height or altitude may be computed. The formula for calculating the area of an isosceles triangle with sides is as follows:

Isosceles triangle area = 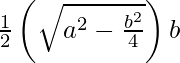$\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

where,

b = the isosceles triangle’s base

a = the length of two equal sides

公式的推导

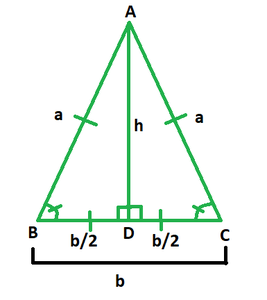
From the above figure, we have,

AB = AC = a (sides of equal length)

BD = DC = ½ BC = ½ b (Perpendicular from the vertex angle ∠A bisects the base BC)

Using Pythagoras theorem on ΔABD,

a² = (b/2)² + (AD)²

AD = $\sqrt{a^2 - \frac{b^2}{4}}$

The altitude of an isosceles triangle = $\sqrt{a^2 - \frac{b^2}{4}}$

It is known that the general formula of area of the triangle is, Area = ½ × b × h

Substituting value for height, we get

Area of isosceles triangle = 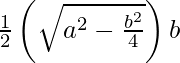$\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

类似问题

问题 1. 求一个等腰三角形的面积，它的等边长为13 厘米，底边为24 厘米。

解决方案：

We have, a = 13 and b = 24.

Area of isosceles triangle is given by, 

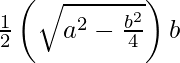
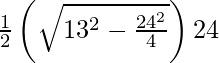
A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{13^2 - \frac{24^2}{4}}\right) × 24$

= 1/2 × 5 × 24

= 60 cm²

问题 2. 求一个等腰三角形的面积，其等边长为10 厘米，底边为 12 厘米。

解决方案：

We have, a = 10 and b = 12.

Area of isosceles triangle is given by,

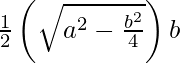
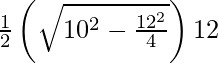
A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{10^2 - \frac{12^2}{4}}\right) × 12$

= 1/2 × 8 × 12

= 48 cm²

问题 3. 求等腰三角形的面积，其等边长为5 厘米，底边为6 厘米。

解决方案：

We have, a = 5 and b = 6.

Area of isosceles triangle is given by,

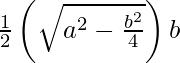
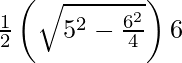
A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{5^2 - \frac{6^2}{4}}\right) × 6$

= 1/2 × 4 × 6

= 12 cm²

问题 4. 求一个等腰三角形的面积，它的等边长为15 厘米，底边为24 厘米。

解决方案：

We have, a = 15 and b = 24.

Area of isosceles triangle is given by,

A = 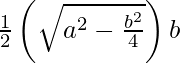$\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{15^2 - \frac{24^2}{4}}\right) × 24$

= 1/2 × 9 × 24

= 108 cm²

问题 5. 求一个等腰三角形的面积，它的等边长为17 厘米，底边为 30 厘米。

解决方案：

We have, a = 17 and b = 30.

Area of isosceles triangle is given by,

A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{17^2 - \frac{30^2}{4}}\right) × 30$

= 1/2 × 8 × 30

= 120 cm²

问题 6. 求等腰三角形的面积，其等边长为20 厘米，底边为 24 厘米。

解决方案：

We have, a = 20 and b = 24.

Area of isosceles triangle is given by,

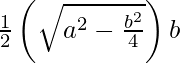
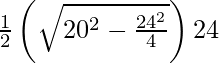
A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{20^2 - \frac{24^2}{4}}\right) × 24$

= 1/2 × 16 × 24

= 192 cm²

问题 7. 求一个等腰三角形的面积，它的等边长为25 厘米，底边为30 厘米。

解决方案：

We have, a = 25 and b = 30.

Area of isosceles triangle is given by,

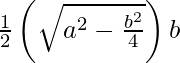
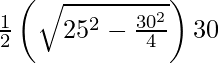
A = $\frac{1}{2} ×\left(\sqrt{a^2 - \frac{b^2}{4}}\right) × b$

= $\frac{1}{2} ×\left(\sqrt{25^2 - \frac{30^2}{4}}\right) × 30$

= 1/2 × 20 × 30

= 300 cm²