超平面，子空间和半空间

1.超飞机：
在几何上，超平面是尺寸小于其周围空间尺寸的几何实体。

这是什么意思?
这意味着以下内容。例如，如果您使用3D空间，则超平面是一个无量纲1的几何实体。因此它将是2维，而3D空间中的2维实体将是一个平面。现在，如果您采用2维，则1维无量纲将是一维几何实体，这将是一条线，依此类推。

• 通常用以下等式描述超平面

  X ^T n + b = 0

• 如果我们将其扩展为n个变量，我们将得到类似这样的结果

  X ₁ n ₁ + X ₂ n ₂ + X ₃ n ₃ +……….. + X _n n _n + b = 0

• 在仅二维中，我们将得到类似这样的东西，它只是直线的方程式。

  X ₁ n ₁ + X ₂ n ₂ + b = 0

例子：

让我们考虑一个2D几何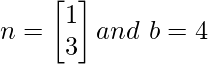$n = \begin{bmatrix} 1\\ 3\\ \end{bmatrix} and\ b = 4$虽然它是2D几何图形，但X的值为$X = \begin{bmatrix} x_1\\ x_2\\ \end{bmatrix}$因此，根据超平面方程可以将其求解为$X^Tn + b = 0\\ \begin{bmatrix} x_1 x_2 \end{bmatrix} \begin{bmatrix} 1\\ 3\\ \end{bmatrix} + 4 = 0\\ x_1 + 3x_2 + 4 = 0$因此，从解决方案中您可以看到，超平面是一条直线的方程。

2.子空间：
通常，超平面不是子空间。但是，如果我们有以下形式的超平面，

X ^T n = 0

也就是说，如果平面经过原点，那么超平面也将成为子空间。

3.半角空格：
考虑下面给出的二维图片。

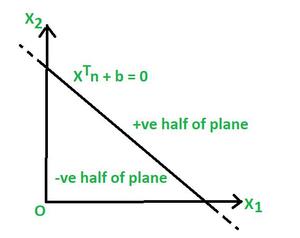
因此，这里我们在X ₁和X _2中有一个二维空间，并且如我们之前所讨论的，二维方程将是一条线，该线将是一个超平面。因此，直线的等式写为

X ^T n + b = 0

因此，对于这两个维度，我们可以像之前讨论的那样写这行代码

X ₁ n ₁ + X ₂ n ₂ + b = 0

您可以从上图看到，整个二维空间被分成两个空间。一条在直线的这一侧(平面的一半)，另一条在直线的这一侧(平面的一半)。现在，这两个空间称为半空间。

例子：

Let’s consider the same example that we have taken in hyperplane case. So by solving, we got the equation as 

x₁ + 3x₂ + 4 = 0

There may arise 3 cases. Let’s discuss each case with an example.

Case 1:

x₁ + 3x₂ + 4 = 0 : On the line

Let consider two points (-1,-1). When we put this value on the equation of line we got 0. So we can say that this point is on the hyperplane of the line. 

Case 2: 
Similarly, 

x₁ + 3x₂ + 4 > 0 : Positive half-space

Consider two points (1,-1). When we put this value on the equation of line we got 2 which is greater than 0. So we can say that this point is on the positive half space. 
Case 3: 

x₁ + 3x₂ + 4 < 0 : Negative half-space

Consider two points (1,-2). When we put this value on the equation of line we got -1 which is less than 0. So we can say that this point is on the negative half-space.