使用 BFS 按字典顺序遍历图

给定一个图， G由N 个节点、一个源S和一个类型为{u, v}的数组Edges[][2]组成，该数组表示节点u和v之间存在无向边，任务是遍历使用 BFS 按字典顺序绘制图形。

Input: N = 10, M = 10, S = ‘a’, Edges[][2] = { { ‘a’, ‘y’ }, { ‘a’, ‘z’ }, { ‘a’, ‘p’ }, { ‘p’, ‘c’ }, { ‘p’, ‘b’ }, { ‘y’, ‘m’ }, { ‘y’, ‘l’ }, { ‘z’, ‘h’ }, { ‘z’, ‘g’ }, { ‘z’, ‘i’ } } 
Output: a p y z b c l m g h i

Explanation: 
For the first level visit the node in lexicographical order as shown and print it: 
 

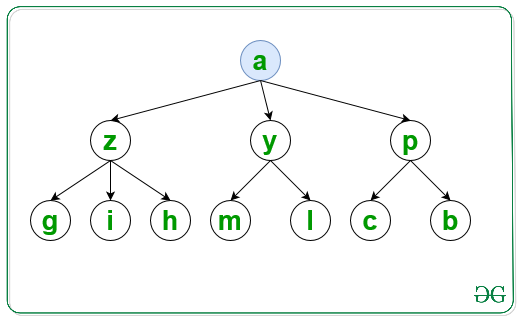
For the second level visit the node in lexicographical order as shown and print it: 
 

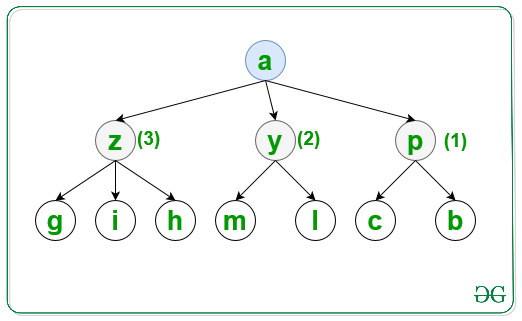
For the third level visit the node in lexicographical order as shown and print it: 
 

 

Input: N = 6, S = ‘a’, Edges[][2] = { { ‘a’, ‘e’ }, { ‘a’, ‘d’ }, { ‘e’, ‘b’ }, { ‘e’, ‘c’ }, { ‘d’, ‘f’ }, { ‘d’, ‘g’ } } 
Output: a d e f g b c

 

处理方法：按照以下步骤解决问题：

• 初始化一个映射，比如G以根据节点的字典顺序存储一个节点的所有相邻节点。
• 初始化一个地图，比如vis检查一个节点是否已经被遍历。
• 遍历 Edges[][2] 数组，并将图的每个节点的所有相邻节点存储在G 中。
• 最后，使用 BFS 遍历图形并打印图形的访问节点。

下面是上述方法的实现：

C++

// C++ program to implement
// the above approach
 
#include 
using namespace std;
 
// Function to traverse the graph in
// lexicographical order using BFS
void LexiBFS(map >& G,
             char S, map& vis)
{
    // Stores nodes of the graph
    // at each level
    queue q;
 
    // Insert nodes of first level
    q.push(S);
 
    // Mark S as
    // visited node
    vis[S] = true;
 
    // Traverse all nodes of the graph
    while (!q.empty()) {
 
        // Stores top node of queue
        char top = q.front();
 
        // Print visited nodes of graph
        cout << top << " ";
 
        // Insert all adjacent nodes
        // of the graph into queue
        for (auto i = G[top].begin();
             i != G[top].end(); i++) {
 
            // If i is not visited
            if (!vis[*i]) {
 
                // Mark i as visited node
                vis[*i] = true;
 
                // Insert i into queue
                q.push(*i);
            }
        }
 
        // Pop top element of the queue
        q.pop();
    }
}
 
// Utility Function to traverse graph
// in lexicographical order of nodes
void CreateGraph(int N, int M, int S,
                 char Edges[][2])
{
    // Store all the adjacent nodes
    // of each node of a graph
    map > G;
 
    // Traverse Edges[][2] array
    for (int i = 0; i < M; i++) {
        G[Edges[i][0]].insert(Edges[i][1]);
    }
 
    // Check if a node is already visited or not
    map vis;
 
    LexiBFS(G, S, vis);
}
 
// Driver Code
int main()
{
    int N = 10, M = 10 S = 'a';
    char Edges[M][2]
        = { { 'a', 'y' }, { 'a', 'z' },
            { 'a', 'p' }, { 'p', 'c' },
            { 'p', 'b' }, { 'y', 'm' },
            { 'y', 'l' }, { 'z', 'h' },
            { 'z', 'g' }, { 'z', 'i' } };
 
    // Function Call
    CreateGraph(N, M, S, Edges);
 
    return 0;
}

Java

// Java program to implement
// the above approach
import java.util.*;
 
class Graph{
 
// Function to traverse the graph in
// lexicographical order using BFS
static void LexiBFS(HashMap> G,
            char S, HashMap vis)
{
     
    // Stores nodes of the graph
    // at each level
    Queue q = new LinkedList<>();
 
    // Insert nodes of first level
    q.add(S);
 
    // Mark S as
    // visited node
    vis.put(S, true);
 
    // Traverse all nodes of the graph
    while (!q.isEmpty())
    {
         
        // Stores top node of queue
        char top = q.peek();
 
        // Print visited nodes of graph
        System.out.print(top + " ");
 
        // Insert all adjacent nodes
        // of the graph into queue
        if (G.containsKey(top))
        {
            for(char  i : G.get(top))
            {
                 
                // If i is not visited
                if (vis.containsKey(i))
                {
                    if (!vis.get(i))
                    {
                         
                        // Mark i as visited node
                        vis.put(i, true);
 
                        // Insert i into queue
                        q.add(i);
                    }
                }
                else
                {
                     
                    // Mark i as visited node
                    vis.put(i, true);
 
                    // Insert i into queue
                    q.add(i);
                }
            }
        }
 
        // Pop top element of the queue
        q.remove();
    }
}
 
// Utility Function to traverse graph
// in lexicographical order of nodes
static void CreateGraph(int N, int M, char S,
                        char[][] Edges)
{
     
    // Store all the adjacent nodes
    // of each node of a graph
    HashMap> G = new HashMap<>();
 
    // Traverse Edges[][2] array
    for(int i = 0; i < M; i++)
    {
        if (G.containsKey(Edges[i][0]))
        {
            Set temp = G.get(Edges[i][0]);
            temp.add(Edges[i][1]);
            G.put(Edges[i][0], temp);
        }
        else
        {
            Set temp = new HashSet<>();
            temp.add(Edges[i][1]);
            G.put(Edges[i][0], temp);
        }
    }
     
    // Check if a node is already visited or not
    HashMap vis = new HashMap<>();
 
    LexiBFS(G, S, vis);
}
 
// Driver code
public static void main(String[] args)
{
    int N = 10, M = 10;
    char S = 'a';
     
    char[][] Edges = { { 'a', 'y' }, { 'a', 'z' },
                       { 'a', 'p' }, { 'p', 'c' },
                       { 'p', 'b' }, { 'y', 'm' },
                       { 'y', 'l' }, { 'z', 'h' },
                       { 'z', 'g' }, { 'z', 'i' } };
 
    // Function Call
    CreateGraph(N, M, S, Edges);
}
}
 
// This code is contributed by hritikrommie

Python3

# Python3 program to implement
# the above approach
from collections import deque
 
G = [[] for i in range(1000)]
vis = [False for i in range(1000)]
 
# Function to traverse the graph in
# lexicographical order using BFS
def LexiBFS(S):
     
    global G, vis
     
    # Stores nodes of the graph
    # at each level
    q = deque()
     
    # Insert nodes of first level
    q.append(ord(S))
 
    # Mark S as
    # visited node
    vis[ord(S)] = True
    #a = []
 
    # Traverse all nodes of the graph
    while (len(q) > 0):
         
        # Stores top node of queue
        top = q.popleft()
        print(chr(top), end = " ")
 
        # Insert all adjacent nodes
        # of the graph into queue
        for i in G[top]:
             
            # If i is not visited
            if (not vis[i]):
                #print(chr(i),end=" ")
 
                # Mark i as visited node
                vis[i] = True
 
                # Insert i into queue
                q.append(i)
 
# Utility Function to traverse graph
# in lexicographical order of nodes
def CreateGraph(N, M, S,Edges):
 
    # Traverse Edges[][2] array
    for i in range(M):
        G[ord(Edges[i][0])].append(ord(Edges[i][1]))
 
    for i in range(1000):
        G[i] = sorted(G[i])
 
    LexiBFS(S)
 
# Driver Code
if __name__ == '__main__':
     
    N, M = 10, 10
    S = 'a'
     
    Edges = [ [ 'a', 'y' ], [ 'a', 'z' ],
              [ 'a', 'p' ], [ 'p', 'c' ],
              [ 'p', 'b' ], [ 'y', 'm' ],
              [ 'y', 'l' ], [ 'z', 'h' ],
              [ 'z', 'g' ], [ 'z', 'i' ] ]
 
    # Function Call
    CreateGraph(N, M, S, Edges)
 
# This code is contributed by mohit kumar 29

Javascript

   
// Javascript program to implement
// the above approach
 
// Function to traverse the graph in
// lexicographical order using BFS
function LexiBFS(G, S, vis)
{
     
    // Stores nodes of the graph
    // at each level
    var q = [];
 
    // Insert nodes of first level
    q.push(S);
 
    // Mark S as
    // visited node
    vis.set(S, true);
 
    // Traverse all nodes of the graph
    while (q.length != 0)
    {
         
        // Stores top node of queue
        var top = q[0];
 
        // Print visited nodes of graph
        document.write( top + " ");
 
        if (G.has(top))
        {
             
            // Insert all adjacent nodes
            // of the graph into queue
            [...G.get(top)].sort().forEach(value => {
                 
                // If i is not visited
                if (!vis.has(value))
                {
                     
                    // Mark i as visited node
                    vis.set(value, true);
 
                    // Insert i into queue
                    q.push(value);
                }
            });
        }
     
        // Pop top element of the queue
        q.shift();
    }
}
 
// Utility Function to traverse graph
// in lexicographical order of nodes
function CreateGraph(N, M, S, Edges)
{
     
    // Store all the adjacent nodes
    // of each node of a graph
    var G = new Map();
 
    // Traverse Edges[][2] array
    for(var i = 0; i < M; i++)
    {
        if (G.has(Edges[i][0]))
        {
            var tmp = G.get(Edges[i][0]);
            tmp.add(Edges[i][1]);
            G.set(Edges[i][0], tmp);
        }
        else
        {
            var tmp = new Set();
            tmp.add(Edges[i][1])
            G.set(Edges[i][0], tmp)
        }
    }
 
    // Check if a node is already visited or not
    var vis = new Map();
 
    LexiBFS(G, S, vis);
}
 
// Driver Code
var N = 10, M = 10, S = 'a';
var Edges = [ [ 'a', 'y' ], [ 'a', 'z' ],
              [ 'a', 'p' ], [ 'p', 'c' ],
              [ 'p', 'b' ], [ 'y', 'm' ],
              [ 'y', 'l' ], [ 'z', 'h' ],
              [ 'z', 'g' ], [ 'z', 'i' ] ];
               
// Function Call
CreateGraph(N, M, S, Edges);
 
// This code is contributed by rutvik_56
 

输出：

a p y z b c l m g h i

时间复杂度： O(N * log(N))
辅助空间： O(N)

如果您希望与专家一起参加现场课程，请参阅DSA 现场工作专业课程和学生竞争性编程现场课程。