杨氏模量

杨氏模量以英国物理学家托马斯·杨命名。应力（每单位面积的力）和应变（物体的比例变形）之间的关系由杨氏模量定义。当给刚性材料施加一定的载荷时，它会变形。当重量从弹性材料中撤出时，身体恢复到原来的形式。除了最小程度的变形，材料通常不是线性和弹性的。只有线弹性表面具有稳定的杨氏模量。

与体积模量、泊松比和剪切模量一样，杨氏模量也是一个弹性常数。因此，在了解杨氏模量的概念之前，让我们先了解一下弹性常数。

弹性常数

弹性常数是确定由作用于材料的给定应力系统产生的变形的常数。有许多类型的弹性常数，例如体积模量、杨氏模量或弹性模量、泊松比和剪切模量或刚性模量。当变形力作用在物体上时，它会导致实体的原始尺寸发生变化。在这种情况下，弹性常数之间的关系可以用来确定变形程度。

以下是需要学习的主要弹性常数：

1.  Bulk Modulus: It is given by the ratio of pressure (P) applied to the corresponding relative decrease in the volume or volumetric strain (∈_V) of the material. Mathematically, it is defined as:

K = P / ∈_V = P / -(∆V/V₀)

where ∆V is the change of the volume of the material due to the compression and V₀ is the Initial volume of the material.

The value is denoted with a symbol of K, and it has the dimension of force per unit area. 

It is expressed in the units per square inch (psi) in the English system and Newtons per square meter (N/m²) in the metric system.

2.  Young’s Modulus: This is a measure of a solid’s stiffness or resistance to elastic deformation under load. It relates stress (force per unit area) to strain (proportional deformation) along an axis or line. Mathematically, it is defined as:

Y = (F/A) / -(∆L/L₀)

where ∆L is the change of the length of the material due to the compression and L₀ is the initial length of the material.

The value is denoted with a symbol of Y, and it has the dimension of force per unit area. 

The SI unit for Young’s modulus is Pascal (Pa), values are most often expressed in terms of megapascal (MPa) or Newtons per square millimeter (N/mm²).

3.  Shear Modulus or Modulus of Rigidity: The ratio of shearing stress (or) tangential stress and shearing strain (or) tangential strain is called modulus of rigidity. Mathematically, it is defined as:

η = (F/A) / ∅ 

where F is the force applied on a unit surface area of the body A and ∅ is the shearing strain.

The value is denoted with a symbol of η. 

It is expressed Pascals (Pa) in the SI system.

现在，让我们进一步详细讨论杨氏弹性模量这个话题：

杨氏弹性模量

杨氏模量是一个数学常数。它以 18 世纪英国医生和科学家Thomas Young的名字命名。它定义了仅在一个方向上受到拉伸或压缩的固体的弹性特性。例如，考虑一根金属棒，它在纵向拉伸或挤压后恢复到原来的长度。

它是一种材料在受到纵向拉伸或压缩时承受长度变化的能力的量度。它也被称为弹性模量。我们通过除以计算它。它被计算为纵向应力除以应变。在张紧金属棒的情况下，可以同时说明应力和应变。

杨氏模量，也称为弹性模量或拉伸模量，是对棒材、线材等线弹性固体的力学性能测量。存在其他数字，可以为我们提供材料弹性特性的度量。体积模量和剪切模量是两个例子。然而，杨氏模量的值是最常用的。这是因为它提供了有关材料拉伸弹性的信息。

当材料被压缩或拉伸时，它会发生弹性变形，并在释放载荷时恢复其原始形状。当柔性材料变形时，它比刚性物质变形时变形更大。换句话说，它可以解释为：

• 具有低杨氏模量值的固体是弹性的。
• 具有高杨氏模量值的固体是无弹性的或僵硬的。

Young’s modulus is described as a material’s mechanical ability to tolerate compression or elongation with respect to its initial length.

杨氏模量公式：

在数学上，杨氏模量定义为施加于材料的应力与对应于材料中施加的应力的应变之比，如下所示：

杨氏模量 = 应力/应变

要么

Y = σ / ε

其中 Y 是材料的杨氏模量，σ 是施加到材料上的应力，ε 是对应于材料中施加的应力的应变。

杨氏模量单位：

杨氏模量的 SI 单位是帕斯卡 (Pa) 。

Y 的维度公式是[ML ^-1 T ^-2 ] 。

这些值通常以兆帕 (MPa)、牛顿每平方毫米 (N/mm ² )、千兆帕 (GPa) 或千牛顿每平方毫米 (kN/mm ² ) 表示。

一些材料的杨氏模量如下：

	Materials	Young’s Modulus (Y) in Nm-2
1.	Rubber	5 × 108
2.	Bone	1.4 × 1010
3.	Lead	1.6 × 1010
4.	Aluminum	7.0 × 1010
5.	Brass	9.0 × 1010
6.	Copper	11.0 × 1010
7.	Iron	19.0 × 1010

杨氏模量的数学解释：

考虑一条半径为 r 和长度为 L 的线。让力 F 沿线的长度施加在线上，即垂直于线的表面，如图所示。如果 △L 是导线长度的变化，则为拉伸应力 (σ = F/A)，其中 A 是导线的横截面积和纵向应变 (ε = △L/L)。

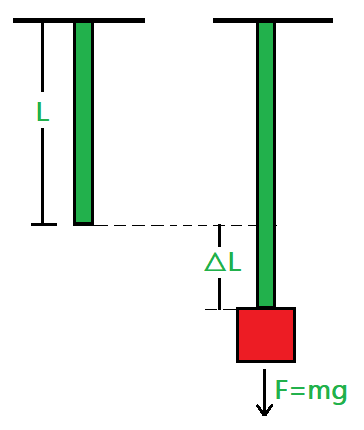
因此，这种情况下的杨氏模量由下式给出：

Y = (F/A) / (△L/L)

= (F × L) / (A × △L)

如果伸展是由质量为 m 的载荷产生的，则力 F 为mg ，其中 m 为质量，g 为重力加速度。

并且导线的横截面面积 A 是πr ²其中 r 是导线的半径。

因此，上式可写为：

Y = (m × g × L) / (πr ² × △L)

Therefore, Factors on which the Young’s Modulus of a material depends are:

• Larger the value of Young’s modulus of the material, the larger the value of the force required to change of length of the material. 
• Young’s modulus of an object depends upon the nature of the material of the object. 
• Young’s modulus of an object does not depend upon the dimensions (i.e., length, breadth, area, etc) of the object. 
• Young’s modulus of a substance decreases with an increase in temperature.
• Young’s modulus of elasticity of a perfectly rigid body is infinite.

因此，根据上述表达式，物体长度 (△L) 和质量 (m) 变化的斜率如下所示：

△L 和 m 之间的图表。

示例问题

问题 1：电缆被剪成一半长度。为什么此更改对最大负载电缆驾驶室支撑没有影响？

解决方案：

The maximum load a cable can support is given by:

F = (YA△L) / L

Here Y and A is constant, there is no change in the value of △L/L.

Hence, no effect on the maximum load.

问题 2：完美刚体的杨氏模量是多少？

解决方案：

The Young’s modulus for a material is,

Y=(F/A) / (△L/L) 

 Here, △L = 0 for rigid body. Hence, Young’s Modulus is infinite.

问题 3：钢的杨氏模量比橡胶大得多。如果纵向应变相同，哪一个将具有更大的拉伸应力？

解决方案：

Since the Tensile stress of material is equal to the product of Young’s modulus (Y) and the longitudinal strain. As steel have larger Young’s modulus therefore have more tensile strain.

问题4：500 N 的力导致横截面积为10 ^-6 m ²的导线长度增加0.5%。计算导线的杨氏模量。

解决方案：

Given that, 

The force acting, F = 1000 N, 

The cross-sectional area of the wire, A = 10^-6 m²

Therefore,    

△L/L = 0.5 = 5/1000 = 0.005

 Y = (F/A)/(△L/L)

    = 10¹² Nm^-2

问题 5：完美刚体的体积模量是多少？

解决方案：

Since, the Bulk modulus of a material is defined as,

K= P / (△V/V)

Since, △V = 0 for perfect rigid body.

Hence, the bulk modulus is infinite for perfect rigid body.