弹簧常数公式

简谐运动，或 SHM，是一种迷人的运动。它通常用于物体的振荡运动。 SHM 常见于弹簧中。弹簧具有定义其刚度的固有“弹簧常数”。胡克定律是一个众所周知的定律，它解释了 SHM，并提供了一个使用弹簧常数的施加力的公式。

胡克定律

根据胡克定律，压缩或拉伸弹簧所需的力与拉伸长度成正比。当弹簧被拉动时，牛顿第三运动定律表明它会以恢复力返回。该恢复力遵循胡克定律，该定律将弹簧力与恒定弹簧力联系起来。

Spring Force = -(Spring Constant) × (Displacement)

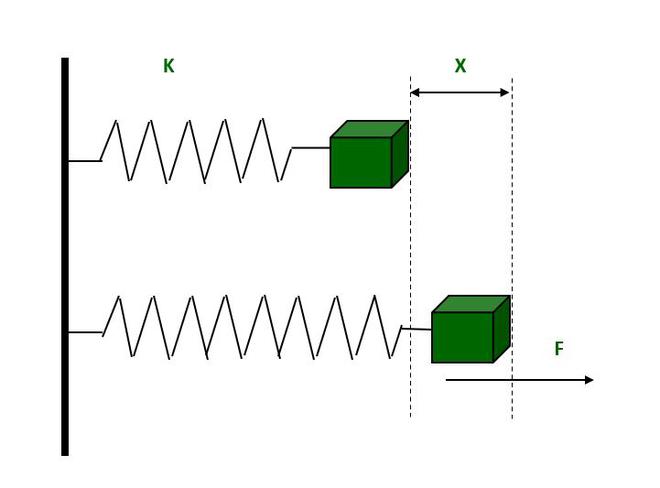
F = -KX

The negative sign indicates that the reaction force is pointing in the opposite direction.

Where,

F: The restoring force of the spring, directed towards equilibrium.

K: The spring constant in N.m^-1.

X: The spring’s displacement from its equilibrium position.

弹簧常数 (K)

弹簧常数现在定义为每单位弹簧伸长所需的力。了解弹簧常数可以轻松计算使弹簧变形所需的力。

From Hooke’s law,

F = -KX 

K = -F/ X ⇢ (1)

Equation (1) is a formula for spring constant and It is measured in N/m (Newton per meter).

弹簧常数尺寸公式

众所周知，

F = -KX

Therefore, K = -F/ X

Dimension of F = [MLT^-2]

Dimension of X = [L]

Therefore, dimension of K = [MLT⁻²]/[L] = [MT⁻²].

弹簧势能 (PE)

储存在可压缩或可拉伸物体中的能量称为弹簧势能。它也被称为弹性势能。它等于力乘以行进的距离。

It is known that, Potential energy = force × displacement

And also force of the spring is equal to the spring constant × displacement. So,  

P.E. = 1/2 KX² .⇢ (2)

The above equation is formula of spring potential energy.

胡克定律的局限性

胡克定律有一个限制，它只适用于任何材料的弹性极限，这意味着材料必须具有完全的弹性才能遵守胡克定律。胡克定律本质上打破了弹性极限。

胡克定律的应用

• 由于弹簧的弹性，胡克定律最常用于弹簧。
• 它们不仅用于工程领域，还用于医学领域。
• 它用于肺、皮肤、弹簧床、跳水板和汽车悬架系统。
• 这是压力计、弹簧刻度和时钟摆轮的基本原理。
• 它也是地震学、声学和分子力学的基础。

应用胡克定律的缺点

以下是胡克定律的缺点：

• 胡克定律仅适用于失效后的弹性区域。
• 胡克定律仅对具有小力和变形的实体产生准确的结果。
• 胡克定律不是一般规则。

示例问题

问题一：Spring 常量的定义是什么？

回答：

When a spring is stretched, the force exerted is proportional to the increase in length from the equilibrium length, according to Hooke’s Law. The spring constant can be calculated using the following formula: k = -F/x, where k is the spring constant. F denotes the force, and x denotes the change in spring length.

问题 2：长度如何影响弹簧常数？

回答：

Assume there is a 6 cm spring with a spring constant k. What happens if the spring is divided into two equal-sized pieces? One of these shorter springs will have a new spring constant of 2k. In general, assuming a specific material spring and thickness, the spring constant of a spring is inversely proportional to the length of the spring.

So, in the preceding example, suppose the spring exactly cut in half, resulting in two shorter springs, each 3 cm in length. For the smaller springs, a spring constant twice as large as the original will be used. This happens because it is inversely proportional to both the spring constant and the spring length. 

问题 3：弹簧被 2N 的力拉伸 4m。确定其弹簧常数。

解决方案 ：

Given,

Force, F = 2 N and

Displacement, X = 4 m.

We know that, 

The Spring constant, K = – F/X

K = – 2N / 4m 

K = – 0.5 Nm^-1 . 

问题 4： 10 N 的力施加到字符串上并被拉伸。如果弹簧常数是 4 Nm ^-1然后计算字符串的位移。

解决方案：

Given, 

Force, F = 10 N and

Spring constant, K = 4 Nm^-1

We know that, F = – KX 

X (Displacement) = – F/K

X = – ( 10 N / 4 Nm^-1 )

X = – 2.5 m.

问题 5：如果弹簧常数为 0.1 Nm ^-1 ，将一个 3 米长的弹簧拉伸到 5 米需要多大的力。

解决方案 ：

Given, 

Length of spring  = 3m

Spring constant, K = 0.1 Nm^-1

Stretch it to 5 meter so the displacement of the spring is  X = 5 – 3 = 2m

Now, Required Force is F = -KX

F = – (0.1 Nm^-1 × 2m )

F = – 0.2 N.