商规则

在区分方面，由一些算子连接的两个功能也可以根据使用的运算符类型很容易区分。假设，在两个函数之间使用了一个正号，这些函数可以用 +ve 符号单独区分，同样的情况也用一个负号发生。但是，乘法或除法符号是不可能的。在乘法中，每个函数分别微分，另一个函数为常数，如果函数是除法，则规则变得有点复杂。让我们了解这个著名的微分商规则。

什么是商数法则？

假设给定两个函数f(x)和g(x)，并且两个函数都是可微的，即两个函数的导数都存在，那么用商法则求解给定函数的商。

商规则指出整个函数的导数是分子乘以分母的导数减去分母乘以分子整数的导数除以分母的平方

$H'(x)=\frac{d}{dx}\frac{f(x)}{g(x)}=\frac{[f'(x)g(x)-f(x)g'(x)]}{[g(x)]^2}$

商规则很容易记住，因为分子与乘积规则相同，只要确保放负号而不是正号，分母也只是分母函数的平方。

商规则证明：

商规则有多种证明方法，其中一种证明规则的方法是使用标准导数定义和极限性质。另一种方法是简单地应用产品规则并安排功能。

让我们用后一种方法证明商规则，

To Prove, $H'(x)=\frac{d}{dx}\frac{f(x)}{g(x)}=\frac{[f'(x)g(x)-f(x)g'(x)]}{[g(x)]^2}$

Given, H(x)=f(x)/g(x)

Proof,

f(x)= H(x)g(x)

Applying Product rule here,

f'(x)=H'(x)g(x)+g'(x)H(x)

Put the value of H(x),

$H'(x)= \frac{f'(x)-\frac{g'(x)f(x)}{g(x)}}{g(x)}$

$H'(x)= \frac{f'(x)g(x)-{g'(x)f(x)}}{g(x)^2}$

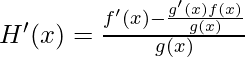
Hence, Proved.

示例问题

问题 1：区分y=  $\frac{x^3-x+2}{x^2+5}$ 

解决方案：

Both numerator and denominator functions are differentiable.

Applying quotient rule,

$y'=\frac {d}{dx}[\frac{x^3-5+2}{x^2+5}]$

$y'= \frac{[d/dx(x^3-x+2)(x^2+5)-(x^3-x+2)d/dx(x^2+5)]}{[x^2+5]^2}$

$y'= \frac{[(3x^2-1)(x^2+5)-(x^3-x+2)(2x)]}{[x^2+5]^2}\\=\frac{(3x^4+15x^2-x^2-5)-(2x^4-2x^2+4x)}{[x^2+5]^2}$

$y'= \frac{x^4+16x^2-4x-5}{[x^2+5]^2}$

问题2：微分，f(x) = tanx

解决方案：

tanx can be written as sinx/cosx

Both the numerator and denominator functions have a possible derivative.

Applying quotient rule,

$f'(x)=\frac{(d/dx(sinx))(cosx)-(d/dx(cosx))(sinx)}{cos^2x}$

$f'(x)= \frac{cosx.cosx-(-sinx)(sinx)}{cos^x}$

$f'(x)=\frac{cos^2x+sin^2x}{cos^2x}$

$f'(x)=\frac{1}{cos^2x}$

问题3：区分， $f(y)=\frac{8}{y^3}$

解决方案：

Derivatives of both numerator and denominator are present,

Applying quotient rule,

$f'(y)=\frac{(d/dy(8))(x^3)-(d/dy(x^3))(8)}{x^6}$

$f'(y)=\frac{0.x^3-3x^2(8)}{x^6}$

$f'(y)=\frac{-24x^2}{x^6}$

问题4：区分， $y=\frac{\sqrt{x}+x}{x+2}$

解决方案：

Both numerator and denominator have possible derivatives.

Applying quotient rule,

$y'=d/dx[\frac{\sqrt{x}+x}{x+2}]$

$y'=\frac{d/dx[\sqrt{x}+x](x+2)-d/dx(x+2)(\sqrt{x}+x)}{[x+2]^2}$

$y'=\frac{(\frac{1}{2\sqrt{x}}+1)(x+2)-(\sqrt{x}+x)}{[x+2]^2}$

$y'=\frac{\frac{\sqrt{x}}{2}+\frac{1}{\sqrt{x}}+2-\sqrt{x}}{[x+2]^2}$

$y'=\frac{\frac{-\sqrt{x}}{2}+\frac{1}{\sqrt{x}}+2}{[x+2]^2}$

问题5：微分，f(x)= e ^x /x ²

解决方案：

Both numerator and denominator have possible derivatives,

Applying quotient rule,

$f'(x)=[\frac{d/dx(e^x)(x^2)-d/dx(x^2)(e^x)}{x^4}]$

$f'(x)=\frac{e^x.x^2-2xe^x}{x^4}$

问题6：区分， $y=\frac{cosx}{x^2}$

解决方案：

Both numerator and denominator have possible derivatives,

Applying quotient rule,

$y'=\frac{d/dx(cosx)(x^2)-d/dx(x^2)(cosx)}{x^4}$

$y'=\frac{-sinx(x^2)-(2x)(cosx)}{x^4}$

$y'=\frac{-(x^2)sinx-(2xcosx)}{x^4}$

问题7：微分，f(p)= p+5/p+7

解决方案：

Both numerator and denominator have possible derivatives.

Applying Quotient rule,

$f'(p)=d/dx[\frac{p+5}{p+7}]$

$f'(p)=[\frac{d/dx(p+5)(p+7)-d/dx(p+7)(p+5)}{(p+7)^2}]$

$f'(p)=[\frac{p+7-p-5}{(p+7)^2}]$

$f'(p)=[\frac{2}{(p+7)^2}]$

问题8：微分，f(x)= $\frac{x^4+3}{\sqrt{x+5}}$

解决方案：

Both numerator and denominator have possible derivatives

Applying quotient rule,

$f'(x)= d/dx[\frac{x^4+3}{\sqrt{x+5}}]$

$f'(x)=\frac{d/dx(x^4+3)(\sqrt{x+5})-d/dx(\sqrt{x+5})(x^4+3)}{{(x+5)}}$

$f'(x)=\frac{(4x^3)(\sqrt{x+5})-(\frac{1}{2\sqrt{x+5}})(x^4+3)}{{x+5}}$

$f'(x)=\frac{(4x^3)({x+5})-(\frac{1}{2})(x^4+3)}{{(x+5)^{3/2}}}$

$f'(x)=\frac{(8x^3)({x+5})-(x^4+3)}{{2(x+5)^{3/2}}}$

$f'(x)=\frac{8x^4+40x^3-x^4-3}{{2(x+5)^{3/2}}}$

$f'(x)=\frac{7x^4+40x^3-3}{{2(x+5)^{3/2}}}$