第 12 类 RD Sharma 解 – 第 20 章定积分 – 练习 20.2 |设置 1
计算以下定积分:
问题 1。 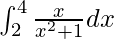
解决方案:
We have,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题2。 
解决方案:
We have,
I = ![]()
Let 1 + log x = t, so we have,
=> (1/x) dx = 2t dt
Now, the lower limit is, x = 1
=> t = 1 + log x
=> t = 1 + log 1
=> t = 1 + 0
=> t = 1
Also, the upper limit is, x = 2
=> t = 1 + log x
=> t = 1 + log 2
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 3。 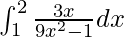
解决方案:
We have,
I = ![]()
Let 9x2 – 1 = t, so we have,
=> 18x dx = dt
=> 3x dx = dt/6
Now, the lower limit is, x = 1
=> t = 9x2 – 1
=> t = 9 (1)2 – 1
=> t = 9 – 1
=> t = 8
Also, the upper limit is, x = 2
=> t = 9x2 – 1
=> t = 9 (2)2 – 1
=> t = 36 – 1
=> t = 35
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 4。 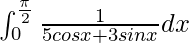
解决方案:
We have,
I = ![]()
On putting sin x = ![]() and cos x =
and cos x = 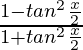 , we get
, we get
I = 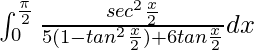
I = 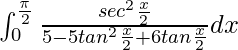
Let tan x/2 = t. So, we have
=> ![]() = dt
= dt
Now, the lower limit is, x = 0
=> t = tan x/2
=> t = tan 0
=> t = 0
Also, the upper limit is, x = π/2
=> t = tan x/2
=> t = tan π/4
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![Rendered by QuickLaTeX.com \left[\frac{2}{5}.\frac{1}{2}\sqrt{\frac{25}{34}}\log\left(\frac{\sqrt{\frac{34}{25}}+t-\frac{3}{5}}{\sqrt{\frac{34}{25}}-t+\frac{3}{5}}\right)\right]^1_0](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_40.jpg)
I = ![Rendered by QuickLaTeX.com \left[\frac{2}{5}.\frac{1}{2}\frac{5}{\sqrt{34}}\log\left(\frac{\sqrt{\frac{34}{25}}+t-\frac{3}{5}}{\sqrt{\frac{34}{25}}-t+\frac{3}{5}}\right)\right]^1_0](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_41.jpg)
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 5。 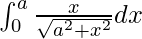
解决方案:
We have,
I = ![]()
Let a2 + x2 = t2. So, we have
=> 2x dx = 2t dt
=> x dx = t dt
Now, the lower limit is, x = 0
=> t2 = a2 + x2
=> t2 = a2 + 02
=> t2 = a2
=> t = a
Also, the upper limit is, x = a
=> t2 = a2 + x2
=> t2 = a2 + a2
=> t2 = 2a2
=> t = √2 a
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = √2a – a
I = a (√2 – 1)
Therefore, the value of ![]() is a (√2 – 1).
is a (√2 – 1).
问题 6。 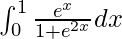
解决方案:
We have,
I = ![]()
Let ex = t. So, we have
=> ex dx = dt
Now, the lower limit is, x = 0
=> t = ex
=> t = e0
=> t = 1
Also, the upper limit is, x = a
=> t = ex
=> t = e1
=> t = e
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 7。 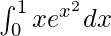
解决方案:
We have,
I = ![]()
Let x2 = t. So, we have
=> 2x dx = dt
Now, the lower limit is, x = 0
=> t = x2
=> t = 02
=> t = 0
Also, the upper limit is, x = 1
=> t = x2
=> t = 12
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 8。 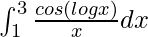
解决方案:
We have,
I = ![]()
Let log x = t. So, we have
=> (1/x) dx = dt
Now, the lower limit is, x = 1
=> t = log x
=> t = log 1
=> t = 0
Also, the upper limit is, x = 3
=> t = log x
=> t = log 3
So, the equation becomes,
I = ![]()
I = ![]()
I = sin (log 3) – sin 0
I = sin (log 3) – 0
I = sin (log 3)
Therefore, the value of ![]() is sin (log 3).
is sin (log 3).
问题 9。 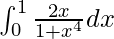
解决方案:
We have,
I = ![]()
Let x2 = t. So, we have
=> 2x dx = dt
Now, the lower limit is, x = 0
=> t = x2
=> t = 02
=> t = 0
Also, the upper limit is, x = 1
=> t = x2
=> t = 12
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 10。 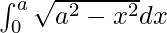
解决方案:
We have,
I = ![]()
Let x = a sin t. So, we have
=> dx = a cos t dt
Now, the lower limit is, x = 0
=> a sin t = x
=> a sin t = 0
=> sin t = 0
=> t = 0
Also, the upper limit is, x = a
=> a sin t = a
=> a sin t = a
=> sin t = 1
=> t = π/2
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 11。 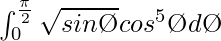
解决方案:
We have,
I = ![]()
I = ![]()
Let sin Ø = t. So, we have
=> cos Ø dØ = dt
Now, the lower limit is, Ø = 0
=> t = sin Ø
=> t = sin 0
=> t = 0
Also, the upper limit is, Ø = π/2
=> t = sin Ø
=> t = sin π/2
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![Rendered by QuickLaTeX.com \left[\frac{t^{\frac{1}{2}+1}}{\frac{1}{2}+1}+\frac{t^{\frac{9}{2}+1}}{\frac{9}{2}+1}-\frac{2t^{\frac{5}{2}+1}}{\frac{5}{2}+1}\right]_{0}^{1}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_106.jpg)
I = ![Rendered by QuickLaTeX.com \left[\frac{t^{\frac{3}{2}}}{\frac{3}{2}}+\frac{t^{\frac{11}{2}}}{\frac{11}{2}}-\frac{2t^{\frac{7}{2}}}{\frac{7}{2}}\right]_{0}^{1}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_107.jpg)
I = ![Rendered by QuickLaTeX.com \left[\frac{2t^{\frac{3}{2}}}{3}+\frac{2t^{\frac{11}{2}}}{11}-\frac{4t^{\frac{7}{2}}}{7}\right]_{0}^{1}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_108.jpg)
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 12。 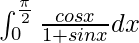
解决方案:
We have,
I = ![]()
Let sin x = t. So, we have
=> cos x dx = dt
Now, the lower limit is, x = 0
=> t = sin x
=> t = sin 0
=> t = 0
Also, the upper limit is, x = π/2
=> t = sin x
=> t = sin π/2
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 13。 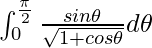
解决方案:
We have,
I = ![]()
Let 1 + cos θ = t2. So, we have
=> – sin θ dθ = 2t dt
=> sin θ dθ = –2t dt
Now, the lower limit is, θ = 0
=> t2 = 1 + cos θ
=> t2 = 1 + cos 0
=> t2 = 1 + 1
=> t2 = 2
=> t = √2
Also, the upper limit is, θ = π/2
=> t2 = 1 + cos θ
=> t2 = 1 + cos π/2
=> t2 = 1 + 0
=> t2 = 1
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 14。 
解决方案:
We have,
I = ![]()
Let 3 + 4 sin x = t. So, we have
=> 0 + 4 cos x dx = dt
=> 4 cos x dx = dt
=> cos x dx = dt/4
Now, the lower limit is, x = 0
=> t = 3 + 4 sin x
=> t = 3 + 4 sin 0
=> t = 3 + 0
=> t = 3
Also, the upper limit is, x = π/3
=> t = 3 + 4 sin x
=> t = 3 + 4 sin π/3
=> t = 3 + 4 (√3/2)
=> t = 3 + 2√3
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 15。 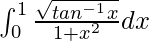
解决方案:
We have,
I = ![]()
Let tan–1 x = t. So, we have
=> (1/1+x2) dx = dt
Now, the lower limit is, x = 0
=> t = tan–1 x
=> t = tan–1 0
=> t = 0
Also, the upper limit is, x = 1
=> t = tan–1 t
=> t = tan–1 1
=> t = π/4
So, the equation becomes,
I = ![]()
I = ![Rendered by QuickLaTeX.com \left[\frac{t^{\frac{1}{2}+1}}{\frac{1}{2}+1}\right]_{0}^{\frac{\pi}{4}}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_143.jpg)
I = ![Rendered by QuickLaTeX.com \left[\frac{t^{\frac{3}{2}}}{\frac{3}{2}}\right]_{0}^{\frac{\pi}{4}}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_144.jpg)
I = ![Rendered by QuickLaTeX.com \frac{2}{3}\left[t^{\frac{3}{2}}\right]_{0}^{\frac{\pi}{4}}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_145.jpg)
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 16。 
解决方案:
We have,
I = ![]()
Let x + 2 = t2. So, we have
=> dx = 2t dt
Now, the lower limit is, x = 0
=> t2 = x + 2
=> t2 = 0 + 2
=> t2 = 2
=> t = √2
Also, the upper limit is, x = 2
=> t2 = x + 2
=> t2 = 2 + 2
=> t2 = 4
=> t = 2
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![Rendered by QuickLaTeX.com 2\left[\frac{t^5}{5}-\frac{2t^3}{3}\right]_{\sqrt{2}}^{2}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_157.jpg)
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 17。 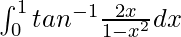
解决方案:
We have,
I = ![]()
Let x = tan t. So, we have
=> dx = sec2 t dt
Now, the lower limit is, x = 0
=> tan t = x
=> tan x = 0
=> x = 0
Also, the upper limit is, x = 1
=> tan t = x
=> tan x = 1
=> x = π/4
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
On applying integration by parts method, we get
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 18。 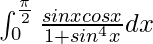
解决方案:
We have,
I = ![]()
Let sin2 x = t. So, we have
=> 2 sin x cos x = dt
=> sin x cos x = dt/2
Now, the lower limit is, x = 0
=> t = sin2 x
=> t = sin2 0
=> t = 0
Also, the upper limit is, x = π/2
=> t = sin2 x
=> t = sin2 π/2
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 19。 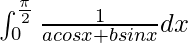
解决方案:
We have,
I = ![]()
On putting cos x = 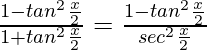 and sin x =
and sin x = ![]() , we get,
, we get,
I = 
Let tan x/2 = t. So, we have
=> 1/2 sec2 x/2 dx = dt
=> sec2 x/2 dx = 2 dt
Now, the lower limit is, x = 0
=> t = tan x/2
=> t = tan 0/2
=> t = tan 0
=> t = 0
Also, the upper limit is, x = π/2
=> t = tan x/2
=> t = tan π/4
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![Rendered by QuickLaTeX.com \frac{2}{a}\int_{0}^{1}\frac{1}{-\left[(t-\frac{b}{a})^2-1-\frac{b^2}{a^2}\right]}dt](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_196.jpg)
I = 
I = ![Rendered by QuickLaTeX.com \left[\frac{2}{a}\left(\frac{1}{2\sqrt{\frac{b^2+a^2}{a^2}}}\right)\log\left(\frac{\sqrt{\frac{b^2+a^2}{a^2}}+(t-\frac{b}{a})}{\sqrt{\frac{b^2+a^2}{a^2}}-(t-\frac{b}{a})}\right)\right]_{0}^{1}](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_198.jpg)
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.
问题 20。 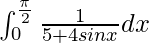
解决方案:
We have,
I = ![]()
On putting sin x = ![]() , we get
, we get
I = 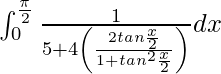
I = 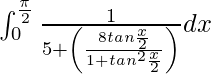
I = 
I = 
I = 
I = 
Let tan x/2 = t. So, we have
=> 1/2 sec2 x/2 dx = dt
=> sec2 x/2 dx = 2 dt
Now, the lower limit is, x = 0
=> t = tan x/2
=> t = tan 0/2
=> t = tan 0
=> t = 0
Also, the upper limit is, x = π/2
=> t = tan x/2
=> t = tan π/4
=> t = 1
So, the equation becomes,
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![]()
I = ![Rendered by QuickLaTeX.com \frac{2}{3}\left[tan^{-1}(\frac{3-\frac{4}{3}}{1+3(\frac{4}{3})})\right]](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_221.jpg)
I = ![Rendered by QuickLaTeX.com \frac{2}{3}\left[tan^{-1}(\frac{\frac{5}{3}}{1+4})\right]](https://mangodoc.oss-cn-beijing.aliyuncs.com/geek8geeks/Class_12_RD_Sharma_Solutions_%E2%80%93_Chapter_20_Definite_Integrals_%E2%80%93_Exercise_20.2_%7C_Set_1_222.jpg)
I = ![]()
I = ![]()
Therefore, the value of ![]() is
is ![]() .
.