前 n 个自然数平方和的Python程序

给定一个正整数N 。任务是找到 1 ² + 2 ² + 3 ² + ..... + N ² 。

例子：

Input : N = 4
Output : 30
12 + 22 + 32 + 42
= 1 + 4 + 9 + 16
= 30

Input : N = 5
Output : 55

方法 1：O(N)这个想法是从 1 到 n 运行一个循环，并且对于每个 i，1 <= i <= n，找到 i ²来求和。

# Python3 Program to
# find sum of square
# of first n natural 
# numbers
  
  
# Return the sum of
# square of first n
# natural numbers
def squaresum(n) :
  
    # Iterate i from 1 
    # and n finding 
    # square of i and
    # add to sum.
    sm = 0
    for i in range(1, n+1) :
        sm = sm + (i * i)
      
    return sm
  
# Driven Program
n = 4
print(squaresum(n))
  
# This code is contributed by Nikita Tiwari.*/

输出：

30

方法2：O(1)

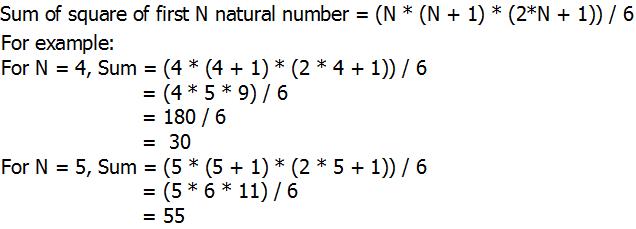
证明：

We know,
(k + 1)3 = k3 + 3 * k2 + 3 * k + 1
We can write the above identity for k from 1 to n:
23 = 13 + 3 * 12 + 3 * 1 + 1 ......... (1)
33 = 23 + 3 * 22 + 3 * 2 + 1 ......... (2)
43 = 33 + 3 * 32 + 3 * 3 + 1 ......... (3)
53 = 43 + 3 * 42 + 3 * 4 + 1 ......... (4)
...
n3 = (n - 1)3 + 3 * (n - 1)2 + 3 * (n - 1) + 1 ......... (n - 1)
(n + 1)3 = n3 + 3 * n2 + 3 * n + 1 ......... (n)

Putting equation (n - 1) in equation n,
(n + 1)3 = (n - 1)3 + 3 * (n - 1)2 + 3 * (n - 1) + 1 + 3 * n2 + 3 * n + 1
         = (n - 1)3 + 3 * (n2 + (n - 1)2) + 3 * ( n + (n - 1) ) + 1 + 1

By putting all equation, we get
(n + 1)3 = 13 + 3 * Σ k2 + 3 * Σ k + Σ 1
n3 + 3 * n2 + 3 * n + 1 = 1 + 3 * Σ k2 + 3 * (n * (n + 1))/2 + n
n3 + 3 * n2 + 3 * n = 3 * Σ k2 + 3 * (n * (n + 1))/2 + n
n3 + 3 * n2 + 2 * n - 3 * (n * (n + 1))/2 = 3 * Σ k2
n * (n2 + 3 * n + 2) - 3 * (n * (n + 1))/2 = 3 * Σ k2
n * (n + 1) * (n + 2) - 3 * (n * (n + 1))/2 = 3 * Σ k2
n * (n + 1) * (n + 2 - 3/2) = 3 * Σ k2
n * (n + 1) * (2 * n + 1)/2  = 3 * Σ k2
n * (n + 1) * (2 * n + 1)/6  = Σ k2

# Python3 Program to
# find sum of square 
# of first n natural 
# numbers
  
# Return the sum of 
# square of first n
# natural numbers
def squaresum(n) :
    return (n * (n + 1) * (2 * n + 1)) // 6
  
# Driven Program
n = 4
print(squaresum(n))
  
#This code is contributed by Nikita Tiwari.                                                               

输出：

30

避免早期溢出：
对于较大的 n， (n * (n + 1) * (2 * n + 1)) 的值会溢出。我们可以使用 n*(n+1) 必须能被 2 整除的事实在一定程度上避免溢出。

# Python Program to find sum of square of first
# n natural numbers. This program avoids
# overflow upto some extent for large value
# of n.y
  
def squaresum(n):
    return (n * (n + 1) / 2) * (2 * n + 1) / 3
  
# main()
n = 4
print(squaresum(n));
  
# Code Contributed by Mohit Gupta_OMG <(0_o)>

输出：

30

有关详细信息，请参阅有关前 n 个自然数平方和的完整文章！