材料的弹性行为

固体由基于原子弹性（或分子）的原子组成。它们被其他同类原子包围，这些原子通过原子间力保持平衡。当施加外力时，这些颗粒会发生位移，导致固体变形。当变形力被移除时，原子间的相互作用使原子恢复到它们原来的平衡状态。弹性是一种理想化，因为没有物质是完全弹性的。例如，如果您使用发带来保养自己，您可能已经看到它的尺寸会随着时间的推移而变形。它可能会在某个点之后突然发生。这是因为随着时间的推移，发带会失去弹性。

固体习惯于保持一种物理形状，因此其中包含的每个分子都处于稳定平衡的位置，即从每一对相反方向作用在物体上的平衡力。刚体是一个固体物体，其中变形可以忽略不计，出于所有实际目的假定为零。这些物体在受到外力时会在去除外力后恢复其原始形状。身体抵抗这些变化，直到达到极限外力极限，称为弹性极限。

弹性

宇宙中的任何刚性物体在外力作用下都会发生其物理方向和结构的变化。刚体在受到外力时会改变其长度、体积或形状。去除外力后，主体保持其原始形状和尺寸。因此，弹性是身体的这样一种特性，当外力移除时，任何人都倾向于恢复其原始形状（或）大小。它表现出对变革的反对。例如：橡皮筋。

固体的弹性行为

在任何固体受到外力的情况下，该物体变形并且形成它们的原子或分子从它们的原始位置移位，从而被扰乱平衡状态。

位移导致固定点的修改，从而导致原子间和分子内距离的变化。因此，变形可以被称为任何物体的结构由于作用在其上的力的影响而发生的变化。引起这些粒子位移变化的力称为变形力。

因为我们知道每一个力都有一个相等和相反的力作用。变形力与恢复力相反，作用方向相反。在移除变形力后，该力推动身体恢复其原始位置。

弹簧球系统包含表示原子的球和表示作用在它们之间的力的弹簧。

用于说明固体弹性行为的弹簧球模型

胡克定律

Hooke’s law states that within the elastic limit, stress developed is directly proportional to the strain produced in a body. This implies that if the strain on anybody increases the stress will increase and vice-versa. 

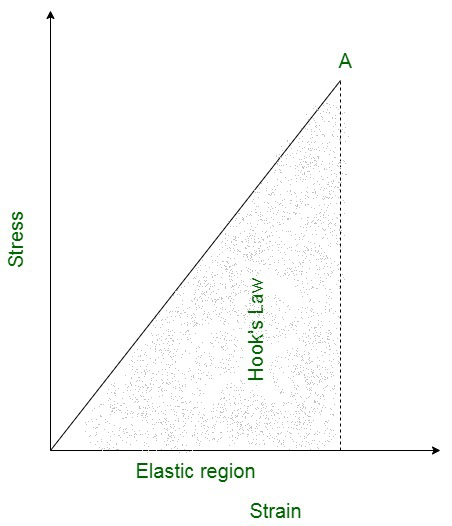
胡克定律适用于所有具有弹性的物体。在数学上，胡克定律定义为，

应力∝应变

让我们假设一个比例常数 k。

所以，

应力 = k × 应变

这里，平衡因子 k 被称为弹性模量。

弹性模量

既然我们知道弹性材料是由于外力而发生变形的，它就会保持一个内阻来抵抗变形，如果不再施加外力，它就会恢复到原来的状态。有不同类型的弹性模量：

1. 杨氏模量：杨氏模量是物体的特性，因为它能够承受长度的变化，在纵向张力或力的情况下，以压缩的形式施加。杨氏模量等于纵向应力除以物体的应变。用 Y 表示。
2. 剪切模量：也称为刚性模量，剪切模量定义为剪切应力与剪切应变的比值。它用“G”表示。
3. 体积模量：体积模量是液压应力与相应液压应变的比值。用“B”表示

固体弹性行为的应用

当你拉伸弹弓时，它会变形。当你移除力时，它会恢复到原来的形状。但是假设您拿一根细钢棒并尝试弯曲它。你稍微弯曲它，然后停止用力。棒能恢复到原来的形状吗？它没有，它没有。材料行为的这种差异是由于其弹性和塑性特性造成的。

弹弓的橡胶条非常柔韧。弹性是身体在承受压力时抵抗不可逆变化的能力。当张力消除时，身体恢复到原来的形状和大小。不同的材料表现出不同程度的弹性。研究材料的弹性行为非常重要。几乎每个工程设计都需要了解材料的弹性行为。用于建造不同的建筑，如桥梁、柱子、柱子和梁等。了解建筑中使用的材料的强度至关重要。材料的弹性行为主要在三种主要情况下被考虑和学习：

1. 起重机使用的钢丝绳的厚度。
2. 桥梁设计，
3. 山的最大高度。

起重机使用的钢丝绳的厚度。

用于提升负载的起重机使用的绳索设计为使最大负载产生的应力不超过断裂应力。还发现，当压在一起时，一组较细的线股使绳索比相同横截面的实心绳索更坚固。这就是为什么起重机绳索是由几股而不是一根制成的。

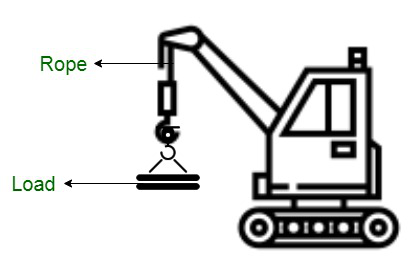
We can find that how thick rope is required for a crane; The load should not deform the rope permanently. Therefore, the extension should not exceed the elastic limit. Mild steel has a yield strength (S_y) of about 300 × 10⁶ Nm^-2.  Thus, the area of cross-section (A) of the rope should at least be:

Elastic limit, S_y = Load to be lifted / Cross-sectional Area = W / A

The load to be lifted by the crane is equal to the weight (W) that is to lift which must be equal to the product of the mass (m) of the weight lifted and gravitational acceleration (g) that is, mg.

And since, the rope is circular-shaped of radius (r) so, its area of cross-section (A) is πr².

Therefore,

S_y = W / A = mg / πr²  

Now, A should be greater than or equals to W / S_y or mg / S_y  

i.e.

A ≥ (10⁴ kg × 10 ms²) / (300 × 10⁶ Nm^-2)

   = 3.3 × 10^-4 m²

This corresponds to the radius of about 1 cm for a rope of circular cross-section. Generally, a large margin of safety (of about a factor of ten in the load) is provided. Thus, a thicker rope of radius about 3 cm is recommended.

桥梁设计

一座桥梁的设计必须使其能够承受流动的交通负荷、风力，甚至是自身的重量。

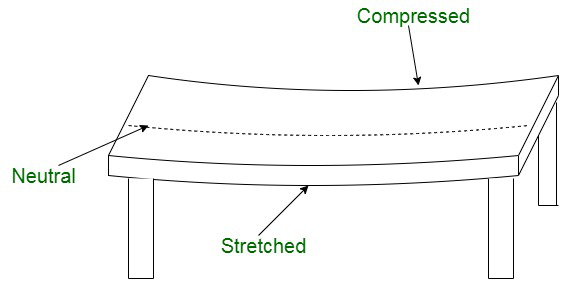
弹性行为或换句话说梁的弯曲是建筑物或桥梁稳定性的主要问题。梁不应弯曲太多或断裂，梁在中心加载并在其末端附近支撑。一根长 l、宽 b、深 d 的杆，当在中心被负载 W 加载时，将有凹陷：

δ = WL ³ / 3YI _G

其中 W 是负载，L 是长度，Y 是杨氏模量， _IG是几何惯性矩。

山的最大高度

Let us assume, the height of the mountains at the bottom is h, the force per unit area due to the weight of the mountain is hρg where ρ is the density of the material of the mountain and g is the acceleration due to gravity.

The material at the bottom experiences this force in the vertical direction, and the sides of the mountain are free. Now the elastic limit for a typical rock is 30 × 107 Nm-2 and put ρ = 3 × 103 kg m-3 therefore, this implies:

hρg = 30 × 107 Nm^-2

Rearrange the above expression for h.

Hence, the maximum height of the mountains should be,

h = 30 × 10⁷ Nm^-2 / ρ × g

  = 30 × 10⁷ Nm-2 / 3 × 10³ kg m^-3 × 10 ms^-2

  = 10000 m or 10 km

This is why the height of the mountains cannot be higher than 10 km.

示例问题

问题1：给出一个为什么用钢来建造桥梁的原因？

解决方案：

Steel has the strongest and most favorable strength attributes of any bridging material, making it appropriate for the most daring bridges with the greatest spans. Normal construction steel has compressive and tensile strengths of 370 N/sq mm, which is approximately ten times the compressive strength of medium concrete and one hundred times its tensile strength.

Steel has a unique property called ductility, which allows it to bend significantly before breaking because it begins to give over a specific stress threshold.

问题2：确定钢索中原子间距为2.5 Å，杨氏模量值为20 × 10 ¹⁰ N/m ²时的力常数。

解决方案：

The formula to calculate the force constant is,

K = Y × r₀​

where Y is the young’s modulus and r₀ is the interatomic spacing.

Lets substitute the given values in the above expression as,

K = 20 × 10¹⁰ N/m² × 2.5 Å × (10^-10 m / 1 Å)

   = 6 × 10⁻⁹ N/Å

问题3：螺旋弹簧的拉伸是由哪个模数类型决定的，为什么？

解决方案：

Upon stretching a coil spring, a change in its shape is noticed. The length as well as the volume remain unmodified, therefore, it can be determined by evaluation through shear modulus.

问题4：哪种材料的弹性更好，钢还是橡胶？

解决方案：

Steel is more elastic than rubber because it returns to its original position faster than rubber.

问题 5：在构建用于支撑负载的梁时，梁中间的倾角如何与其杨氏模量相关。

解决方案：

The relation for the determination of the dip in the middle of the beam is,

δ = WL³ / 3YI_G

where W is the load, L is the length, Y is the Young’s Modulus and I_G is the geometrical moment of inertia.

However, here if the load, length and geometrical moment of inertia of the beam remains constant then,

δ ∝ 1 / Y

Hence, the dip in the middle of the beam is inversely proportional to its Young’s modulus.