📌  相关文章
📜  粘度

📅  最后修改于: 2022-05-13 01:57:38.082000             🧑  作者: Mango

粘度

假设一个人有两个碗，一个碗盛水，另一个碗盛蜂蜜，蜂蜜比水需要更多的时间来形成碗的形状。发生这种情况是因为两种流体的粘度不同。粘度是防止液体扩散的液体特性。由于液体的性质，每种流体都反对其不同层之间的相对运动，因此称为粘度。由于粘性而产生的力称为粘性力。由于这种力是在液体层之间，所以也称为内摩擦力。

在本文中，将学习液体中发现的重要特性的各种不同问题。流动的流体具有许多特性，流动的流体在其内部携带许多能量。流动的流体有很多因素阻碍它成为理想的液体，并不是所有的液体都是理想的液体，它们会对运动产生一定的阻力。液体运动中的这种阻力可以看作是内部摩擦，类似于固体表面运动产生的摩擦，称为粘度。当流体表面具有相对运动时，就会出现这种力。

粘度

每当液体的这些层之间存在相对运动时，液体的不同层之间就会产生相反的力（内摩擦），这种液体的特性称为粘度。

想象一个流体在水平面上与其平行移动。流体可以被认为由几层组成。如果流体流动剧烈，则与水平面接触的层的速度约为零。但随着层距水平面的距离增加，层流的速度会增加。

如上图所示，最上面的流体层有一个最大的速度，因此，流体的两个相邻地址的速度总是不同的。一层比另一层流动得更快，就像在固体中一样，由于它们的相对运动，相反的摩擦力作用在它们的整个平面之间。同样，由于流体中相邻层之间的相对运动，它们之间也会产生相对于层的摩擦力来抵抗运动。换言之，可以说低速流动的层试图降低快速流动的层的速度。流体的这种性质称为粘度。

粘度系数

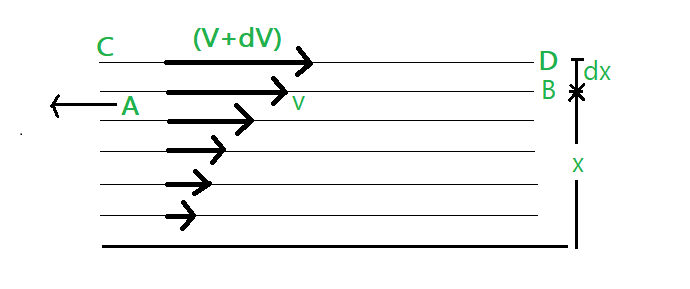
粘度系数的表示

考虑液体在水平固体表面上的流动，如图所示。让我们考虑两层 AB 和 CD 分别以速度 v 和 (v + dv) 从固定的固体表面移动。根据牛顿粘度定律，这些层之间的固体表面上的粘性阻力或反向力 (F) 为，

  • 与层的面积 (A) 成正比。
  • 与层间的速度梯度 (dv/dx) 成正比。

因此，它可以写成：

F ∝ A (dv/dx)

让我们通过引入比例常数 η 来去除比例符号。

F = -η A (dv/dx)

这里 η 称为粘度系数。此外，上式中的负号表示粘性阻力 (F) 的方向与液体的运动方向正好相反。

如果 A = 1 m 2并且 dv/dx = 1 s -1那么上面的表达式变为：

η = F

因此，液体的粘度系数被定义为在具有垂直于液体流动方向的单位速度梯度的层的单位面积上作用的粘性阻力或力。

粘度系数单位

  • 在 CGS 系统中，粘度系数的单位是达因 s cm -2或泊。
  • 而在 SI 系统中，粘度系数的单位是 N sm -2或十泊。
  • 粘度系数的量纲公式为[ML -1 T -1 ]。

粘度变化

  • 温度对粘度的影响：液体的粘度随着温度的升高而降低。气体的粘度随着温度的升高而增加，η ∝ √T。
  • 压力对粘度的影响：液体的粘度系数随着压力的增加而增加，尽管到目前为止还没有任何关系可以解释这种现象。

获取某种物质的粘度数据有助于生产商预测材料在现实世界中的表现。了解材料的粘度会影响生产和运输操作的构建方式。因此，下表列出了一些流体及其在不同温度下的粘度系数：

Fluid

Temperature (in °C)

η (deca-poise)

Air

20

0.018 × 10-3

Water

0

1.8 × 10-3

20

1.0 × 10-3

Blood

100

0.3 × 10-3

37

2.7 × 10-3

Engine Oil

30

250 × 10-3

Glycerine

0

10

20

1.5

粘度的应用

各种液体和气体的粘度知识已在日常生活中得到应用。其知识的一些应用讨论如下：

  1. 有机液体的粘度系数用于计算它们的分子量。
  2. 了解粘度系数以及它如何随温度变化使我们能够为每台机器选择最佳润滑剂。低粘度的稀油（例如，钟表中使用的润滑油）用于轻型机械。高粘度油（例如油脂）用于重型机械。粘度是润滑油在润滑中最重要的品质，在润滑脂中也是非常重要的，却经常被忽视。运动阻力定义为粘度。水的粘度很低，因为它流动得很快，但蜂蜜的粘度很高。
  3. 一些药物的粘度，例如用于根除痣的众多溶液，也已降低，以使应用更简单。为了覆盖喉咙，制药公司提供高粘度但仍可饮用的治疗方法，例如止咳糖浆。
  4. 油漆、清漆和其他家居用品的粘度受到严格控制，以便可以用刷辊平滑均匀地涂抹。
  5. 粘度是食物制备和上菜的一个重要因素。食用油的粘度在加热时可能会变化，也可能不会变化，但是当它们冷却时，许多会变得更加粘稠。当脂肪变冷时，它们会变成固体，因为它们在加热时会变得粘稠。
  6. 为了函数运行，制造设备需要使用适当的润滑剂。太粘的润滑剂会堵塞和堵塞管道。过薄的润滑剂对运动部件的保护不足。
  7. 涂层粘度是决定涂层技术成功与否的重要特性之一。由于涂装操作的均匀性和可重复性经常与涂料的粘度有关，因此它是一个重要的调节参数。

示例问题

问题1：平板和大盘之间有3mm厚的甘油层。如果甘油的粘度系数为2 N s/m 2 ，平板的面积为48 cm。以 6 cm/s 的速度移动板需要多大的力？

解决方案：

问题 2：管道的直径是 2 厘米。平流水的最大平均技巧是多少？水的粘度系数为 0.001 Ns/m 2 。

解决方案：

问题3：液体和气体的粘度如何随温度变化？

解决方案：

问题 4：发现液体中某点的剪切应力为 0.03 N/m 2 。该点的速度梯度为 0.21 s -1 。它的粘度是多少？

解决方案：

问题5：水在水平面上缓慢流动，水的粘度系数为0.01泊，表面积为100 cm 2 。保持流体 1 s -1的速度梯度所需的外力是多少？

解决方案：

问题 6：在水平无摩擦地板上，将一块 0.10 m 2表面积的块放置在 0.03 mm 厚的流体层上。施加在块上的水平力为 0.010 kg，它以 0.085 m/s 的固定速度开始移动。求流体的粘度系数。

解决方案：

问题 7：我们可以看到运动粘度有什么不同，如果将具有恒定比重的流体带到一个行星，其重力加速度是地球上的 3 倍？

解决方案：

问题 8：解释伯努利定理。

解决方案：

问题 9：讨论粘度和固体摩擦之间的一些主要异同。

解决方案：