部分分数展开

如果 f(x) 是一个需要积分的函数，则 f(x) 称为被积函数，函数没有任何限制或边界的积分称为不定积分。不定积分有自己的公式，可以使积分过程更容易。但是，有时有些功能过于复杂，无法轻松集成，需要更多时间。例如，在函数的分母中有一个长的二次表达式。在这种情况下，可以在部分分数的帮助下进行不定积分，以使该过程变得简单且耗时更少。

无限整合

不定积分也称为反导数，因为它是微分的逆过程。不定是指函数的整合没有任何限制或界限，需要整合整个函数。函数f(x) 是积分，这里的 x 是积分的变量，积分使用的符号是∫。让我们看一下用于不定积分的一些基本公式，

不定积分的一些基本公式：

Functions	Formulae
∫a dx	ax + C, a∈ R
∫xn dx	$\int\frac{x^{n+1}}{n+1}$ +C, x∉ -1
∫1/x dx	log|x| + C
∫ex dx	ex + C
∫1/√x dx	2√x + C
∫ax dx	$\int\frac{a^x}{loga}+C, a>0$
∫Sinx dx	-Cosx +C
∫Cosx dx	Sinx +C
∫Tanx dx	log|secx| +C
∫Secx tanx dx	Secx + C
∫Cosecx cotx dx	-Cosecx + C
∫ Sec2x dx	tanx +C
∫ Cosec2x dx	– cotx + C

例1：求函数的积分，

f(x) = x ⁵ + 3/x

解决方案：

∫f(x)dx = ∫x⁵ dx + ∫3/x dx

∫f(x)dx = $\frac{x^6}{6}+ 3logx +C$

示例 2：积分，f(x)= 5cosx – 9tanx

解决方案：

∫f(x)dx= ∫[5cosx- 9tanx]dx

∫f(x)dx= ∫5cosxdx- ∫9tanxdx

∫f(x)dx= 5sinx- (log|secx|) +C

除了只需要公式的简单问题外，重要的是要了解复杂的函数不能轻易解决。为了找到复函数的积分，一种方法是使用部分分数，

部分分数积分

有理函数定义为 P(x)/Q(x) 形式的函数，其中 Q(x) ≠ 0。这些函数可以是适当形式，也可以是不适当形式。 Proper ration函数定义为 Q(x) 中的最高程度大于 P(x) 中的最高程度的函数。不当函数是指 P(x) 的最高次数大于 Q(x) 的函数。如果函数中的分母可以分解为线性因子，则有理函数可以很容易地通过偏分数积分。

部分分数分解

被积函数，即给定函数可以分解为更简单的形式，这称为部分分数分解。下面给出了函数的一些广义部分分数，

Functions	Partial Fractions
$\frac{px+q}{(x-a)(x-b)}$, a≠b	$\frac{A}{x-a}+\frac{B}{x-b}$
$\frac{px+q}{(x-a)^2}$	$\frac{A}{x-a}+\frac{B}{(x-a)^2}$
$\frac{px^2+qx+r}{(x-a)(x-b)(x-c)}$	$\frac{A}{x-a}+\frac{B}{x-b}+\frac{C}{x-c}$
$\frac{px^2+qx+r}{(x-a)^2(x-b)}$	$\frac{A}{x-a}+\frac{B}{(x-a)^2}+\frac{C}{(x-b)}$
$\frac{px^2+qx+r}{(x-a)(x^2+bx+c)}$, x2+ bx+ c cannot be factorized	$\frac{A}{x-a}+\frac{Bx+C}{x^2+bx+c}$

这些广义形式是如何获得的，或者是否有任何步骤可用于部分分数分解？是的。这些步骤可以很容易地帮助达到完美的部分分数形式，

部分分数分解的步骤

• 为了分解，从正确的有理表达式开始。将分母分解为最基本的形式。
• 写下得到的单独的部分分数，为了处理分子，用很快就会发现的变量填充它们。
• 现在，为了找到变量的值，这里是 A、B 和 C，将方程乘以分母。
• 通过相应地替换不同的值来求解变量。
• 得到分子的值后，将它们放在部分分数中。

使用长除法或合成除法积分

当函数没有以有理形式给出时使用这种方法，即分数是假分数并且分子的次数大于或等于分母的次数。在这些情况下，假设分子的度数是 a，分母的度数是 b，在这里做部分分数时，需要记住，（ab）的附加值与分母一起被添加到分母中。部分分数。

让我们考虑下面给出的函数：

f(x) = $\frac{x^5 - 6x^4 + 5x^2 + 8 }{x + 2}$

该函数是一个不正确的积分，因此可以使用长除法求解。

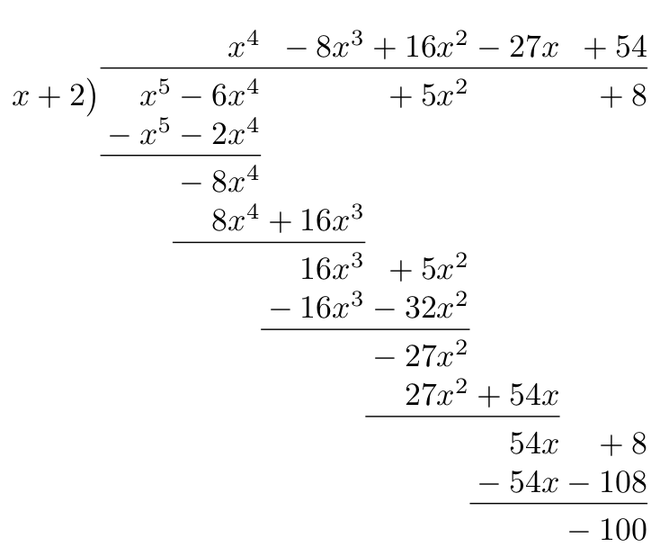
这里，S(x) = x ⁵ – 6x ⁴ + 5x ² + 8 和 R(x) = -100 和 Q(x) = x + 2。

因此，积分可以重写为，

∫(x ⁵ – 6x ⁴ + 5x ² + 8 + $\frac{-100}{x + 2}$ )dx

⇒ $\int (x^5 - 6x^4 + 5x^2 + 8 + \frac{-100}{x + 2} )dx \\ = \frac{x^6}{6} -\frac{6x^5}{5} + \frac{5x^3}{3} + 8x -100ln(x + 2)$

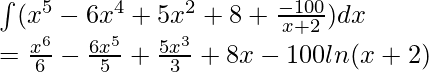
If the numerator P(x) has a degree greater than or equal to the degree of the denominator Q(x), then the rational function $\frac{P(x)}{Q(x)}$is called improper. In this case, we use long division of polynomials to write the ratio as a polynomial with a remainder. 

Let’s say dividing P(x) by Q(x) gives S(x) with the remainder R(x), then the degree of R(x) is less than the degree of Q(x) as a result of the long division.  

$\frac{P(x)}{Q(x)} = S(x) + \frac{R(x)}{Q(x)}$

After doing this, the function can be integrated.

示例问题

问题一：积分，f(x)= sin(x) + 3tanx

解决方案 ：

∫f(x)dx= ∫[sin(x) + 3tanx]dx

∫f(x)dx= ∫sin(x)dx + ∫3tanxdx

∫f(x)dx= -cos(x) + 3(log|secx|) +C

问题2：求下列函数的积分。

f(x) = $\frac{x + 1}{x(x + 2)}$

解决方案：

The given function can be decomposed into partial fractions. 

f(x) = $\frac{x + 1}{x(x + 2)}$

$\frac{x + 1}{x(x + 2)} = \frac{A}{x} + \frac{B}{x + 2} \\ = \frac{x + 1}{x(x + 2)} = \frac{A(x + 2) + B(x)}{x(x + 2)} \\ = \frac{x + 1}{x(x + 2)} = \frac{x(A + B) + 2A)}{x(x + 2)}$

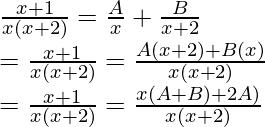
Comparing both sides of the equation, 

A + B = 1

2A = 1

From both these equations, it can be concluded that. 

A = 1/2, B = 1/2 

Thus, the function becomes, 

f(x) = $\frac{1}{2x} + \frac{1}{2(x + 2)}$

Now, 

F(x) = ∫f(x) 

⇒ F(x) = $\int (\frac{1}{2x} + \frac{1}{2(x+2)})dx$

⇒ F(x) = $\frac{1}{2}ln(x) + \frac{1}{2}ln(x+2)$

问题 3：求下列函数的积分。

f(x) = $\frac{x }{(x + 1)(x + 2)}$

解决方案：

The given function can be decomposed into partial fractions. 

f(x) = $\frac{x + 1}{x(x + 2)}$

$\frac{x}{(x + 1)(x + 2)} = \frac{A}{x+1} + \frac{B}{x + 2} \\ = \frac{x}{x(x + 2)} = \frac{A(x + 2) + B(x + 1)}{x(x + 2)} \\ = \frac{x}{x(x + 2)} = \frac{x(A + B) + 2A + B}{x(x + 2)}$

Comparing both sides of the equation, 

A + B = 1

2A + B = 0

From both these equations, it can be concluded that. 

A = -1, B = 2 

Thus, the function becomes, 

f(x) = $\frac{-1}{x + 1} + \frac{2}{(x + 2)}$

Now, 

F(x) = ∫f(x) 

⇒ F(x) = $\int (\frac{-1}{x + 1} + \frac{2}{x+2})dx$

⇒ F(x) = $-ln(x + 1) + 2ln(x+2)$

问题 4：求下列函数的积分。

f(x) = $\frac{x - 1}{x(x^2 - 1)}$

解决方案：

The given function can be decomposed into partial fractions. First let’s simplify the function. 

f(x) = $\frac{x - 1}{x(x^2 - 1)}$

$\frac{x - 1}{x(x^2 - 1)} = \frac{x - 1}{x(x + 1)(x - 1)} \\ = \frac{x - 1}{x(x^2 - 1)} = \frac{1}{x(x+1)} \\$

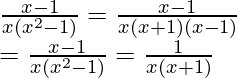
Decomposing the function, 

$f(x) = \frac{1}{x(x+1)} \\ = \frac{1}{x(x+1)} = \frac{A}{x} + \frac{B}{x + 1} \\ = \frac{1}{x(x+1)} = \frac{A(x + 1) + Bx}{x(x + 1)} \\ = \frac{1}{x(x+1)} = \frac{x(A + B) + A}{x(x + 1)} \\$

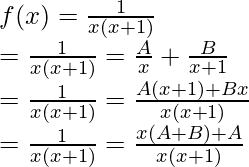
Comparing both sides of the equation, 

A + B = 0

A = 1

From both these equations, it can be concluded that. 

A = 1, B = -1

Thus, the function becomes, 

f(x) = $\frac{1}{x} + \frac{-1}{x + 1}$

Now, 

F(x) = ∫f(x) 

⇒ F(x) = $\int (\frac{1}{x} + \frac{-1}{x+1})dx$

⇒ F(x) = ln(x) – ln(x+1) 

问题 5：求下列函数的积分。

f(x) = $\frac{x^3 - 8}{x(x - 2)}$

解决方案：

The given function can be decomposed into partial fractions. First let’s simplify the function. 

f(x) = $\frac{x^3 - 8}{x(x - 2)}$

$\frac{x^3 - 8}{(x)(x - 2)} = \frac{(x-2)(x^2 + 4 + 2x)}{(x)(x - 2)} \\ = \frac{x^2 + 4 + 2x}{x} \\ = x + \frac{4}{x} + 2$

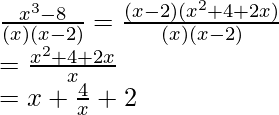
Integrating the function, 

F(x) = ∫f(x)dx

⇒ F(x) = ∫($x + \frac{4}{x} + 2$)dx

⇒ F(x) = $\frac{x^2}{2} + 4ln(x) + 2x$